Symmetry breaking for 4 scalar fields

Question

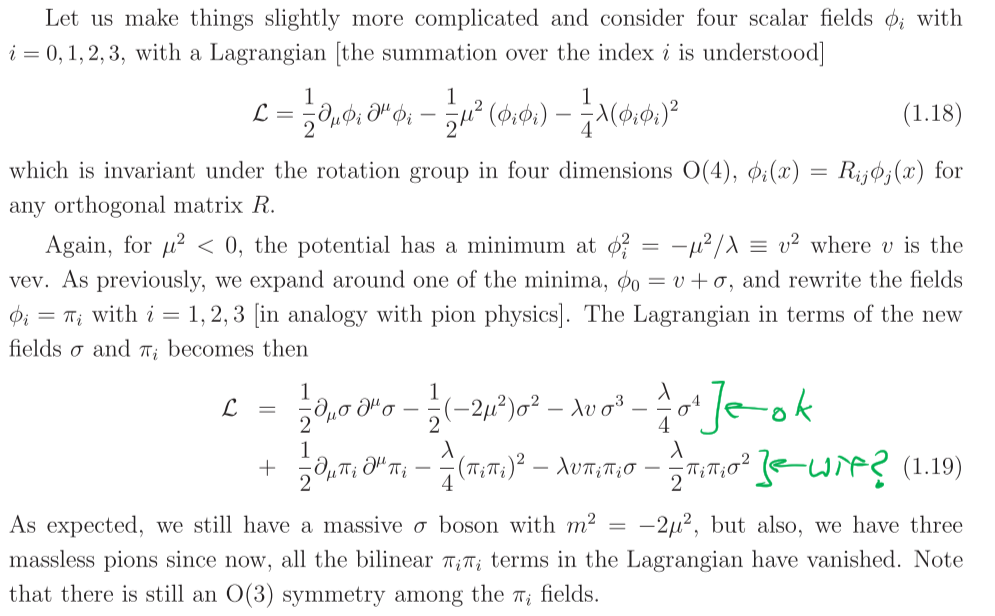
So I've been trying to get familiar with concept of symmetry breaking with minimal experience with field theory and I've got stuck on, I think, a simple problem. Let's consider four scalar fields $\phi_i$ and their Lagrangian: $$\mathcal{L}=\frac{1}{2}\partial_\mu\phi_i\partial^\mu\phi_i-\frac{1}{2}\mu^2(\phi_i\phi_i)-\frac{1}{4}\lambda(\phi_i\phi_i)^2.$$ The potential is quartic and, if $\mu^2<0$, the minima are at $\phi_i=-\mu^2/\lambda\equiv v^2$. To correctly(why?) interpret theory we have to expand around one of the minima by introducing new scalar field $\phi_0=v+\sigma$. Additionally the reference decided to rewrite, for $i\neq0$, $\phi_i=\pi_i$. Therefore they obtained new Lagrangian: $$\mathcal{L}=\frac{1}{2}\partial_\mu\sigma\partial^\mu\sigma-\frac{1}{2}(-2\mu^2)\sigma^2-\lambda v\sigma^3-\frac{\lambda}{4}\sigma^4$$ $$+\frac{1}{2}\partial_\mu\pi_i\partial^\mu\pi_i-\frac{\lambda}{4}(\pi_i\pi_i)^2-\lambda v\pi_i\pi_i\sigma-\frac{\lambda}{2}\pi_i\pi_i\sigma^2.$$ The first line is clear to me, but I struggle with the second one - I have no idea where do the terms mixing $\pi_i$ and $\sigma$ come from and why there is no $\pi_i\pi_i$ term, which is important for further discussion about fields' masses. I would be very grateful if somebody could lead me through the calculations. I've attached a picture with the whole example from the publication I'm reading trough: https://arxiv.org/abs/hep-ph/0503172 I hope it's clearer than what I've wrtitten.

Answer 1

The easiest way to work out your algebra, in case you haven't, and are alarmed by the missing constant term, is to rewrite your potential, eliminating the pestiferous unphysical $\mu^2$ that has caused grief to generations of students. Recalling that summations over repeated indices is implied, the potential is $$ \tfrac{\lambda}{4} (\phi_i \phi_i - v^2)^2 -\frac{\lambda}{4} v^2 \\ = \tfrac{\lambda}{4} (\pi_a \pi_a +(\sigma +v)^2- v^2)^2 -\frac{\lambda}{4} v^2 \\ =\tfrac{\lambda}{4} (\pi_a \pi_a +\sigma^2 +2v\sigma)^2 -\frac{\lambda}{4} v^2. $$ I have used indices a=1,2,3 for the 𝜋 s, to avoid confusion with the four i s.

Expanding the square, you retrieve your (1.19), six terms, now with manifest O(3) symmetry; with a quartic in the 𝜋 s, but no bilinear mass term, as there is no constant term inside the square anymore. These fields are massless (goldstons, upon quantization)! There is a bilinear for the 𝜎 field, from the linear term inside the square, so that field is massive.

You should be able to work out the kinetic terms, as $\partial_\mu v=0$.

This is a mere change of variables, but now you can make sense of your field theory expanding around the vacuum, which you couldn't do before, as a comment/link reminds you.