PN junction: not sure to understand why reverse-biais lead to negligible current

Question

My question is very highly related to Why doesn't current flow in reverse biased diode?

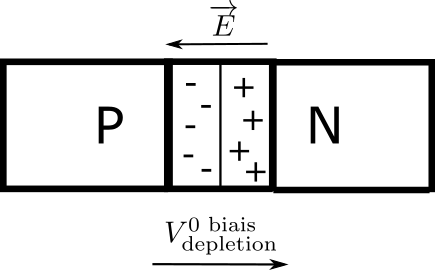
Basically, I would like to understand in more depth John Rennie answer. At $t=0$, I consider a PN junction not plugged to any generator. Then, I will have a depletion region and something like:

The diffusion of the holes from P to N (and of the electron from N to P) is being compensated by electrostatic force created on the depletion region. Nothing is moving.

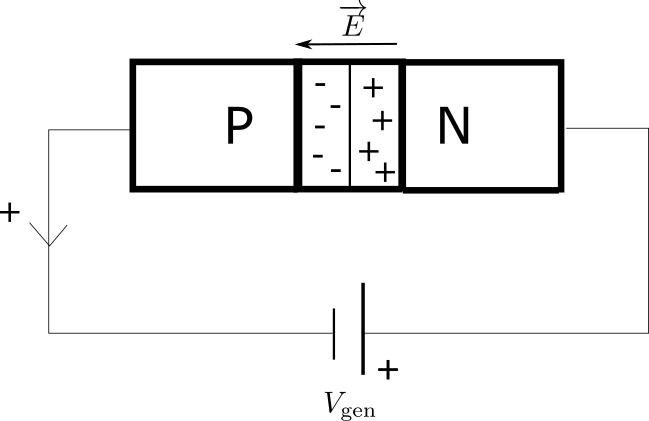
Then, at $t=0$, I plug in a voltage generator in reverse biais.

I focus on holes (but analog reasoning holds for electrons). They cannot move from left to right in the depletion region because of the electrostatic field. I could imagine them moving from right to left. They are negligible in the N region so we could believe that such motion is impossible. But they can go from P to the wire, arrive to N and then cross the depletion region.

On the other post it is explained that this gives rise to a transient current that will stop after some time. This is precisely what I would like to understand.

My question

At $t=0^+$, a current can be initiated from the phenomenon I described. Now, in principle $V_{gen}$ can be different from the potential of the depletion region at zero biais.

Thus I guess that what happens is that for a short time: $$V_{\text{gen}} \neq V_{\text{depletion}}$$ (we are not in electrostatic so it is not contradictory with electric laws: $\overrightarrow{E}=-\overrightarrow{\nabla} V - \partial_t \overrightarrow{A}$, electric field is not conservative outside of electrostatic regime)

Then, after this transient regime, things go to equilibrium and I have:

$$V_{\text{gen}}=V_{\text{depletion}}$$

Where of course, now $V_{\text{depletion}} \neq V_{\text{depletion}}^{\text{0 biais}}$.

Do you agree until now ?

We could believe that the physics is now equivalent to a standard PN junction in which the depletion zone would have instead of a voltage drop $V_{\text{depletion}}^{\text{0 biais}}$, a voltage drop $V_{\text{depletion}}$.

But still, there are those "wires" around that allow the holes from P to move toward N. The fact $V_{\text{gen}}=V_{\text{depletion}}$ is for me irrelevant to the discussion, if I replaced the PN junction by a resistor I would have $V_{\text{gen}}=V_{\text{resistor}}$ and there would obviously have a current in the resistor in this case.

What am I missing here ?

Answer 1

I think you understand almost everything, but it seems the crucial thing you’re missing is this:

There will be a short-lived initial current but it will quickly (mostly) stop as soon as the potential at the top end of each wire (mostly) equilibrates with the potential at the bottom end of each wire. This never happens in the case of a resistor.

Let me explain how this happens, and why the resistor case is different. First, to make sure my makeshift terminology is clear, let's look at the picture:

When I say the top end of the left wire, I am referring to the place where the left wire connects to the p-type semiconductor. The bottom end is the place where it connects to the battery.

Now, to avoid a 0/0 indeterminacy, and to be more realistic, we should remember that each wire has some low, but non-zero resistance. This will actually make the analysis more clear. Also, let's only look at the left wire. The analysis for the right wire will be the same.

When you connect a battery to the diode, or to a resistor, what happens? We can think of the battery as some black box mechanism to enforce a constant potential difference $\Delta V$ between its negative and positive terminals, no matter what happens. This will set up a gradient of potential throughout the whole circuit, which will push the current to start flowing counterclockwise.

Let's focus again specifically on the left wire. As part of that overall gradient of potential, the battery created a potential difference between the two ends of the left wire, and the current is flowing from its top to its bottom end.

In the case of a resistor, as we know this will be the case forever, the current will never stop.

By contrast, in the case of the diode, as holes flow through the left wire, the left side of the junction is getting robbed of more and more holes, and will therefore develop a negative charge, which will gradually make it harder and harder for the holes to keep flowing. The same of course will happen in the wire on the right hand side, for electrons.

From the perspective of the potential, the potential at the top end of the left wire will get lower and lower until it (mostly) matches the potential at its bottom end, at which time current will (mostly) stop.

What's the crucial difference between the diode and the resistor? The holes have a very hard time crossing from the right side of the diode to the left side, to replenish the deficit! Why? If we look at the picture again, it would seem that the electric field in the depletion zone should help them cross from right to left, because it’s pointing to the left. So why wouldn't they want to cross?!

The answer is, if any hole makes it to the right side of the depletion zone, then yes, the electric field will propel it further to the left and it will happily cross. The problem is that the overwhelming majority of holes supplied by the right wire "die" way before they reach the depletion zone. And when I say die, I mean they will encounter an electron and annihilate.

The reason I say "(mostly)" several times in the above paragraphs is because there will be some very small number of holes that manage to make it from the right side of the diode to the left side, so some very small amount of current will exist. Even though the right side of the diode is teeming with electrons (majority carriers), thermal fluctuations occasionally produce an electron-hole pair (an electron gets excited from the valence band to the conduction band). If a hole produced this way makes it to the depletion zone without being annihilated (or is born in the depletion zone itself), then it will happily be swept away by the electric field and will make it to the left side of the diode to join its comrades. The tiny current produced this way is called the thermal current.

Note: just to make sure we understand the final configuration, and ignoring the tiny thermal current, in the end the potential everywhere in the left wire will be the same, but it will be lower than the potential everywhere in the right wire, by $\Delta V$, the battery’s voltage.

Answer 2

The electric field at the junction results from electrons migrating from the conduction band of the N-type to the valence band of the P-type, because they fill lower energy states in the process.

The region of the conduction band near the junction loses its precious charge carries (electrons). And the valence band of the P-side close to the junction loses its precious holes.

When electrons flow from the N type to the battery, in reverse polarization, the continuation of the process requires that those electrons go through the wire to the P-type. There, they recombined with holes. So there is a flow of holes from the junction to the left.

The electric field in the junction increases as a consequence until match the battery voltage. But you can say that the same happens with a battery and a resistor, and there is a flow of current.

The analogy can be made, but here the central region is so depleted of charge carries, that the resistor has very high resistance, working as an isolator for all practical considerations. The analogy with a capacitor, with a dielectric without charge carries is maybe more close to reality.

When the polarity is direct, charge carries are forced to the junction, and the natural process of electrons losing energy from N to P continues because the resulting electric field is overcome by the electric field of the battery.

Answer 3

Congratulations, you have got most of what you need to understand junction capacitance. Yes, you add a reverse bias voltage, and charge will move from the p-region (and n-region) through the supply. What you missed are two key pieces:

1. a hole (electron) will not last long in n-type (p-type) material - it is playing tag with the opposite carrier type but is outnumbered a billion to one. The net result is carrier recombination and the minority carrier disappears. (Note - to really understand semiconductor physics you must think about both carriers at the same time).

2. As the hole (electron) leaves the p-type (n-type) region, additional ionic charge is left behind in the depletion region. This growth in the depletion region continues until the depletion region counteracts the applied voltage. You are now back to a static situation, just with a broader depletion region.

Answer 4

Your drawing is missing 2 junctions. One between the p-type and the wire, and another between the n-type and the wire.

The voltage drop on these 2 junctions will compensate V_depletion and the wire will be no need potential across it.

See Feynman section 14-4 for a similar discussion