0
$\begingroup$

I know that the question "why can't a reverse biased diode conduct" has been asked before (here and here), but I'm asking it from a bit different perspective.

Why can't we inject electrons into the conduction band of the p-type material, which then would fall down the junction into the conduction band of the n-type material, and vice versa, inject holes into the valence band of the n-type material, which would climb up the junction to reach the valence band of the p-type material? This would even be energetically favourable, since this way both carriers would move through the reverse biased built-in potential which is their natural movement direction, not like in the case of forward bias, where they have to move by diffusion.

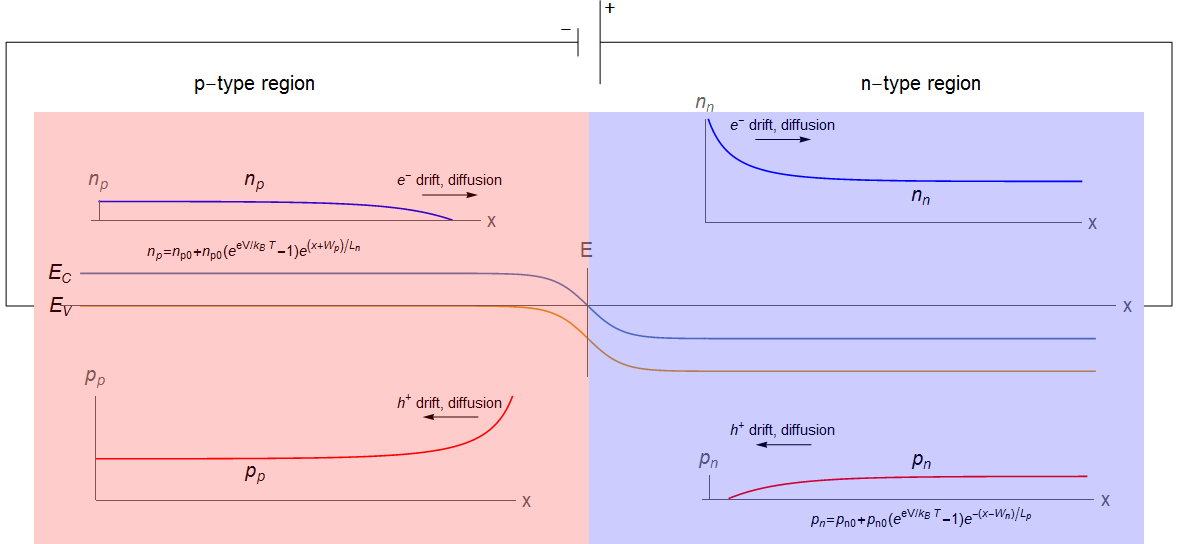
I have been thinking a lot about this, but still couldn't understand, so I'm updating the question. I meant injecting charge from the electrodes (suppose that they are ideal, non-rectifying), not injecting (e.g. photo-) generated charge through the depletion region. I have drawn a figure, which shows the charge carrier concentrations and energy levels in the diode in case of a reverse bias. To be honest, in case of the majority carrier concentrations in a region, I just simply took the $n_i^2/\text{minority carrier concentration}$, which is not exactly true near to the junction, due to the Fermi levels being separated (so this might be the trick). The "$0$" index denotes equilibrium concentrations, when there is no junction formed, there is just the p- and the n-type material, separate from each other. The way I see it, both the concentrations, and the electric field is such, that there could be current flowing. So my question remains, why can't be electrons injected from the (ideal!) contacts to the conduction band of the p-type material, and holes into the valence band of the n-type material, which would permit a (quite huge) reverse current. enter image description here

Improve this question
$\endgroup$
5
  • 1
    $\begingroup$ You mean like how a solar cell works (or a photodiode)? Or a surface barrier detector for charged particles? Yes, if you inject charge carriers through some (external) process into a depletion layer, they will move. $\endgroup$
    – Jon Custer
    Commented Apr 6, 2016 at 17:33
  • $\begingroup$ @JonCuster I didn't mean into the depletition layer, but into either of the semiconductors, from the battery. See my comment below L.Levrel's answer. $\endgroup$
    – user3237992
    Commented Apr 7, 2016 at 9:13
  • $\begingroup$ OK, you might want to look in to the Gunn and/or Hecht equations. For example, in the solar cell case, the minority carrier in the electron-hole pair generated near to, but not in, the depletion layer can indeed diffuse to the depletion layer edge and then get swept in to the junction to generate current. $\endgroup$
    – Jon Custer
    Commented Apr 7, 2016 at 12:46
  • $\begingroup$ @JonCuster But can you tell me why it is not possible what I said in the comment? (Honestly, I'd like to avoid further equations, unless absolutely necessary.) $\endgroup$
    – user3237992
    Commented Apr 7, 2016 at 16:16
  • $\begingroup$ Tip: Concerning editing see this meta post. $\endgroup$
    – Qmechanic ♦
    Commented May 16, 2016 at 13:27

1 Answer 1

Reset to default
3
$\begingroup$

If you inject electrons in the p-type material, they will "immediately" recombine with holes there (which are in excess). If you inject holes in the n-type, conduction electrons (which are in excess) will "immediately" fill them.

As stated by Jon Custer, if you create electron-hole pairs in the depletion region, the field will separate them, thus creating a current. E.g. in a photodiode, where the internal photoelectric effect excites a valence electron into the conduction band, thus creating an electron-hole pair.

Answer to the update: your diagram looks wrong. Have a look at the Wikipedia page about P-N junctions. Whatever the bias direction, the concentration of majority carriers decreases approaching the junction. And this is not due to increasing numbers of minority carriers, but to recombination. A crucial feature missing in the diagram is the space-charge region.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Well, I guess this "immediate recombination" is the key to it, it's just hard for me to believe. I wasn't talking about generation of pairs in the depletition region, but simply a diode with a reverse bias (let's say at room temperature and in dark). However, after all the majority carriers recombined, why doesn't current (larger than the usual reverse current) flow by the process I described by the question? (E.g. after all the holes are gone in the p-region, the conduction band could be filled up by electrons which can conduct current.) $\endgroup$
    – user3237992
    Commented Apr 7, 2016 at 9:12
  • $\begingroup$ The more you reverse-bias the diode, the larger the depletion layer, the stronger the electric field in it. Practically it looks like breakdown (Zener and/or avalanche) will take place before the depletion layer fills the whole diode, because I see no other breakdown situation described (what you expect would be a kind of breakdown). $\endgroup$
    – L. Levrel
    Commented Apr 7, 2016 at 19:15
  • $\begingroup$ I still can't really understand, although I have been thinking quite a lot about this. I have clarified the question a bit more, and have drawn a figure. Could you maybe update your answer? $\endgroup$
    – user3237992
    Commented May 16, 2016 at 13:23
  • $\begingroup$ Well, the space charge region is implicitly there, but never mind, I think I finally got it. The only thing I ask finally is, whether you can tell me what equations describe the majority carrier distributions. I would just like to see (plot) the distribution in the reverse case. $\endgroup$
    – user3237992
    Commented May 16, 2016 at 21:23
  • 1
    $\begingroup$ I don't know of any explicit formulas. The animated GIF on the WP page was made from this tool. From the demo (scroll down to find it), you can see the graphs are simulation results. I didn't explore that site, but it looks like it has lots of material about semiconductors. $\endgroup$
    – L. Levrel
    Commented May 17, 2016 at 17:35

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.