In a question What is incorrect about the original statement of Fermat's principle? is showed an example of reflection in which Fermat principle involve a maximum, and in comments is said that it could be interesting an example involving refraction but question is left pending. I can think examples in which Fermat principle with refraction involve minima (Snell's law) or stationary optical path length (lenses converging light from a point source to another point), but I can't find a refraction example that involve a maximum.
$\begingroup$
$\endgroup$
1
-
$\begingroup$ In non-imaging optics light trapping structures (using refraction) are used to maximise the entropy of incoming light rays so they ergodically occupy the phase space (essentially by randomising their directions). This increases the path length of rays by $4n^2$. This is more of a statistical mechanics observation of the whole beam, rather that single particle principle like least action. $\endgroup$– boyfarrellCommented Dec 25, 2020 at 12:25
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
First of all, note that a stationary optical path of finite length can never be a local maximum among virtual paths (even if we are allowed to use reflections), because it is always possible to locally device a longer virtual detour. (The previous sentence does not imply that the set of stationary optical paths of finite length couldn't have a maximal element.)
From now on let us assume that the refractive index $n({\bf r})\geq 1$ is a smooth function of position ${\bf r}$, and that there are no mirrors.
It is still possible to artificially mimic reflection (as long as the angle of incidence is not zero) by letting the $n({\bf r})$ become smaller in the "reflection layer" (and bigger in the bulk). Think e.g. of an optical fiber with a smooth gradual fusing of the cladding, cf. Fig. 1.
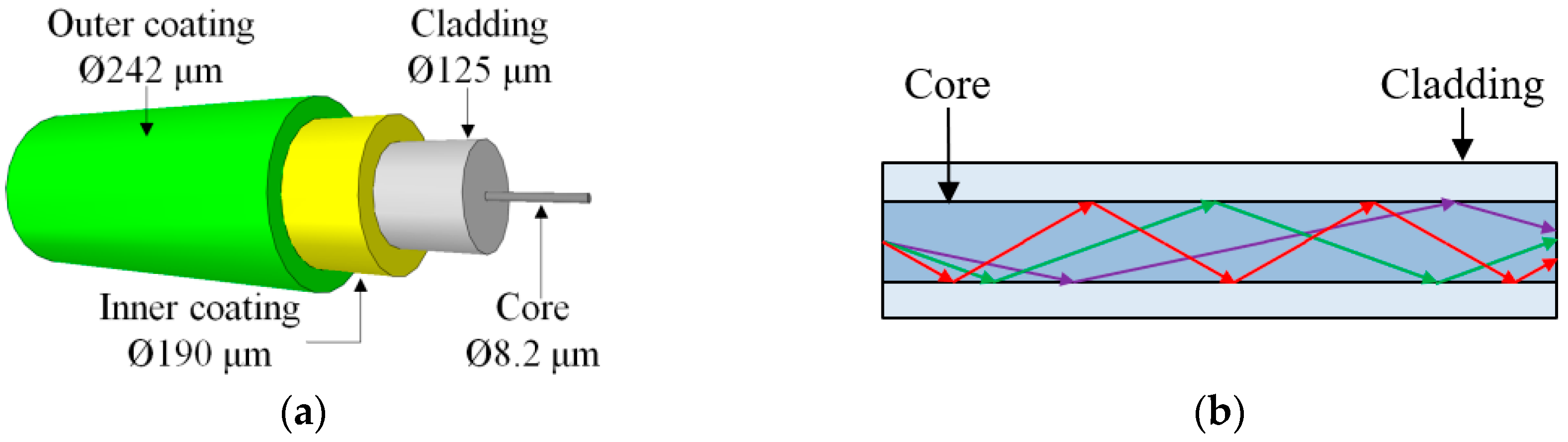
$\uparrow$ Fig. 1a & 1b. A typical optical fiber. The reader should imagine that refractive index $n({\bf r})$ varies smoothly between the core and the cladding, thereby effectively creating a tubular mirror.
Similarly, we can approximate/mimic many optical systems that contains mirrors, or say, boundaries between 2 media. E.g. the systems discussed in OP's linked Phys.SE post and links therein.
answered Dec 24, 2020 at 18:09
-
$\begingroup$ It Is not important if se are dealing with transparent media or not, transparent media too have reflection (partial or total) but if we exploit reflection the essence of the question is lost. I could write: we know that exploiting reflection we can build rays that have optical path length of all 3 possible type. But if I try to build examples in which refraction play a role I can find only 2 examples: I can't find an apparatus in which a refracted ray is selected by Fermat principle because optical path length is maximum. I wonder if it is possibile, this is a puzzle. In point 1 you say not? $\endgroup$ Commented Dec 25, 2020 at 13:03
-
$\begingroup$ The example is a parabolic prism, the light entering by the "rear" plane and should following snell law go straight to the exit which is the tip of the parabola. But if speed outside the prism is bigger then this is not the quickest path, if one knows the endpoint. So it is a check between local and nonlocal approach of the problem. $\endgroup$ Commented Mar 16 at 11:29
-
$\begingroup$ Here it is a local maximum. But if the fastest path is taken, then probably shapewaves and feedback should be used, which is entirely counter intuitive $\endgroup$ Commented Jul 7 at 20:10
-
$\begingroup$ Another way were to use the smoothness of the description of the system : at the entry point light get enough information on the derivatives forexample that it knows the shape of the whole setup. $\endgroup$ Commented Jul 25 at 6:14