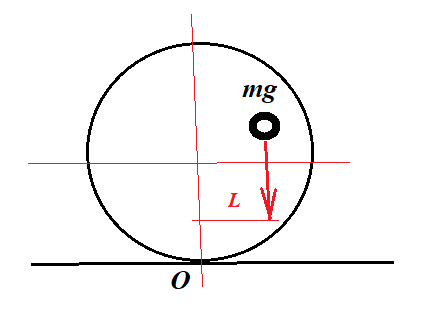
If you're just curious about why the instantaneous system of forces has a horizontal component which leads to horizontal motion (w.r.t. the earth), then you can consider that the system is instantaneously similar to an inverted pendulum. If the friction doesn't allow any slip between floor and rocking chair, then the centre of mass is instantaneously constrained to move on a tangential motion around the pivot on the floor, as in the following diagram:
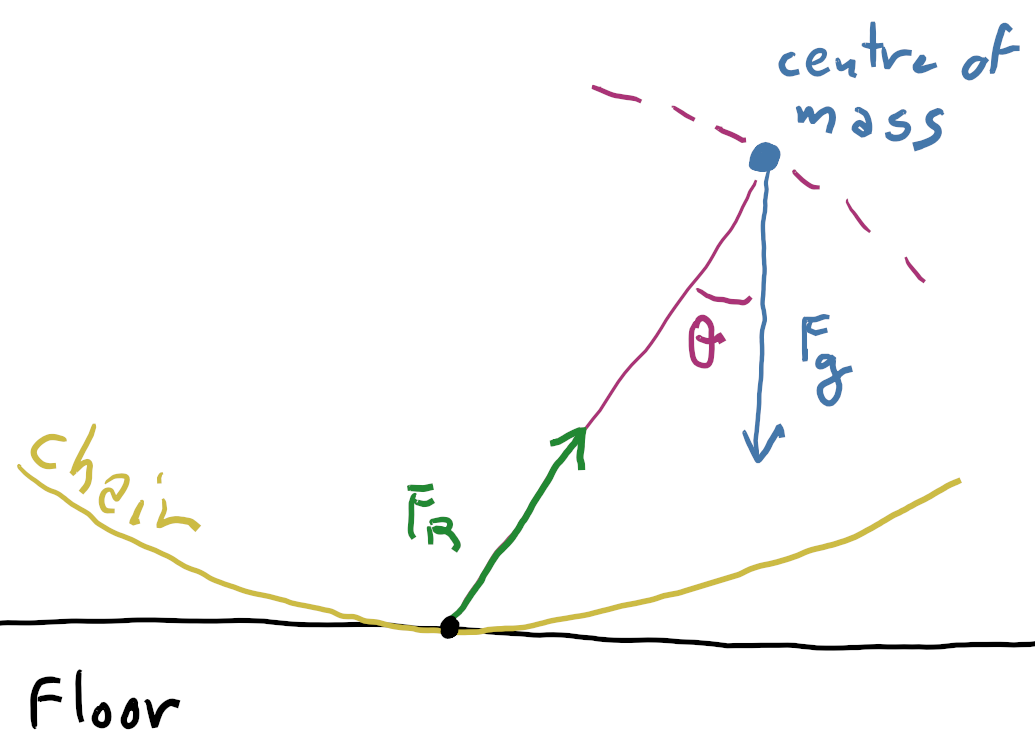
The pivot is the black dot; the centre of mass is the blue dot; its constrained instantaneous direction of motion is the dashed red curve.
This situation implies that the reaction force of the floor is directed as the green vector $\pmb{F}_{\text{R}}$ in the picture, and its magnitude must balance the component of the gravitational force $\pmb{F}_{g} \equiv m\pmb{g}$ along the line connecting centre of mass and pivot. With some trigonometry we find that this magnitude is $\lvert \pmb{F}_{\text{R}} \rvert = \lvert \pmb{F}_g\rvert\ \cos\theta$, in terms of the angle $\theta$ depicted in the picture.
So the total force on the centre of mass is $\pmb{F}_{\text{T}} = \pmb{F}_{g} + \pmb{F}_{\text{R}}$, and again with some trigonometry we see that by construction it must be tangential to the dashed red curve. It therefore has a vertical component and a horizontal component:
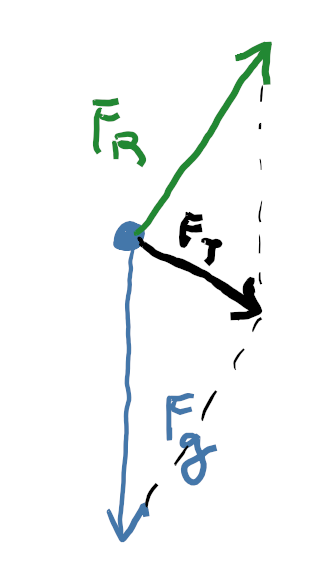
If there is no friction between floor and chair (say because of a layer of oil), then the reaction force would be purely vertical upwards, and its magnitude would be less than $F_g$, because it would only compensate for the weight of the part of the system directly above it. Then the total force would also be purely vertical and directed downwards, and the centre of mass would not have any horizontal motion.
This latter case is more or less what happens when people slip and fall on the floor: typically their feet go horizontally one direction, say backwards, and the face goes the opposite direction, say forwards. And the centre of mass falls more or less vertically.
It may be worth noting that in this kind of problems our deductions using Newton's laws sometimes go in the direction "motion $\rightarrow$ forces", rather than "forces $\rightarrow$ motion": we experimentally see that there is horizontal motion and no slip on the floor, and therefore conclude that there must be horizontal friction. This was exactly your kind of deduction :)