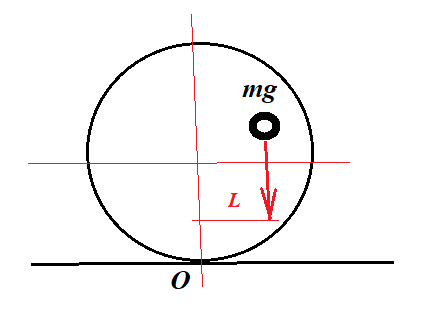
I've replaced the rocking chair with a circle with a smaller inset circle representing the centre of gravity (CoG). The weight is $mg$.
Now assume there's plenty of friction between the circle (rocking chair) and floor, so as to prohibit any sliding/slipping.
At this point the distance between the vector $\mathbf{mg}$ and the vertical running through $\text{O}$ is $L$.
Gravity now forces the CoG to be lowered. A torque $\tau$ about the point $\text{O}$ arises:
$$\tau=mgL$$
This torque causes rotational acceleration of the circle:
$$\tau=I\alpha$$
This angular acceleration causes not only to move the CoG downward but also to the right.
Note that due to inertia the system, unimpeded, will enter into an oscillatory mode (hence 'rocking chair')