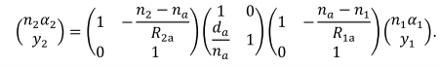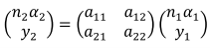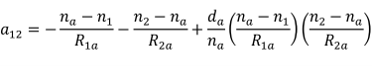1
$\begingroup$

The well-known equation for thin lens is: $$\frac{1}{f}=\left(\frac{n_L}{n_m}-1\right)\left(\frac{1}{R_1}-\frac{1}{R_2}\right)$$ But there's a more appropriate equation that includes the thickness of the lens, which is: $$\frac{1}{f}=\left(\frac{n_L}{n_m}-1\right)\left(\frac{1}{R_1}-\frac{1}{R_2}+\frac{(\frac{n_L}{n_m}-1)d}{n_LR_1R_2}\right)$$ However, I can not find any derivation of it online. As far as I managed to find is the derivation of the following: $$\frac{1}{f}=\left(\frac{n_L}{n_m}-1\right)\left(\frac{1}{R_1}-\frac{1}{R_2}+\frac{n_Ld}{s_{i1}(s_{i1}-d)}\right)$$ which can be found at page 167 (Equation 5.14) of Optics by Eugene Hecht: https://edisciplinas.usp.br/pluginfile.php/5054148/mod_resource/content/1/Hecht-optics-5ed.pdf

Improve this question
$\endgroup$
2
  • $\begingroup$ Focal length of the lens is the place where a parallel bunch of rays converge. Spherical lens does not, in fact, collect those rays into one exact location. Thus, it is said that the first well-known formula is valid only for f>>R and of course f>>R>>d even though d does not even enter the first formula. Now, you are asking for a more complicated formula where f is modified by d. The first question I would ask, if this thickness d is not too small, are you going to measure the distance to the focal point from the front or the back of the lens? $\endgroup$
    – Kphysics
    Commented Nov 29, 2020 at 9:16
  • $\begingroup$ ...contd...And the second question is, if are you going to allow d ~ R in other words a a really thick lens, how well is it going focus? Is this correction to the first formula worth it? $\endgroup$
    – Kphysics
    Commented Nov 29, 2020 at 9:16

3 Answers 3

Reset to default
1
$\begingroup$

A spherical thick lens, of index of refraction na, immersed between two different optical media, with index of refraction n1 and n2, consist in two refractive spherical surfaces of radios R1a and R2a, separated a distance da over the optical axis, see Figure bellow:
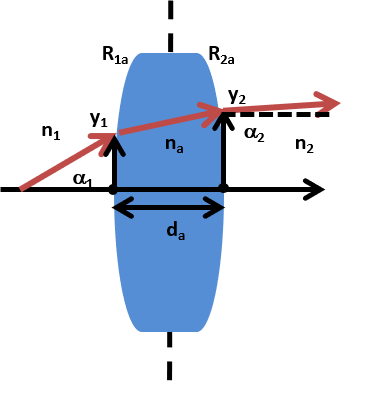
Thick Lens

In the figure, a generic optical ray (in brown color) is propagating in the first media with index of refraction n1 with a alpha1 angle with respect to the optical axis. Then the ray is refracted by the first surface of the lens, at a height y1. After that the ray propagates inside the lens with refraction index na (a distance da measured over the optical axis) and is refracted by the second surface, leaving the lens with an angle of alpha2 at a height y2 with respect to the optical axis, following further propagation into the n2 medium. The mathematical relation between angles and heights in the two surfaces can be obtained using the optical matrix formalism in the following way:

enter image description here

Performing the matrix product:

enter image description here

The expression corresponding to the matrix element a12 is:

enter image description here

Which is the focal distance for the thick lens. Making n1=n2=1, it is obtained the expression for the focal distance (or effective focal distance, as is called by Hetch in "Optics"), for a thick lens inmersed in air. See http://pubs.sciepub.com/ijp/9/6/1/ for more details.

Improve this answer
$\endgroup$
1
  • $\begingroup$ While this link may answer the question, it is better to include the essential parts of the answer here and provide the link for reference. Link-only answers can become invalid if the linked page changes. - From Review $\endgroup$
    – Miyase
    Commented Feb 28, 2023 at 20:47
1
$\begingroup$

Here is the answer I came up with after thinking about my question again.

Consider the derivation of the common lens equation (See below the picture of the linked derivation). Note that one of the steps is to consider the height at which the beam of light enters and exits the lens to be the same. To account for the thickness of the lens, one needs to consider the difference in these heights.

2

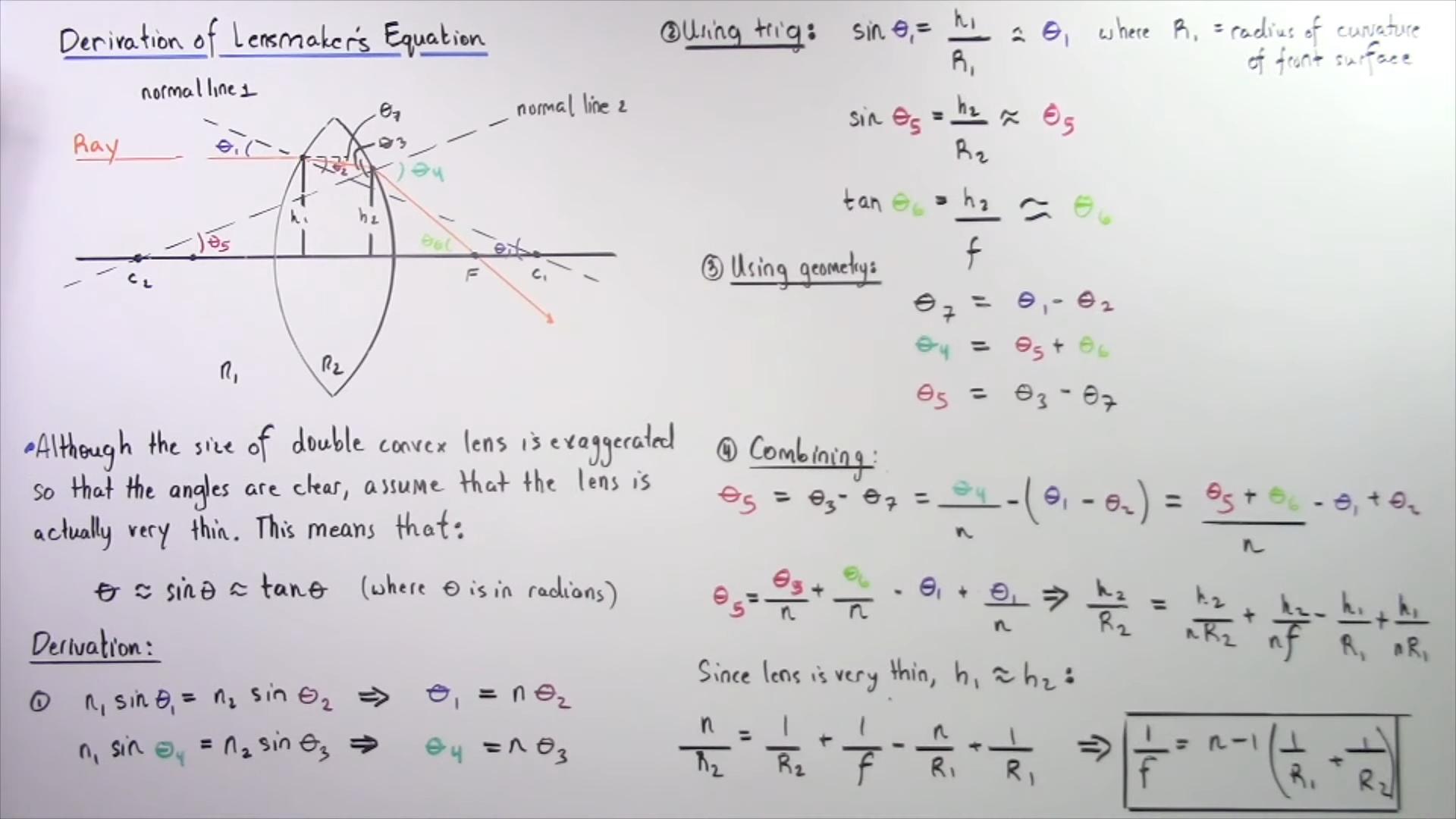
Note that $$\tan(\theta_7)\approx\frac{h_1-h_2}{d}$$ Then, using trigonometric approximations and the equations in the derivation, $$\theta_7\approx\frac{h_1-h_2}{d}$$ $$\theta_1-\theta_2\approx\frac{h_1-h_2}{d}$$ $$\frac{h_1}{R_1}-\frac{h_1}{nR_1}\approx\frac{h_1-h_2}{d}$$ Therefore, $$\frac{h_1}{h_2}=\frac{1}{1-\frac{d}{nR_1}(n-1)}\approx1+\frac{d(n-1)}{nR_1}$$ Substituting this equation at step (4) of the derivation gives $$\frac{1}{f}=(n-1)\left(\frac{1}{R_1}+\frac{1}{R_2}+\frac{d(n-1)}{nR_1^2}\right)$$ However, I recommend not approximating $h_1/h_2$. If you approximate it, as done in the above formula, the result will be more accurate than the common lens equation, but worse than the original thick lens equation. Using it without approximation (which is better than the common and original thick lens formula), the result is $$\frac{1}{f}=(n-1)\left(\frac{1}{R_1-\frac{d(n-1)}{n}}+\frac{1}{R_2}\right).$$ Also, note that:

  • The difference in sign of the $1/R_2$ term seems to be due to sign convention.
  • The definition of $f$ here is the horizontal distance between the point which light exits the lens (which is approximately the right end of the lens) and where it is focused.
  • $R_1$ is the radius of the side the light enters the lens.
  • $R_1 \gg R_2$ approximation is also used.
Improve this answer
$\endgroup$
0
$\begingroup$

I was looking into this myself this week. A very thorough derivation can be found here: http://www.learnoptics.com/Optics%20Chapters/opticsintrochap1.pdf. Unfortunately, you will likely have to read all until page 46. Luckily, it closely mirrors the Hecht book and is very informative. Note the ray transfer matrices follow a different convention from Hecht in which the terms of the ABCD matrices are rearranged.

Alternately, a simpler derivation would be by using ray transfer matrices as shown here:https://www.youtube.com/watch?v=nNFhsmVlyeE

Improve this answer
$\endgroup$
4
  • 2
    $\begingroup$ It would be much better if you expand your answer rather than provide lightly commented links to other sources. $\endgroup$
    – ZeroTheHero
    Commented Dec 4, 2020 at 13:21
  • $\begingroup$ Here's another link with a short derivation of the formula. $\endgroup$
    – wyphan
    Commented Dec 4, 2020 at 20:53
  • $\begingroup$ @wyphan I found that link too but step 6 just says "as derived by Morgan" and refers to an obscure book. $\endgroup$
    – B Green
    Commented Dec 4, 2020 at 22:55
  • $\begingroup$ I think it goes like this from equation (4) to (6): use the relation for conjugate points (equation (5) ) to substitute $s_{o1}$, $s_{1i}$, $s_{o2}$, and $s_{i2}$ with $f$. Then, use $n_m = 1$ for air. Equation (6) is simply the final result. $\endgroup$
    – wyphan
    Commented Dec 4, 2020 at 23:00

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.