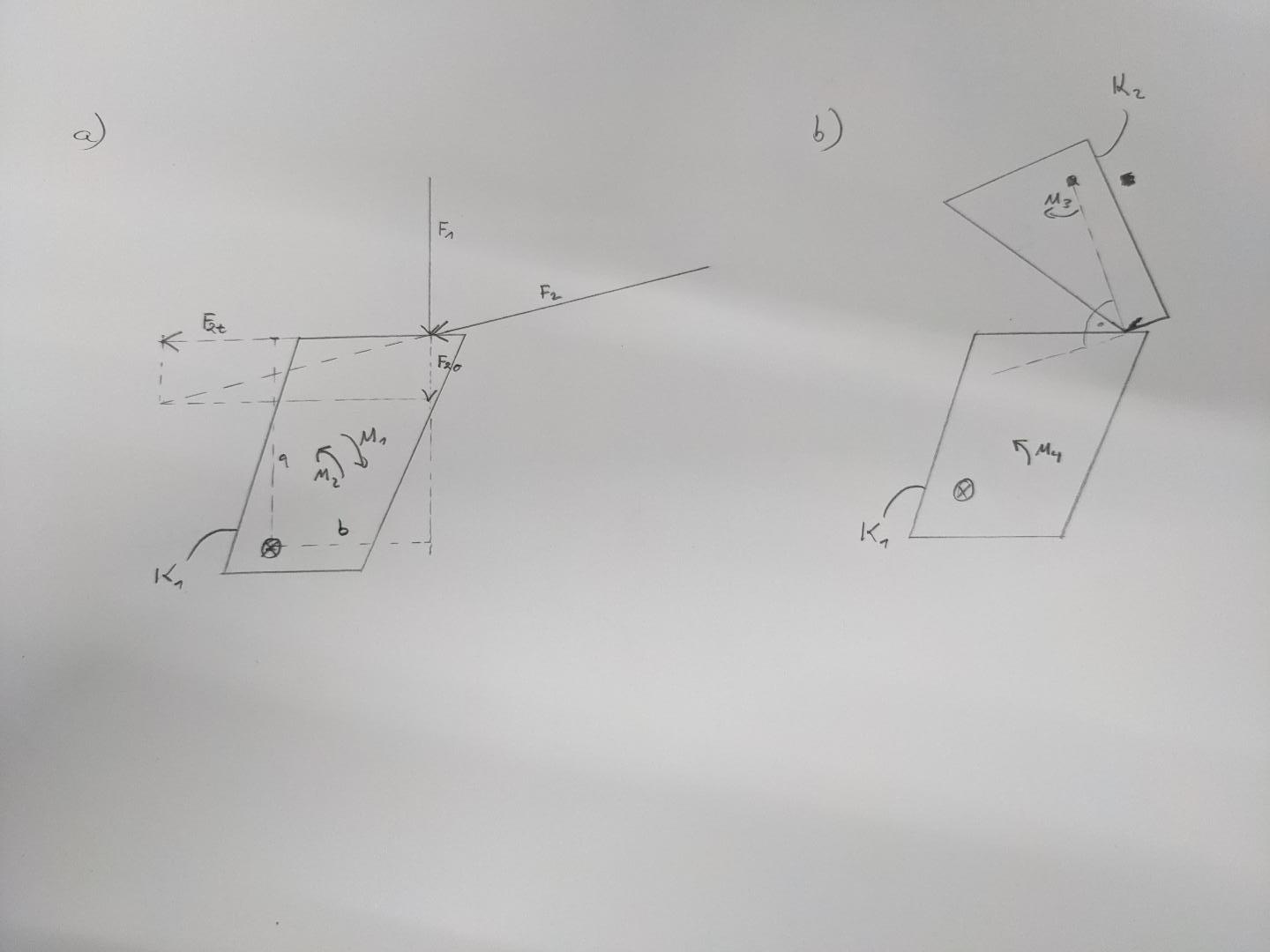
In the picture below, in a), a body K1 is pivotably attached to a bearing. My question is about the torque that results from a force exerted onto a surface of the body K1.
A first force F1 applied orthogonally onto the surface should result in a torque M1 in clockwise direction.
Is it correct that a second force, F2, applied almost parallel to the surface will result in a torque M2 in counterclockwise direction?
My thoughts are, F2 is split into F2t and F2o (transversal and orthogonal components) by the surface of the body K1. To get a torque, F2o is multiplied by the lever b and F2t is multiplied by the lever a (M2 = F2t * a - F2o * b > 0). As a>b and F2t>F2o, the torque from the force F2 results in counterclockwise direction.
Applying these thought to the two bodies K1, K2 in b), a torque of M3 applied to the body K2 will result in a torque M4 in the body K1. (The bodies won't move because they are in each others movement path)
Is this correct or am I forgetting something? What is the job of friction in this case? From looking at b), K2 should push K1 away by applying a clockwise torque, but that is wrong then, right?
Suppose there is enough friction so that no slippage occurs.