Minimal angle that will cause a cube to tip on an inclined plane

Question

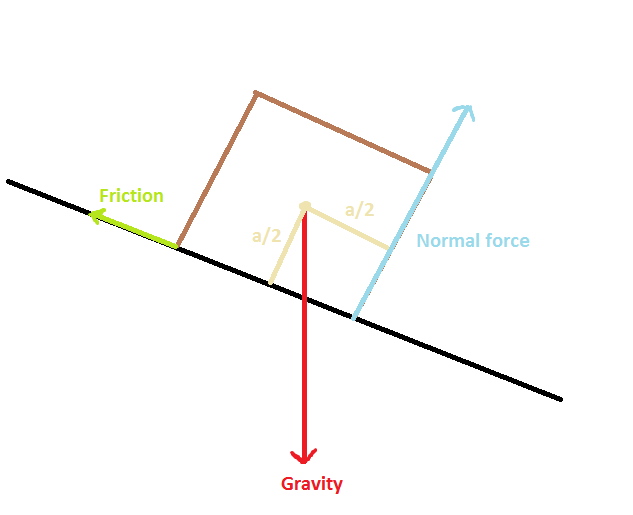
Let's say that I have a cube with side $a$ and a ramp such, that the coefficient of friction between the cube and the ramp is $\mu$.

I want to determine the minimal angle of inclination of the ramp that will cause the cube to tip over.

This is what I have established so far:

The cube will tip over when it experiences a non-zero torque. My diagram represents a critical point, that is, when the torque equals zero and increasing the angle by any arbitrary value will tip the cube.

I am computing the torque with respect to the center of mass, and so the gravity contributes nothing.

Since the cube is about to collapse, the normal force is applied to the rightmost point of it in contact with the ground.

The torque generated by the normal force: $$\tau_N = \frac{a}{2} F_n$$ Counterclockwise. Where $F_n = mg \cos(\theta)$

The torque generated by the force of friction: $$\tau_F = \frac{a}{2} F_f$$ Clockwise Where $F_f = \mu mg \cos(\theta)$

However, the net torque will equal zero iff $\mu = 1$, therefore, it does not depend on the angle - this does not match my experience.

Where is my mistake?

Answer 1

$F_f = \mu m g \cos(\theta)$

This is the maximum value of friction on an object, not necessarily the actual friction. If the ramp is horizontal, you'll calculate a large value, when the actual friction will be zero.

For the static case, the actual friction will be no larger than the component of gravity parallel to the ramp that is pulling it to the right.

I'd say that on surfaces with large friction, the cube would tip over sooner.

You didn't do the analysis this way, but in the static case, the object will never tip over unless the center of mass is to the right of the corner. In the case of a cube, that means a 45 degree angle. Raising the coefficient of friction doesn't make it tip over sooner. It just keeps it from sliding away before it tips.

If you're considering a sliding case (the block is already moving to the right with "sufficient speed"), then your analysis is already correct and the angle is irrelevant.

And a final problem (if you're trying to match what you see with actual blocks and imperfect ramps) is that the modeled friction force isn't always realistic once edges appear. If that lower corner of the cube "catches" a bump on the ramp, then the forces that appear can be much larger than what you'd calculate by the friction equation. So sharp edges are going to allow it to tumble in cases where the simple calculation says it should not.