Young slit conundrum - Finding positions of maxima when one of the slits emanates light with phase difference

Question

Second guessing myself a bit here, but I've run into a bit of an issue.

The problem I'm trying to solve is this:

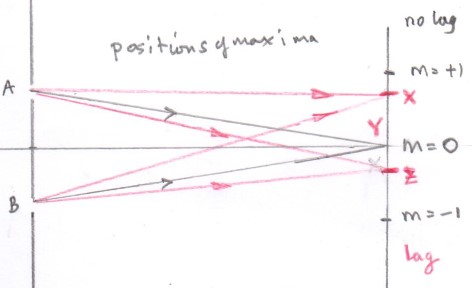
Coherent light of $532nm$ is passing through a double slit, which are separated by $25\mu m$. Due to misalignment of the setup, the light from the bottom slit lags behind the light emanating from the top, with a phase shift of $\frac{\pi}{2}$. An interference pattern is observed on the screen that is $5m$ away. Calculate the angular positions of the $0th, 1st$ and $2nd$ interfecence maxima.

Now, I went for a more "first principle" approach to try and work out the diffraction equation, so I could find the angles.

The logic I used mainly followed the derivation of the fringe maxima under normal circumstances, with the small modification:

The "new path difference" for an arbitrary point P on the screen is $\delta + \frac{\lambda}{4}$, which is equal to $d\sin(\theta)$, where $\delta$ is an arbitrary path difference, and the $\frac{\lambda}{4}$ accounts for the phase lag.

Because of the condition for maxima, I therefore said the path difference must be a whole number of wavelengths, so I fiddled with the fact that:

$$\delta + \frac{\lambda}{4} = d\sin(\theta) = m\lambda$$

I ended up going in circles a little bit from here, not too sure where to proceed, but I thought "oh, it's obvious" and wrote down that for this situation: $$d\sin(\theta) = \left(m+\frac{1}{4}\right)\lambda$$ To calculate the angular positions.

After discussing with a friend of mine, she said that this didn't take too much sense, as this was showing destructive interference, not constructive. Upon reviewing my work, I thought, "oh, why did I add the 1/4, the other wave is lagging?" and resolved to $$d\sin(\theta) = \left(m-\frac{1}{4}\right)\lambda$$

My friend then offered an explanation of "because it's 1/4 of a wavelength behind, you simply need to add 3/4 of a wavelength to the maxima criteria to get 'back in phase' ", which makes sense to me- but I was craving a diagrammatic derivation of this, if possible.

Further, I'm confused as to why my initial attempts weren't providing the (apparent) correct answer of:

$$d\sin(\theta) = \left(m+\frac{3}{4}\right)\lambda$$ At first I thought, because of the off-centre diffraction pattern, this answer and my (2nd) answer must be the same thing, but in a later question:

Find the linear density of the maxima.

We got ever so slightly differing answers.

Any pointers would really be appreciated! My head is spinning trying to figure it out...

In general, for a problem like this, would I always calculate as:

$$d\sin(\theta) = \left(m + \text{fraction of } \lambda \text{ corresponding to phase difference}\right)\lambda$$

Answer 1

The phase difference between the light from each of the slits changes by $2\pi$ from one fringe to an adjacent fringe.
Usually the bright fringed formed when there is zero phase difference is called the $m=0$ fringe at position $Y$ in the diagram.
Other bright fringes can be labelled $m = \pm 1,\,\pm2,\,\pm3, . . . . . $.

If light from the bottom slit $B$ lags behind the light emanating from the top slit $A$ with a phase shift of $\frac \pi 2$ then this corresponds to a shifting of the fringe pattern by $\frac 14$ of a fringe width shown as positions $X$ and $Z$ in the diagram.

Now where is the new zero order fringe, at position $X$ ($m=\frac 34$) or position $Z$ ($m=-\frac 14$)?
I would favour the approach of "speeding up" the arrival of waves from the lagging slit $B$ and the "slowing down" the arrival of waves from slit $A$ which can be done by decreasing path $BY$ and increasing path $AY$ and this corresponds to position $Z$.

For the waves to arrive in phase at position $X$ requires a greater path difference as $BX-AX> AZ-BZ$.

Consider being able to change the phase lag from slit $B$ continuously from zero.
What would you see?
The $m=0$ bright fringe (zero order) would be seen to move down towards position $Z$ whilst the $m=+1$ bright fringe would be moving down towards position $X$.

I read the problem Calculate the angular positions of the 0th,1st and 2nd interference maxima as Calculate the new angular positions of the old 0th,1st and 2nd interference maxima.