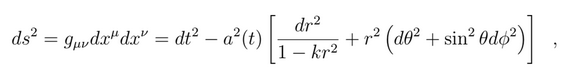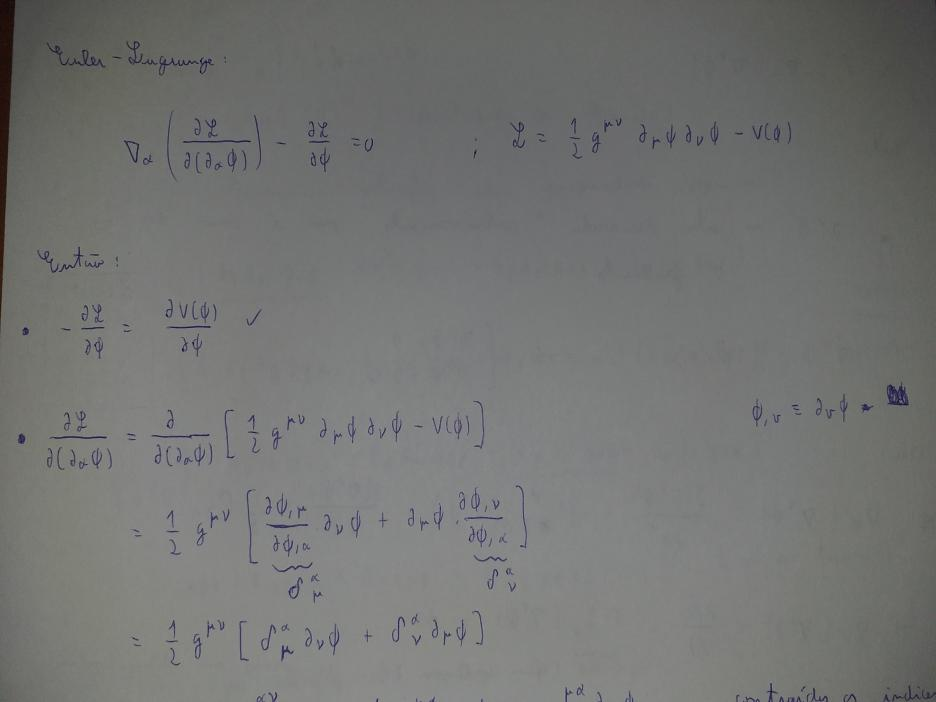Euler-Lagrange equations of a scalar field [closed]

Question

Given the Robertson-Walker metric

for a scalar field $\phi(t)$, how can we obtain the equation of motion for this scalar field?

I took the contravariant derivative of the scalar field is which is nothing more thant the gradient of that same field and then I applied the covariant derivative to that quantity, since the gradient of the scalar field is a vector, I took the covariant derivate of his components, which are by themselfs contravariant, although the spatial components of the 4-vector are zero the Christoffel are not, therefore having a component that is not zero and is $H\dot{\phi}$ ,where H is $H = \frac{\dot{a(t)}}{a(t)}$.

Finally, is this approach right? If not, how would you approach it?

Answer 1

The following hints might be useful:

1. The Euler-Lagrange equations can also be written as follows:

\begin{equation} \frac{1}{\sqrt{-g}}\partial_{\alpha}\left[\sqrt{-g}\frac{\delta L}{\delta (\partial_{\alpha}\phi)}\right] - \frac{\delta\mathcal{L}}{\delta\phi} = 0\ , \end{equation}

due to the fact that the divergence can be written in terms of the determinant of the metric tensor (see for instance Tensors - Computing the Divergence formula for a given metric tensor or https://en.wikipedia.org/wiki/Divergence). This form could be useful for diagional metrics, reducing the amount of calculations to do.

2. Although the results must be the same, there could be terms that you included and should not be there, due to the fact that $\phi$ depends on $t$ only. From the expression I wrote,

\begin{align} \frac{1}{\sqrt{-g}}\partial_{\mu}\left[\sqrt{-g}g^{\mu\nu}\partial_{\nu}\phi\right]& = \frac{1}{\sqrt{-g}}\partial_{\mu}\left[\sqrt{-g}g^{\mu\ t}\partial_{t}\phi\right]\\ & = \frac{1}{\sqrt{-g}}\partial_{t}\left[\sqrt{-g}g^{tt}\partial_{t}\phi\right]\\ & = \frac{1}{\sqrt{-g}}\partial_{t}\left[\sqrt{-g}\partial_{t}\phi\right]\\ & = \frac{\dot{a}}{a}\partial_{t}\phi+\partial^{2}_{t}\phi\\ & = H(t)\dot{\phi}+\ddot{\phi}\ . \end{align}