2
$\begingroup$

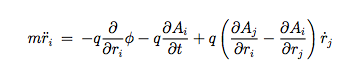
I'm a math guy, just wanting to clarify some notation. enter image description here

This is the Euler Lagrange equation associated with a charge in an electric field I found in a book. Where $\phi$ and $A$ are the scalar and vector potential related to Maxwell Equations. I am just curious, this last term, the one with the subscript $j$, is this last term suppose to be a sum over $j=1,2,3$ of that last quantity, i.e it will be three terms? I just wanted clarification for this, since I'm not sure why this sum of omitted if there should be a summation here. If there is not a summation here. Can someone tell me what $j$ is referencing.

I know i is referencing different components of the position vector.

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ Yes, it sums over $j = 1, 2, 3$. $\endgroup$
    – knzhou
    Commented May 24, 2017 at 2:50
  • $\begingroup$ Thank you for the response. Just curious in physics is this omittance of the sum typical? Does the change in subscript over another variable just imply sum? $\endgroup$
    – Vogtster
    Commented May 24, 2017 at 2:55
  • 3
    $\begingroup$ Yes, the convention is that any index repeated twice is summed. Also, if it's a regular letter (as opposed to Greek) it's over $1, 2, 3$ instead of $0, 1, 2, 3$. $\endgroup$
    – knzhou
    Commented May 24, 2017 at 2:56
  • 1
    $\begingroup$ The sum is omitted because a lot of equations would become bulky and almost unreadable with all the sums included. Einstein notation is very neat and compact :) $\endgroup$
    – koldrakan
    Commented May 24, 2017 at 7:46
  • 1
    $\begingroup$ Actually, beware, because in Special and General relativity, the implicit sum is only for "up-down" indices in tensors. For example, in $F_{\mu\nu}F^{\mu\nu}$, there is an implicit sum $\sum_{\mu=1}^4\sum_{\nu=1}^4$. But if you write $F_{\mu\nu}F_{\mu\nu}$, there is no implicit sum. $\endgroup$
    – user154997
    Commented May 24, 2017 at 8:05

1 Answer 1

Reset to default
1
$\begingroup$

I'd add a small comment, which is to give a name to the notation to possibly help in the future, but I don't have enough reputation yet.

Therefore I'm writing this as an answer: Yes, it's extremely powerful, and it's known as the Einstein Summation Convention. There are nice articles on Wikipedia and Wolfram's MathWorld on the topic.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.