Even we don't know much about scattering areas of photons and electrons does the fact that a photon scattering an electron at an angle mean that the photon cross-section area hits only a small lateral area of the electron causing it to move at an angle. If the photon cross-section area was greater than the electron's one would the electron be scattered only in the direction of the photon incoming motion because the whole its area would be uniformly objected to photon's pressure? So should the electron have a finite volume?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ See Is the zero distance limit of Coulomb's law something to worry about? $\endgroup$– mmesser314Commented Sep 9, 2020 at 13:43
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
1
Photons and electrons are elementary particles in the standard model of particle physics, SM , a quantum field theory model.
They are by axiomatic definition point quantum mechanical particles and their behavior is perfectly described by the SM. Their scattering can only be described within this quantum mechanical framework.
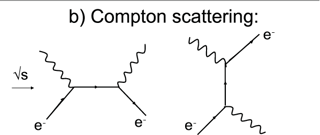
So, as the SM fits the great plethora of particle physics data and predict new observations, it is accepted that the electron and the photon do not have a crossection. Their scattering is due to the electric field of the electron and the coupling of the photon to the electromagnetic field as given by the Feynman diagrams that can compute the probability of the scattering, example:
The experiments have put an experimental limit on the "size"of the electron , see this, as < $10^{-18 } m$ , which gives a limit to the validity of the standard model.
$\begingroup$
$\endgroup$
I think the question is based on a very mechanistic idea of scattering. IN reality what is important is energy and momentum conservation. Assuming for simplicity a non-relativistic electron, this means $$ \frac{p_i^2}{2m} + \hbar ck_i = \frac{p_f^2}{2m} + \hbar ck_f,\\ \mathbf{p}_i + \hbar\mathbf{k}_i = \mathbf{p}_f + \hbar\mathbf{k}_f. $$ To satisfy the momentum conservation (i.e. the second equation) the initial and final momenta of either the electron or the photon do not have to be parallel. In fact, there are simply many more possibilities to satisfy both conservation laws when they are not parallel, i.e. when photon and electron are scattered at an angle.
