Rotational mechanics in a gyroscope question

Question

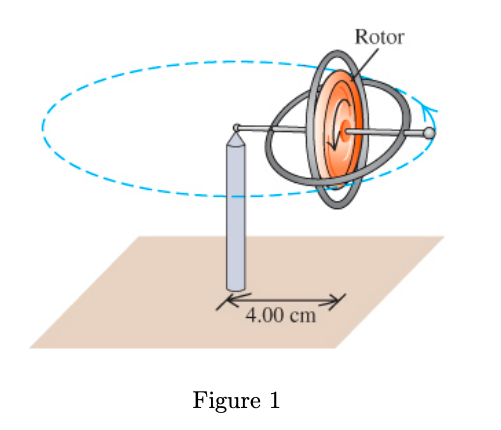
I have a homework question about gyroscope rotation, but I will describe my confusion more specifically as follows:

Below is the setup of the problem. I understand the concept that if the rotor is made to spin, then there is an angular momentum generated in the direction given by right-hand rule, in this case to the right of the page. This, combined with the torque into the page given by the object's weight, will result in the gyroscope to start rotating in a circle.

However, what confuses me is that if I am given the period of gyroscope rotation $T$ and the mass and moment of inertia of rotor, how can I then calculate the rate at which the rotor is spinning at? What kind of equation can allow me to equate these two concepts and solve for the rotor spinning speed?

Answer 1

First I will state the notation I'll use, to avoid any confusion between the two angular velocities we'll be dealing with. The mass of the gyroscope is $m$, its moment of inertia $I$ and its distance to the pivot point $r$. The angle $\theta$ denotes the angle that the gyroscope traces with respect to the pivot point, and $\Omega = \frac{d \theta}{dt}$ is what I will call the precessional angular velocity. Meanwhile $\omega_s$ denotes the spin angular velocity of the gyroscope itself. As often seen in textbooks $\vec \tau$ denotes torque and $\vec L$ denotes angular momentum.

The anwer to the question lies on the relation between the spin angular velocity of the gyroscope itself and the precessional angular velocity of the whole system. We first must realize that the spin angular momentum of the gyroscope will be given by: $$\vec{L} = I \omega_s \hat r$$ where $\hat r$ is a unit vector that points from the pivot where the rod is placed toward the center of the gyroscope at every moment. Since there is also an precessional angular velocity there will be an orbital angular momentum pointing upward (assuming that the gyroscope stays rotating in the plane perpendicular to the vertical rod), but as we will see the torque will be perpendicular to it and thus it will be constant, so it is uninteresting for the question asked.

We can also note a crucial relation in the problem, which arises in finding the total time derivative of this angular momentum vector (it is important to keep in mind that want to find $\frac{d \vec L}{dt}$ and not $\frac{d |\vec{L}|}{dt}$, since the magnitude of the spin angular momentum will be constant due to the fact that both $I$ and $\omega_s$ are constant; instead we are interested in the change of the vector, to study its rotation, directly related to the precessional angular veolcity $\Omega$) Using: $$\frac {d \hat r}{dt} = \frac{d \theta}{dt}\hat \theta$$, where $\hat \theta$ is perpendicular to $\hat r$ and in the same plane as it and $\theta$ is what has been established before. We can thus perform the time derivative of the angular momentum vector with result: $$ \frac {d \vec L}{dt} = I \omega_s \frac{d \theta}{dt} \hat \theta = I \omega_s \Omega \hat \theta$$ Thus the magnitude of this derivative is given by: $$|\frac{d\vec L}{dt}| = I \omega_s \Omega $$ But from the definition $\vec \tau = \vec r \times \vec F = \frac{d \vec L}{dt}$ we also obtain: $$|\frac {d \vec L}{dt}| = mgr$$ Since here $\vec F = m\vec g$ is the gravitational force. Equating the two: $$mgr = I \omega_s \Omega$$ Or because $\Omega = \frac{2\pi}{T} $: $$mgr = \frac{2\pi I \omega_s}{T}$$ And we can solve for $\omega_s$, the desired quantity: $$\omega_s = \frac{mgrT}{2 \pi I}$$ In summary the equation than one could have used to relate this two is $\Omega = \frac{\tau}{L_s}$, which relates the torque and the spin angular momentum of the object.

Answer 2

$\dot\psi$ is the rotation of the rotor , $\vartheta=\pi/2$ is your configuration.

you can obtain the solution of your problem from the conservation of the energy :

$$E=T+U=~\text{constant}$$ where T is the kinetic energy and U is the potential energy

for $\vartheta=0$ is $$E_0=m\,g\,h$$ and for $\vartheta=\pi/2$ is the energy $$E=\frac{1}{2}\,(I_\phi\,\dot{\phi}^2+I_\psi\,\dot{\psi}^2)$$

With

$$E=E_0$$ you can solve this equation and obtain

$$\dot{\psi}=\frac{\sqrt{I_\psi\,(2\,m\,g\,h-I_\phi\,\dot{\phi}^2})}{I_\psi}$$