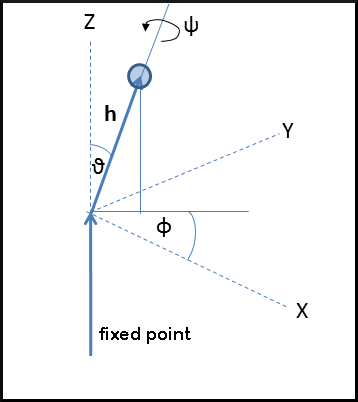
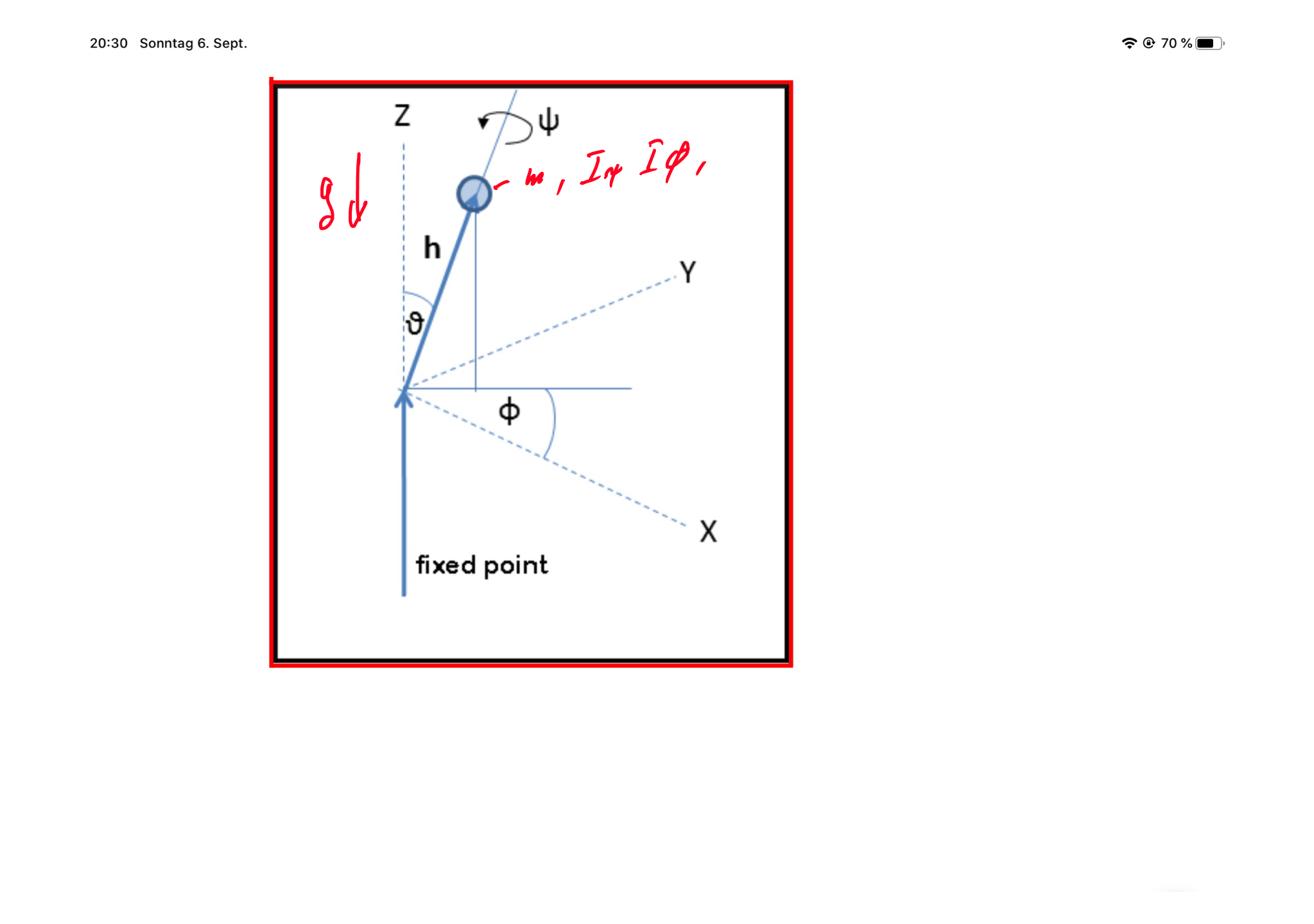
$\dot\psi$ is the rotation of the rotor , $\vartheta=\pi/2$ is your configuration.
you can obtain the solution of your problem from the conservation of the energy :
$$E=T+U=~\text{constant}$$ where T is the kinetic energy and U is the potential energy
for $\vartheta=0$ is $$E_0=m\,g\,h$$ and for $vartheta=\pi/2$$\vartheta=\pi/2$ is the energy $$E=\frac{1}{2}\,(I_\phi\,\dot{\phi}^2+I_\psi\,\dot{\psi}^2)$$
thus:With
$$E=E_0$$ solveyou can solve this equation for $\dot{\psi}$ youand obtain :
$$\dot{\psi}=\frac{\sqrt{I_\psi\,(2\,m\,g\,h-I_\phi\,\dot{\phi}^2})}{I_\psi}$$