What makes a wrist-energized gyroscope rotate faster?

Question

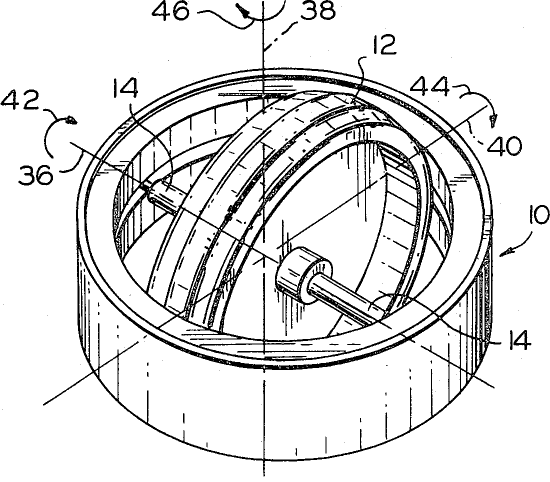
I'm considering a wrist-energized gyroscope, shown below (after my daughter let it fall and it broke open). That one was sold as Roller Ball, but variants are known as Powerball, DynaBee, Dynaball, Dyna-Flex, Wrist Ball, NSD Spinner..

There are essentially 3 relatively moving parts:

• the rotor (yellow) and its (metal) shaft;
• the washer (white);
• the casing (green); normally its two halves are glued together. There's a circular groove in the joint of the casing. The groove holds the washer and rotor, is larger than the washer's thickness, and slightly larger than the diameter of the shaft at its extremity.

There are essentially 2 degrees of liberty:

• The rotor can spin w.r.t. the washer, with little friction, around the rotor's axis. It also touches the casing's groove, on which it can roll with some friction. Update: the diameter of the shaft is reduced to 2.5 mm at its rolling extremity.
• The washer can rotate w.r.t. the casing, with some friction, around an axis perpendicular to the plane of the casing's groove. Update: its outer diameter is 60 mm.

The two axis are perpendicular and coplanar.

To operate the device, the rotor is given an initial spin-up (one method is using a string wound on the rotor; that's the sole purpose of the groove on the rotor, and of the big hole in one side of the casing). Then the device's casing is held firmly in the hand, and its orientation changed in a certain manner, with the effect that the spin rate of the rotor increases, reportedly well over 10,000 RPM for skilled operators.

I conjecture that the washer is non-essential, as the device was first described without it in US patent 3,726,146, from which the following illustration is taken. .

---

Question 1: What causes this increase of the spin rate of the rotor? Update: In particular, if that involves friction between casing and rotor, how come that increases the spin rate, even though the casing is NOT rotated faster than the rotor (in a rotation movement with the rotor's axis), giving some credibility to the argument that friction of rotor on casing can only slow the spin rate of the rotor?

Question 2: Is there a convincing argument that if the rotor was practically frictionless (and not touching the casing), the spin rate of the rotor could not be increased?

---

Update: I think the rotor is rolling (rather than sliding) on the casing's groove, and now realize that this friction is instrumental in spinning-up the rotor: when the casing's grove gets polished, or worse greasy or oiled (reducing friction), it becomes harder or impossible to spin-up the rotor.

When the rotor is spinning steadily with the casing passively held, and I observe the washer (or equivalently the rotor's axis), there are clearly three discrete modes:

• washer does not move (relative to casing);
• washer rotates at constant speed (decreasing steadily with the rotor spin rate) in one direction;
• washer rotates at same constant speed in the other direction.

This is consistent with the hypothesis of a rolling of the rotor on casing, each extremity of the rotor rolling on a different half of the casing, with the direction of the rotation depending on which extremity of the rotor rolls on which half of the casing (depending on how the casing is held/moved, there is an occasional quick inversion).

At "cruse" speed, I guesstimate the spin rate of the rotor on its axis to be perhaps 100 rotations per seconds within a factor of five, based on the sound/vibration (that's about consistent with this stating 250 Hz as a high spin rate); and the washer's spin rate to perhaps 5 rotations per seconds, based both on observing it when I stop moving the casing, and actuating the casing at a rate of 10 times per second to maintain/increase the spin rate (that casing excitation period corresponding I think to a half turn of the washer). Given how crude these spin rate estimates are, the ratio 100/5 of rotational speed is rather close to the ratio 60/2.5 of diameters.

Answer 1

You seem to be saying that friction couldn't speed it up, because nothing else is moving that fast. Well, how fast is it moving?

We can imagine the gyroscope axis parallel to the z axis, and the casing to be aligned such that the x axis goes through it. If the casing is tipped slightly, the gyroscope resists that turning and one side of the shaft has firm contact with the side of the groove.

Now, I cannot tell easily from the photo the size of the shaft portion within the groove, but it appears to have a radius to me no larger than about 4mm. If we can imagine that the rotor has a speed of 4000rpm, what would that translate to at the edge of the shaft contact point?

$$v = {\omega r}$$ $$v = {4000rpm \times 2mm}$$ $$v = {418.9 radians/s \times 0.2cm}$$ $$v = 83cm/s$$

What's the diameter of the groove? Maybe 3.5 inches? If so the circumference would be about 27cm. So if you could spin the thing in way that made the axis tumble more than 3 times a second, then the motion of the axis would be such that it is carrying the edge of the shaft past the groove faster than rotation is carrying it backward. Friction would serve to increase the rotation, not decrease it.

You'll have to let me know if these assumptions are correct. I appear to have made guesses about the size of the shaft in the groove, the speed it can rotate, the diameter of the groove, and the rate of "tumbling" that the rotor does when you are accelerating it.