How does angular momentum contributes to increase the normal force?

Question

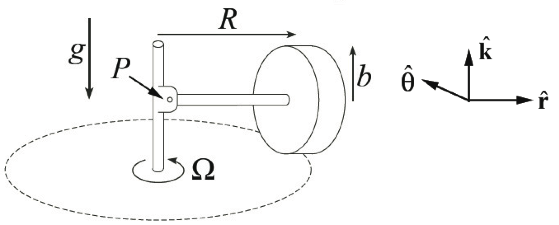
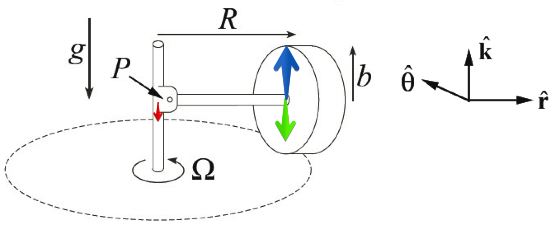
I found the following in an exercise of gyroscopes:

In a grain mill, grain is ground by a massive wheel that rolls without slipping in a circle on a flat horizontal surface driven by a vertical shaft. The rolling wheel is constrained to roll in a horizontal circle about the vertical axis. Because of the stone's angular momentum, the contact force with the surface can be considerably greater than the weight of the wheel. In this problem, the angular speed about the shaft is such that the contact force between the ground and the wheel is equal to twice the weight.

How is the angular momentum working so that the force of the wheel on the floor is magnified, to be twice the weight of the wheel?

--

A way that I have thought of it is that the angular momentum is in the $ -\hat{r} $ direction and to move the wheel in the way described in the image a torque is required in the $ -\hat{\theta} $ direction. Looking at the forces in play on the wheel, this can only be provided by the normal force being greater than the weight.

But, wouldn't that mean that the wheel would experience a very unlikely, intuitively speaking, acceleration vertically upwards?

Also, this would mean that the wheel moves in a sort of precessional motion, which leaves me wondering if there's a static friction force acting on the point of contact?

Answer 1

1. Angular speed doesn't affect weight (at least from a classical mechanics perspective). Weight is a gravitational force that is conservative, i.e depends only on the position of massive objects in space, not velocities.

2. Reaction force from the ground is indeed greater than the weight during the precession. If one quickly snaps the rod connecting the wheel to the shaft, the wheel will indeed jump upwards.

3. However, during the normal operation the wheel doesn't have vertical acceleration because weight $\color{green}{mg}$ and ground reaction $\color{blue}{N_G}$ aren't the only vertical forces acting on the wheel. There is also a reaction force $\color{red}{N_S}$ from the shaft. One may say that additional ground reaction and the shaft reaction form a couple. This couple has no effect on linear acceleration but provides the torque needed.

4. There's indeed a static friction force between the ground and the wheel. But it's not due to precession. If there is no friction, the wheel wouldn't be rolling in the first place and the motion would be a simple rotation around the shaft. This force creates the torque that is needed to provide initial angular momentum in the direction of the shaft (when the system starts to move). However, during the operation under constant angular velocity, the friction force can be considered zero if there is no friction in the bearing. In reality, there is friction in the bearing which tries to stop the wheel from rotating, so the wheel-ground friction creates the torque to keep the rotation.

Answer 2

When viewed from above, the wheel goes CCW around the vertical axis. As this happens, the angular momentum vector (which points at the vertical axis) must swing CCW. This requires a torque vector in the direction of swing. To produce that torque (about the center of the wheel), the vertical axis must push down on its end of the horizontal axle. To transmit this downward force to the wheel, the horizontal axle must be rigidly welded to the vertical axis. Then: FR = Iω(dΏ/dt) = (1/2)m$b^2$(ω)(bω/R). To double the normal force set F = mg and solve for ω.