Two Polytropic and Two Adiabtic processes in Thermodynamic cycle [closed]

Question

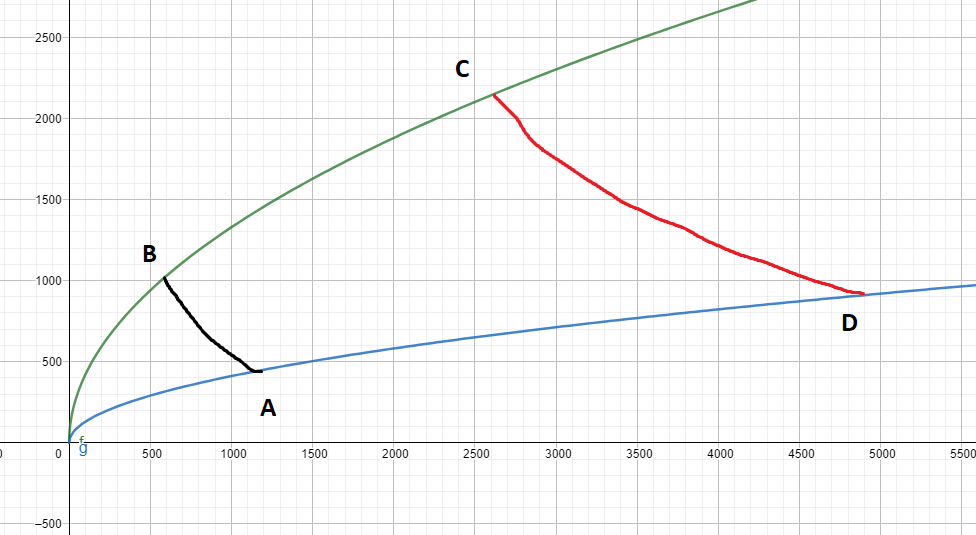
$1$ mole of an Ideal gas performs a reversible cycle with 2 adiabatic and 2 polytropic processes.

$A(P_0,V_0,T_0)$ to $B$ with $V_B=V_A/\lambda$ with an adiabatic process.

$B$ to $C$ with a polytropic process where $P=C_1V^{1/2}$

$C$ to $D$ with an adiabatic process.

$D$ to $A$ with a polytropic process where $P=C_2V^{1/2}$

Find the cycles efficiency and draw the PV diagramm of the cycle.

Solution:

Since there is heat exchange only during the two polytropic processes we can easily calculate the efficiency to be $η=1-\frac{Q_{out}}{Q_{in}}=1-\frac{T_C-T_B}{T_A-T_D}$ since we know $C_{23}=C_{41}=C$ since the processes share the same $k=-\frac{1}{2}$.

$T_B=1.6T_A$ can easily be calculated using the fact that AB is adiabatic and PV=RT.

My problem is that I can find no way to calculate sates C and D. This is because i have only 3 equations to calculate $(PC,Vc,Pd,Vd)$. These equations are:

$P_C=C_1V_C^{1/2}$

$P_D=C_2V_D^{1/2}$

$P_C V_C^{γ}=P_D V_D^{\gamma}.$

So the two polytropic processes could end anywhere and $T_C$,$T_D$ cannot be determined. Same goes for the Pressure and Volume at those points. What I am saying is that in this $P-V$ diagramm the red line could be moved anywhere to the left or to the right and where exactly it is affects the efficiency of the cycle.

I tried using the fact that $\Delta U=0$ for the whole cycle but it didnt get me anywhere. Is there anything I'm missing?

Answer 1

The input data don't seem to be consistent. For the adiabatic leg from A to B, we must have $$\frac{P_B}{P_A}=\left(\frac{V_A}{V_B}\right)^{\gamma}=6.4086$$From the polytropic equations, we must have $$P_A=13V_A^{1/2}$$and$$P_B=42V_B^{1/2}$$implying that $$\frac{P_B}{P_A}=\frac{42}{13}\left(\frac{1}{4}\right)^{1/2}=1.615$$

Answer 2

Here is a version of the problem that is consistent. Maybe you would consider solving this one?

Let $\lambda = V_A/V_B$, and let the polytropic relationships be given by:

$P=C_1V^{1/2}$ for D to A

$P=C_2V^{1/2}$ for B to C

1. In terms of $\lambda$ and $\gamma$, for a consistent formulation, what is the ratio $C_2/C_1$?

2. Show that $\frac{V_D}{V_C}=\frac{V_A}{V_B}$, and thus that $\frac{V_D}{V_A}=\frac{V_C}{V_B}=r$

3. Derive an expression for the efficiency of the cycle exclusively in terms of $\lambda$ and $\gamma$

Answer 3

This is a homework and exercise problem and the rules are solutions should not be given. I haven't attempted to follow your analysis, but here is some general guidance that may be of help.

Both the reversible polytropic and reversible adiabatic process for an ideal gas has the form

$$PV^{n}=C$$

where $C$ = constant.

For the adiabatic process,

$$n=\frac{C_{P}}{C_{V}}$$

Which equals 1.34 for the adiabatic processes, per your information.

I suggest you combine these equations with the ideal gas equation which, for one mole of gas, is

$$PV=RT$$

Which applies to the equilibrium states A, B, C and D, and you may be able to determine the properties at states C and D.

Good luck.