Do we have good reasons to believe that neutrino oscillations aren't the result of a new particle? If so, what are they?

Question

The standard way of describing neutrino oscillations seems to be that the mass eigenstates differ from the flavour eigenstates, which leads to an oscillation of neutrino flavour over time.

However, every other change in the Standard Model seems to be mediated by a gauge boson, so I was wondering why neutrino oscillations were somehow the exception to this, changing flavour all on their own without any other interactions. Have physicists ruled out the possibility of a gauge boson being absorbed/emitted in the process of neutrino oscillation causing the neutrino to change flavour, and if so, how? (Or, is there an even simpler theoretical reason why this is unlikely to be the case?) Or are there theories out there which propose an extension to the Standard Model with some kind of a mediating gauge boson for neutrino oscillations?

Answer 1

The reason that no other Standard Model particles oscillate into each other is that they are defined not to.

An "oscillation" is another name for an interaction in which one particle goes in and one particle goes out. In the standard model (after electroweak symmetry breaking) any fermion of a particular type (neutrino, electron, up, down) can oscillate into a fermion of the same type and a different generation. For each such oscillation there's a coefficient which governs the rate at which it takes place. These coefficients appear quasirandom and we have no idea where they come from. The coefficients for a particular fermion type can be written in a 3×3 matrix. That matrix can be diagonalized by changing the basis for the fermion fields, in the abstract 3-dimensional space of flavors. For all fermions except the neutrinos, that's what we do: we simply define the three particles to be the three stable modes of oscillation.

With the neutrinos, partly for historical reasons (we didn't know there was an oscillation matrix for them) and partly for practical reasons (it's the only way we observe them experimentally), we chose the basis instead to diagonalize a different matrix, the one governing the weak interaction with the electron-type fermions. An electron/mu/tau neutrino can only interact with an electron/muon/tauon respectively, by definition.

The non-oscillating neutrinos (the mass eigenstates) are unimaginatively named 1, 2, and 3. If you take those as your three neutrinos then the leptons are just like the quarks: none of them oscillate, but they do change generation in weak interactions. The reason solar neutrinos arrive in all three generations is not that they change generation en route, but rather that some of them change generation on emission in the sun and some change generation on absorption in our detectors. Although it sounds like a completely different explanation, it's the same physics in different words.

Before electroweak symmetry breaking, the "oscillation" of fermions is actually a three-way interaction with the Higgs field, so it is mediated by a boson, though it isn't a gauge boson.

Answer 2

Actually, if you are trying to contrast neutrino oscillations to the paradigmatic $\bar K^0-K^0$ strangeness oscillations, they are both traceable to the W-boson interactions, so no new particles needed. (You only need new GUT intermediators for the highly speculative $\bar n -n$ oscillations.)

It is assumed you are familiar with the WP background on Neutral particle oscillations and Neutrino oscillation, as you assert.

In both cases, the respective particles are produced in a "flavor eigenstate", that is a superposition of mass eigenstates dictated by how the weak charged vector boson (W) couples to fermion currents in a manner athwart different generations, thus violating a quantum number (e/μ/τ -ness; respectively, s which turns into u whence an $\bar s$ in a celebrated doubly weak box diagram, 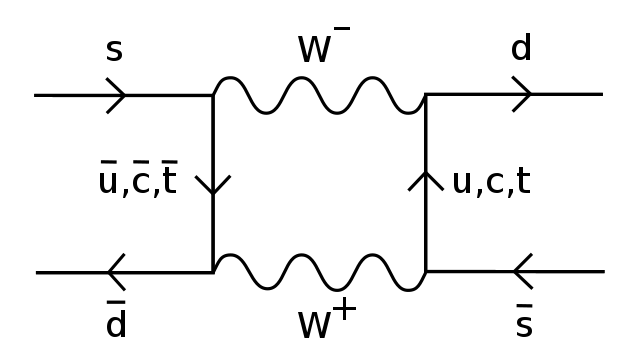. The non-diagonal mass matrix so engendered is then diagonalized, ready for propagation).

Once produced, the mass eigenstates propagate independently, and their phases have shifted when they are detected by a reverse weak process, also detecting "flavor eigenstates" (actually, mass eigenstates of the weak isodoublet partner fermions, perversely used to anchor/tag "flavor".)

In conclusion, neutrino oscillation is quite similar to strangeness oscillation; it is just that, in the former case the propagating states are ultralight fermions, and in the latter one relatively heavy bosons (pseudo scalar mesons), a billion times heavier. They all fit into the standard model quite well at present.

While novel, unexpected, interactions are conceivable for neutrinos, their oscillation by itself does not even hint at, let alone necessitate such!

Neutrino production and absorption interactions are purely weak, W induced, if that is what you are asking in part of your question. Deep inelastic scattering experiments starting in the 1970s have provided more detail on that aspect of neutrinos than any other one!

Answer 3

In the Rishon model neutrinos are seen as combinations of two truly elementary particles (T-rishons and V-rishons). In this case, the standard model has wrongly identified the elementary fields, i.e. the lepton and quark fields.
Because the elementary fields (according to the linked model) in the standard model are wrongly identified, though this isn't apparent in the experiments (yet?), the weak and electromagnetic interactions are wrongly described as a unified force, the electroweak force (in a rather unNatural and forced way).
There is instead a fourth force, the hypercolor force, which makes the W- and Z-vector bosons for the weak interaction stand on the same foot as the pi mesons in the old strong force.

So the neutrino oscillations can be considered as $VVV$ combinations and excitations there of (the three different neutrinos in the rishon model that go along with the electron, muon, and the tau, all being $\bar T\bar T\bar T$ combinations and excitations there of)
that change into one another.
The weak force is considered a residue force. The underlying force being the hyper color force, as the underlying force of the old strong nuclear force was the color force. The hyper color force is much stronger that the color force.
Untill now the standard model is doing well right now, but it remains to be seen what happens at higher energies.