Following my above comment I'll try to give a more comprehensive answer now:
The "standard" mechanism which is usually considered when talking about neutrinoless double beta decay ($0\nu\beta\beta$) is the so collad mass-mechanism shown in the picture below:
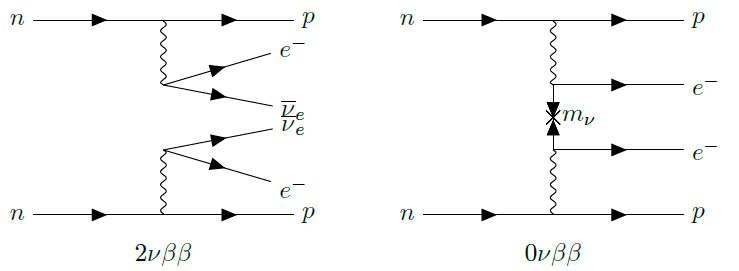$2\nu\beta\beta$) diagram vs the standard mechanism of neutrinoless double beta decay ($0\nu\beta\beta$) mediated by the exchange of a light Majorana neutrino." />
This mechanism can take place if the neutrino is a Majorana particle. The diagram involves a chirality flip which is proportional to the effective Majorana mass of the electron neutrino. That is, this mechanism follows both statements (1) and (3).
Comment on (1) + (3): Statement (1) in fact implies statement (3). Talking about a massless fermion being Majorana or not does not make much sense. You can describe massless fermions as Dirac, Weyl or Majorana equivalently
So what is it about statement (2)? As I said above, the exchange of a light Majorana neutrino is "just" the standard most straight-forward mechanism to generate $0\nu\beta\beta$ decay. There are many other lepton number violating extensions to the standard model of particle physics which can do the same. One famous example is the so-called minimal Left-Right symmetric Standard Model (mLRSM). As you may know the Standard Model of particle physics is based on the gauge group $SU(3)_C\times SU(2)_L\times U(1)_Y$ where the $L$ in $SU(2)_L$ signals that the corresponding gauge bosons only couple to left-chiral particles. It looks quite weird that nature chose to distinguish between left and right chiral particles and because physicists like symmetries the mLRSM was introduced as a possible extension to the Standard Model. All it does is make sure that the Standard Model is symmetric under a transformation $L\rightarrow R$ i.e. all right and left chiral particles should behave the same. Therefore one introduces an additional gauge symmetry $SU(2)_R$ and 3 right-handed neutrinos which we will call $N$ to distinguish them from the standard left-handed $\nu$ (the Standard Model only incorporates 3 left-handed neutrinos) + you need to change the Higgs sector of the theory but that's details. If you do this you find that additionally to the standard left-handed weak interaction you have a right-handed counterpart. Also neutrinos in this model are again Majorana. Therefore, instead of having the Majorana mass term generate the chirality flip in this model this can be done by the right-handed $W$-boson and the decay amplitude is no longer proportional to the neutrino mass. This is how the diagram looks
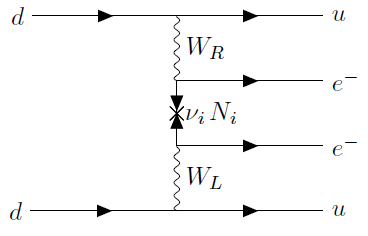$0\nu\beta\beta$" />
However, already in the mLRSM you have not only this diagram but actually 4 different types of contributions
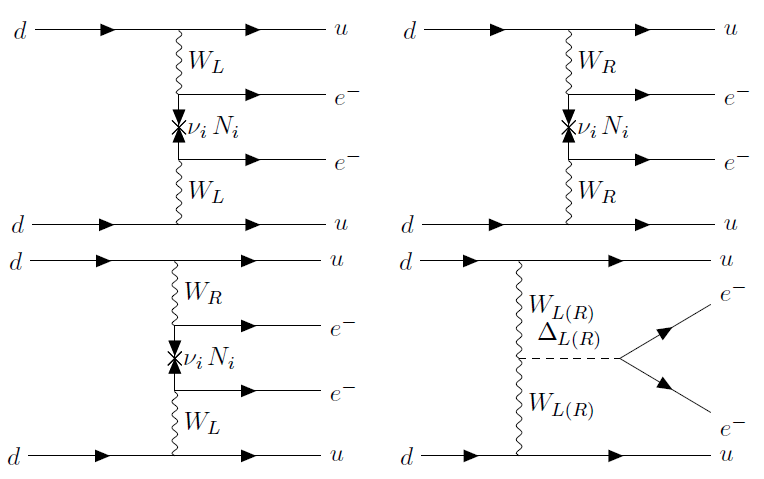$0\nu\beta\beta$ in the mLRSM. Upper left: Standard neutrino exchange diagram. Upper right: same but with right-handed current. Lower left: Both left and right handed currents. Lower Right: Contribution from the scalar triplets." />
Please take a special look at the lower right diagram. As I noted before you have to also add some new scalars to the Higss sector. Those scalar triplets can also generate $0\nu\beta\beta$ decay. However, in this diagram there is no neutrino involved. This is what you would call a short-range mechanism. It does not necessarily involve a right-handed current. Instead of exchanging a light-neutrino the new heavy scalar $\Delta$ takes care of lepton number violation.
Effective field theory approach:
As we just discussed there can be many different mechanisms. It is convenient to summarize them in terms of an effective field theory approach (EFT). An effective field theory is something like Fermi's theory which describes the weak interaction without the use of $W$ and $Z$ bosons. In low-energy regimes where neither the $W$ nor the $Z$ can be produced (because they are too heavy) the interactions they mediate as virtual particles look like point interactions. Those are described in terms of an EFT. You can categorize them into 3 different types of diagrams (just ignore (c)). The big blobs represent effective interactions that are generated from some new heavy particle. The numbers within the blobs are the dimensionality of the effective operator (you can ignore this number for the sake of this discussion)
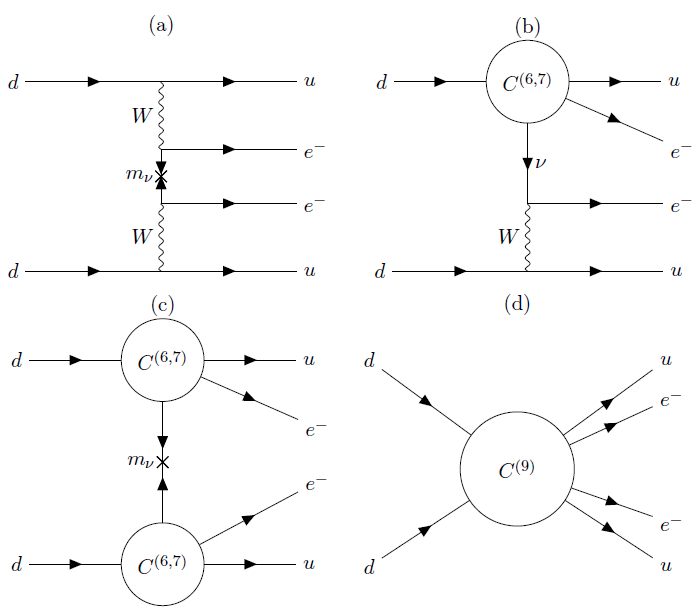
(a): Standard mechanism
(b): long-range mechanisms (they involve the exchange of a light neutrino but without the insertion of a chirality flip originating from the neutrino mass)
(c): short-range mechanisms (they do not involve the exchange of light neutrinos. Instead they are generated by the exchange of some heavy particle and therefore those interactions look like point interactions at low energy, hence short-range)
Leptoquarks: Statement (2) is one possibility of a long-range mechanism. However, there are also other long-range mechanisms e.g. mediated by scalar particles that do not involve the introduction of a new gauge boson. One simple example is the introduction of scalar (or vector) leptoquarks $S$ (or $V$). The diagrams look like this:
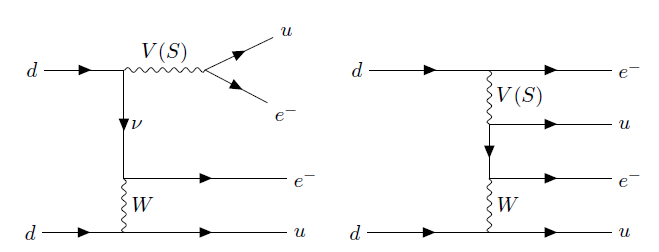$0\nu\beta\beta$ generated by scalar (S) or vector (V) leptoquarks" />
You see that here no additional right-handed weak current is necessary.
Ok but if there are other mechanisms then how are (1) and (3) correct? As I said, short-range mechanisms do not involve any light neutrino. Therefore you might wonder why statements (1) and (3) are still correct. The reason for this is the so-called black-box theorem by Schechter and Valle. The key takeaway is that you can use the diagram of $0\nu\beta\beta$-decay to generate a Majorana mass term for the neutrino.
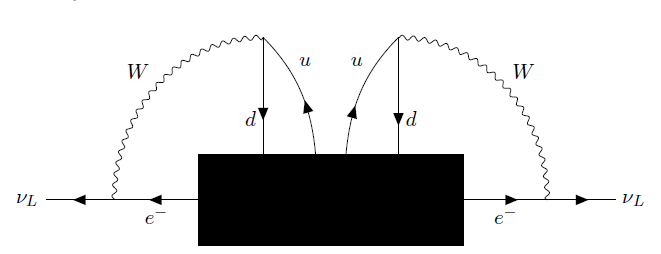For this it is completely irrelevant what mechanism within the black box is causing $0\nu\beta\beta$. As soon as you observe the decay you know that the neutrino is a Majorana fermion.
Comments: If you need the references for the diagrams I can add them. Also there might be typos in the diagrams. It's a year ago or so that I made them...
tl;dr Statement (2) is wrong. There can be other mechanisms that generate $0\nu\beta\beta$ like e.g. leptoquarks or any short-range mechanism that doesn't even involve the exchange of neutrinos between the 2 nucleons. Statement (3) is redundant because if (1) is true then (3) is also true. Statement (1) is true because of the black-box theorem by Schechter and Valle.
