0
$\begingroup$

I'm trying—unsuccessfully, as of yet—to follow how the second half of equation 1.56 is derived in Solid State Physics by Ashcroft and Mermin (equations below). Please keep in mind that I'm using this inaccurate derivation of thermopower for a high school research essay, so I'd appreciate it if things could be explained as simply as possible.

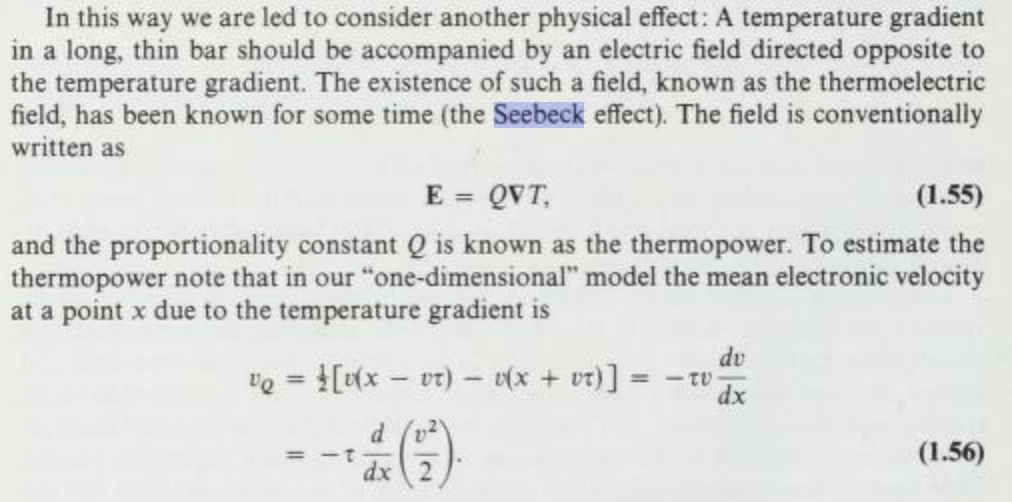
Ashcroft and Mermin are essentially deriving an estimate of thermopower in the Drude model of conduction in a metal. As part of this, they derive an equation for the average velocity at a point in a metal bar under a temperature gradient, using v(x) to denote the average velocity of electrons at point x: ctricit

How is it that $\frac{1}{2}[v(x - v\tau) - v(x + v\tau)] = -\tau v \frac{dv}{dx}$?

Improve this question
$\endgroup$
1
  • 3
    $\begingroup$ That is a math question. But remember how derivatives are defined. $\endgroup$
    – user137289
    Commented Jul 29, 2020 at 15:15

1 Answer 1

Reset to default
1
$\begingroup$

Remember that $v(x+a) \approx v(x) + a\cdot v'(x)$ in the limit of small $a$. The rest should follow immediately.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.