Why does charge flow when a conductor is 'touching' the surface of the earth?

Question

If I have a sphere of charge Q and I ground it then the charges would flow from the sphere to the earth because Earth's potential is zero. But the potential difference between the two ends of the connecting wire would be distance dependent(?).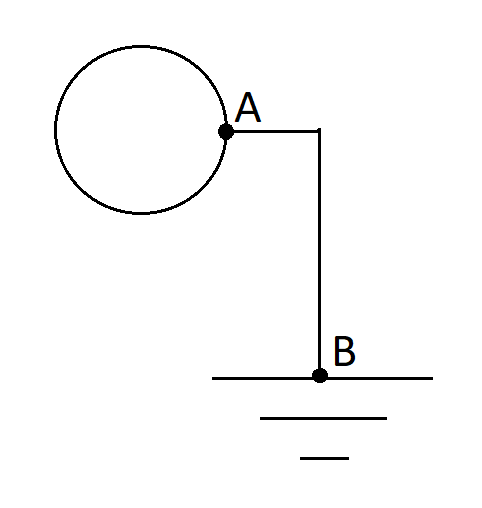

As in the figure the potential at $A$ would be potential due to the sphere and the earth i.e. $\frac{KQ}{r}$, where the radius of the sphere is $r$. And the potential at $B$ would be $\frac{KQ}{x}$ where $x$ is the separation between the sphere's centre and the point $B$. Also we can see that the potential contributed by the earth would be zero as earth doesn't have charge comparable to its radius. So, the Potential difference becomes: $$V=\frac{KQ}{r}-\frac{KQ}{x}$$ And as $x$ approaches $r$ (the conductor touches the surface), the potential difference should become zero and no charge should actually flow. But this isn't the case as charges do flow and potential of the sphere eventually becomes equal to zero. So, what am I missing here?

Answer 1

Potential Difference is similar to Temperature Difference in Thermodynamics. When we talk about potential by convention we consider the potential at infinity to be zero. So you are right when you say that the potential of the surface of sphere is $KQ/R$. But the potential of point b is not $KQ/X$. The potential of ground is considered to be $0$ since it is considered that ground is neutral. In your case the end points are the surface of sphere and a point on wire just above the surface of ground. But on the ground the potential is $0$. So as we go closer closer to the surface of ground,the current in wire would reduce but between the end of the wire and ground potential difference still exists therefore ,charge will still flow. I hope it helps!!