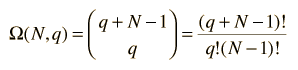Does the number of accessible microstates decrease overall when heat is transferred?

Question

We have two systems of ideal gas with different temperatures. $N$ & $V$ are being kept constant. The number of accessible microstates of each gas is thereby only influenced by a change in $E$.

The number of accessible microstates is: $$\Omega = \frac{(N-1+U)!}{(N-1)!\,U!}. $$

In regards to $E$ the function is growing at an increasing pace. Since all the energy is kinetic energy this means that the number of accessible microstates further only depends on the temperature.

Now we connect the two systems for only an extremely short amount of time, so that they keep their respective volumes and number of particles. Just a long enough timeframe that a small amount of $Q$ can be transferred from the warm system to the cold system.

This decreases the number of accessible MS in the warm system and increases the number of accessible MS in the cold system. Since $\Omega$ increases rapidly with $E$ this means that the change in the warm system is bigger than the change in the cold system. So if the decrease of MS in one system is bigger than the increase in the other the number of accessible MS overall is decreasing.

How is that possible if we know the number of accessible MS should always increase as stated by the 2nd law of thermodynamics?

kind regards

Answer 1

I think the main confusion here is that you use $\Omega$ and the entropy interchangeably. $\Omega$ is not directly proportion to entropy. Rather $S$, the entropy, is proportional to $\log \Omega$. Let’s call the two systems you have $A$ and $B$. The entropy is additive $S_{tot} = S_A + S_B$. However the total number of states isn’t, rather we have $\Omega_{tot} = \Omega_A \Omega_B$.

Now to answer your question, suppose system $B$ has more energy than system $A$ (and hence higher temperature) and let’s allow the two systems to exchange energy and see what happens to the total number of states $\Omega_{tot}$. As you mentioned, as $B$ loses energy and $A$ gain that energy, $\Omega_A \rightarrow \Omega_A + \delta_A$ and $\Omega_B \rightarrow \Omega_B - \delta_B$ and so, $$\Omega_{tot} \rightarrow (\Omega_A + \delta_A)(\Omega_B - \delta_B) = \Omega_{tot} - \Omega_A \delta_B + \Omega_B \delta_A, $$ here I’m only keeping first order terms of $\delta$’s. So you see the all very important point is that we don’t directly compare $\delta_A$ and $\delta_B$ but rather $\Omega_A \delta_B$ and $\Omega_B \delta_A$. Indeed as you mentioned, $$\delta_A < \delta_B,$$ however $$\delta_A \Omega_B > \delta_B \Omega_A,$$ and so the total number of states does increase. You can check this directly from your formula, but I’ll give a more physical reason here. The condition $\delta_A \Omega_B > \delta_B \Omega_A$ also mean $\delta_A / \Omega_A > \delta_B / \Omega_B.$ It’s not hard to see that $\delta / \Omega \propto \frac{d}{dU} \log \Omega(U)$. Not to get much into statistical mechanics details, but $\frac{d}{dU} \log \Omega $ is a decreasing function of the energy, in fact $\frac{d}{dU} \log \Omega \propto 1/T$, and so since system $A$ has lower temperature, it will have the higher ratio of $\delta/\Omega$.

Answer 2

I think it's always good to keep in mind that these "accessible" microstates and their "increase" or "decrease" are not real physical properties or processes of the system. Strictly speaking the system has only one accessible microstate at time $t+\mathrm{d}t$: namely the one determined by its microstate at time $t$ and the equations of motion.

The accessible microstates are those that you consider most likely candidates to be the actual microstate, when you don't know the latter. This set is determined by the macroscopic information you have – in this case the total energy, volume, and number of molecules. If you have different information, their number is different. If you have full information, their number is 1.

It may happen that during that brief contact energy passes from the system with lower mean kinetic energy to the one with higher.

Coming to your question, the total number of likely microstates before contact is $\varOmega(E_+)\times \varOmega(E_-)$, where $E_+$ is the energy of one system and $E_-$ of the other.

The change in this number for a small exchange of energy is $$-Q\;\frac{\partial \varOmega(E)}{\partial E}\Biggl\rvert_{E=E_+} \times \varOmega(E_-) + Q\;\varOmega(E_+) \times \frac{\partial \varOmega(E)}{\partial E}\Biggl\rvert_{E=E_-} \;,$$ where $Q>0$ is the small amount of energy exchange, the same for the two systems apart from its sign.

If compute this you'll see that the change in total number of likely microstates is positive from the fact that $E_+ > E_-$.

Answer 3

The formula is valid for units of energy

The multiplicity $Ω$ for q units of energy among N equally probable states is given by the expression

This is sometimes called the number of microstates for the system.

Organic life exists because it exchanges energy and diminishes entropy by using the environment it finds itself in. It is only in closed systems that entropy always increases. Crystals appear because the total entropy is conserved or grows, it diminishes in the crystal and increases in the environment.

The error is in the imaginary experiment:

Now we connect the two systems for only an extremely short amount of time, so that they keep their respective volumes and number of particles. Just a long enough timeframe that a small amount of Q can be transferred from the warm system to the cold system.

If they "keep their respective volumes and number of particles" how can a unit of energy be transferred?