Comparing the entropy of diffusion calculated clasically and statistically

Question

Consider first a closed system divided into two subsystems A and B, separated by a fixed, adiabatic and impermeabler wall. These have a different amount of the same ideal gas, $n_{i,A}$ and $n_{i,B}$, $n_{i,A} > n_{i,B}$. Their temperatures and volumes are the same. Pressure is different, as per they different amounts of gas. Now change the wall so that it is permeable. Gas from subsystem A will quickly diffuse into subsystem B, until equilibrium is achieved. The entropy of each subsystem is straightforward to determine:

$$S_{A,B}=n_{A,B}[c_v\ln T+ R\ln(V/n_{A,B})+k]$$

Using this information, it's easy to determine that $\Delta S_A<0$ and $\Delta S_B > 0$ (knowing that $n_{f,A}=n_{f,B}=n_T/2$). In other words, the entropy of subsystem A decreases and the entropy of subsystem B increases. It's also possible to the determine that the overall entropy change is an increase. However, what I'm trying to point out here is that the entropy of subsystem A decreases.

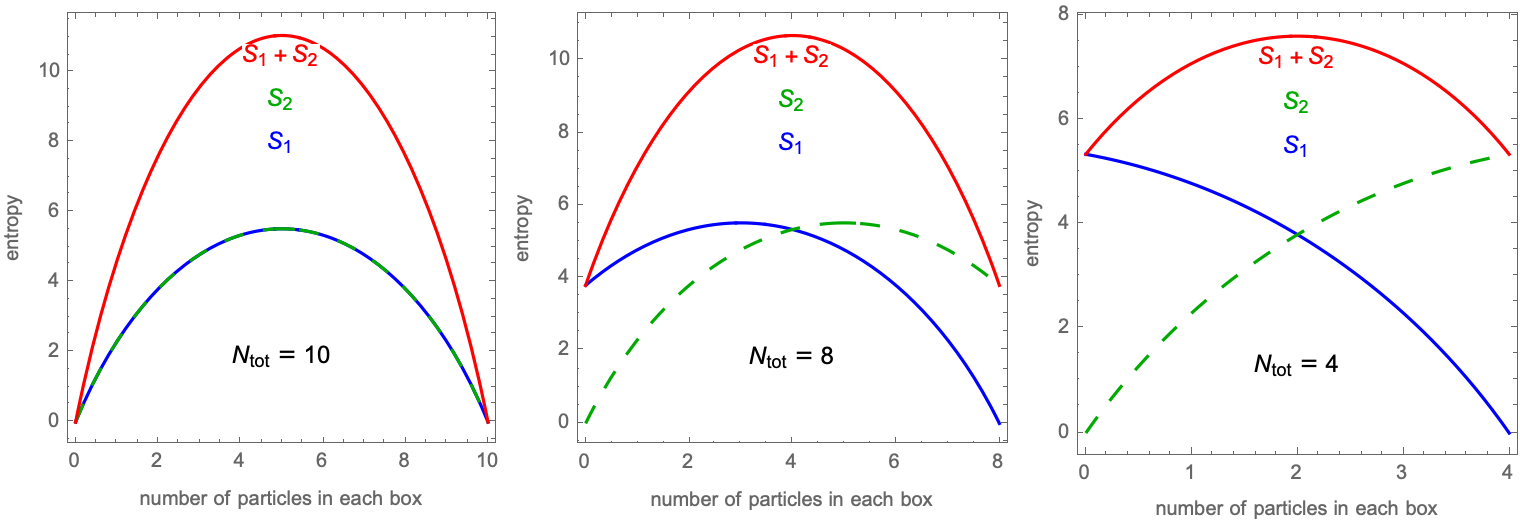
Now consider a similar system, but this time with only 10 particles we can place either in the right or in the left (let's say that left is A and right is B). Originally, we have 7 particles in A and 3 in B. We let the system evolve until there are 5 particles in each side: equilibrium is achieved. The Botzmann postulate for entropy is $S=k_B \log W$. We can caclulate the mutiplicity with the binomial coefficient. It's easy to see that, now, both entropies increase (and obviously the total entropy of the system increases) because the macrostate with 5 particles in one side has more microstates than either the one with 3 or 7 (in fact, both entropies increase the same amount).

So, what's going on? How come in the purely thermodynamical version, one entropy decreases, while in the statistical version, both increase?

Answer 1

Here is the correct calcualtion of entropy. Assume that each box is a lattice with $L$ sites, each with volume $v_0$ so that the volume of each box is $V=L v_0$. Put $N_1$ particles in box 1 and $N-N_1$ in box 2. The entropies are:

$$ S_1 = k_B \ln\frac{L!}{N_1! (L-N_1)!}, \quad S_1 = k_B \ln\frac{L!}{(N-N_1)! (L-N+N_1)!} $$

To compare the above result with the ideal gas calculation we must operate in the dilute limit $N/L\to 0$. In this limit particles truly become point masses as the ideal gas requires. Using the Stirling formula $\ln x! = x\ln x - x$ the entropy of compartment 1 becomes $$ \frac{S_1}{k_B N_1} = -\ln\frac{N_1}{L} - \frac{L-N_1}{N_1}\ln\frac{L-N_1}{L} $$ With $N_1\ll L$ we have $L-N_1 \approx L$ and the entropy becomes $$ \frac{S_1}{k_B N_1} = -\ln\frac{N_1}{L} = \ln\frac{L}{N_1} $$ Now write the volume of the system as $V = v_0 L$ where $v_0$ is the volume of a lattice site, also the volume of a molecule. The entropy becomes $$ \frac{S_1}{k_B N_1} = \ln\frac{V}{N_1} - \ln v_0 \Rightarrow \boxed{ \frac{S_1}{k_B N_1}= \ln\frac{V}{N_1} + \text{const} }. $$ The last result gives the ideal gas entropy as a function of volume at fixed temperature.

Conclusion To obtain the ideal gas limit in the combinatorial model we must take the system in the limit of infinite dilution.

The progression to the ideal gas state is demonstrated below with $L=10$, $N=10, 8, 6$: as $N$ decreases we approach the ideal-gas behavior where $S_1$ decreases and $S_2$ increases. For true ideal gas behavior we need to use a much larger $L$ relative to $N$.