Consider the following question.
The answer is $C$.
I am wondering why is there no centripetal force at point R acting towards the center to give it a centripetal acceleration such that the resultant acceleration vector is pointing down and to the left (or southwest) when both gravity and the centripetal force are accounted for?
The term centripetal force does not refer to a "real" force. It is just the name for the resultant force pointing towards the center of an object's circular path. It could be provided by many different sources, gravity or tension, for example.
Your statement "both gravity and the centripetal force are accounted for" suggests you think the centripetal force is an additional force in its own right. It is not. It is just the name for the resultant force that causes some kind of "circular motion".
So, to get to your question. Just think in terms of the forces acting on the mass at different positions. Adding these forces will give you the resultant force which will point in the direction of acceleration ($\vec F = m\vec a$).
At position $R$, the only force acting on the mass is its weight, directed vertically downwards, since there is no tension in the rope. Therefore the acceleration at this point is downwards.
At position $Q$, there is the same force due to gravity acting downwards but there is also tension now. The tension is acting upwards, and is larger than the force due to gravity downwards. How do we know it is larger? Well if it wasn't the resultant force would be downwards and the string would break. Therefore the resultant force is upwards and hence the acceleration is upwards at this point.
Hopefully this clears up your confusion. To summarise: there is no centripetal force or acceleration at position $R$. The acceleration is downwards, not inwards.
Technically "centripetal force" is just a force component just like how "horizontal force" and "vertical force" are force components. So really when you say
...when both gravity and the centripetal force are accounted for?
you should instead say
...when both gravity and the tension force are accounted for?
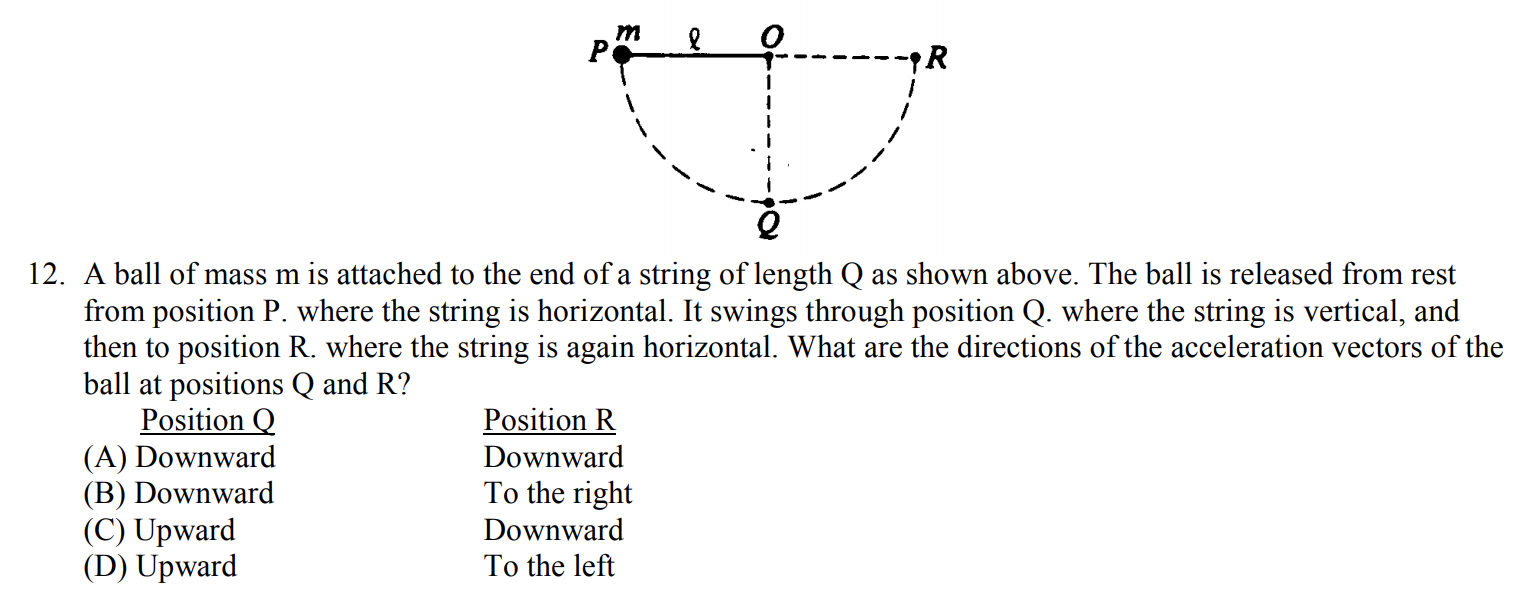
At point $R$ the only force that can be centripetal is the tension force, therefore $T=mv^2/r$, but $v=0$, therefore, $T=0$. So, the simple answer here is that the mass is at rest at point $R$, so there is no tension force, and the only force acting on the mass is gravity. However, continue reading you want a more quantitative approach.
We can take both into account both forces at any point along the circle. We know the force of gravity is a constant force $\mathbf F_g=-mg\,\hat y$, and we have a tension force $\mathbf T=-T\,\hat r$ where $T$ is a varying force magnitude and $\hat r$ is a unit vector that points away from the circle.
Considering how the centripetal force component is always equal to $-mv^2/r\,\hat r$ in circular motion, we know that $$\frac{mv^2}{r}=T-mg\sin\theta$$ where $\theta$ is measured counter-clockwise from point $P$. This lets us determine $T$ in terms of $\theta$ and $v$, and hence the net force at each point along the circle.
$$T=\frac{mv^2}{r}+mg\sin\theta$$
For example, at point $P$ we have $\theta = 0$ and so $$\mathbf F_P=\mathbf F_g+\mathbf T=\frac{mv^2}{r}\hat x-mg\,\hat y$$
At point $Q$ we have $\theta = \pi/2$ $$\mathbf F_Q=\mathbf F_g+\mathbf T=\frac{mv^2}{r}\,\hat y$$
At point $R$ we have $\theta=\pi$ $$\mathbf F_R=\mathbf F_g+\mathbf T=-\frac{mv^2}{r}\hat x-mg\,\hat y$$
Since the tension force does no work on the mass, and because gravity is conservative, energy is conserved here. Therefore, we can easily determine the velocity at any point on the circle given that the mass starts at rest at point $P$
$$\frac12mv^2=mgr-mgr(1-\sin\theta)=mgr\sin\theta$$ $$v^2=2gr\sin\theta$$
This lets us find the net force at each point
$$\mathbf F_P=-mg\,\hat y$$
$$\mathbf F_Q=2mg\,\hat y$$
$$\mathbf F_R=-mg\,\hat y$$
Notice how because the mass is at rest at points $P$ and $R$ the tension force is $0$, and so the net acceleration at these points is downwards.
