EDIT: Added an animation at the end that might be easier to follow.
First let's consider a simpler model of a perfect spherical planet rotating about the vertical axis with North at the top.
Diagram 1:

The only real force present is the force of gravity (a) acting inwards. This force is broken down into two components, (g) and (c). (g) is the centripetal force which is just a component of the gravitational force and there is no balancing force for this component, so the force accelerates the particle inwards and this keeps the particle moving in a circle as the planet rotates. This leaves component force (c) which is dissected into two more components (d) and (e) in the diagram below.
Diagram 2:
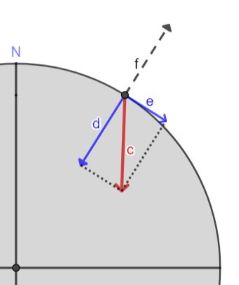
(d) is the inward weight force and (f) is a balancing reaction force. (e) is a sideways force and if there is insufficient friction, this unbalanced force tends to accelerate everything on the surface towards the equator. If the Earth was a sphere, this sideways force would eventually deform the Earth and any surface liquid tends to pile up at the equator. (The molten rock below the surface ensures the Earth is not a perfectly rigid object)
Eventually an equilibrium point is reached where the sphere becomes oblate as in the diagram below. The oblateness of the Earth is greatly exaggerated (as is the centripetal/centrifugal force) for clarity.
Diagram 3:

Again, the only real force is the force of gravity (a) acting towards the centre. (c) is the inward component normal to the surface that is felt as weight and (f) is the reaction force to (c). Component force (g) is not balanced and provides the inward centripetal acceleration. Since g is fully accounted for as providing the centripetal force, the only forces left are component (c) and reaction force (f). Since these are parallel and both normal to the surface, there is no longer any sideways force on surface particles. You can view the centripetal force simply as a component of the inward gravitational force. You could interpret the centrifugal force as the resultant of forces (a) and (f), but this is difficult to justify, as force (f) is cancelled by force (c). It also worth noting that the reaction force (f) is not really a 'tilted' normal. It is perpendicular or normal to the surface. It is only tilted in the sense that is not parallel to the inward acting gravity force (a). It is also worth noting that something like the Earth that has had millions of years to find equilibrium should be almost the perfect oblate shape required for equilibrium.
===========================================================
Food for thought: If you build a tall tower using a plumb bob to ensure it is vertical, will the tower be parallel to (f) or (a)?
P.S. While I narrated this from the point of view of starting with a sphere for simplicity, it should be obvious from the points above that if the the Earth starts as an over oblate smarty shaped planetoid, then the sideways forces will tend to contract the smarty to something closer to spherical at the equilibrium point.
Edit: Decided to add this final free body diagram to illustrate the last point. It represents a zoomed in portion of an over oblate planetoid.
Diagram 4:
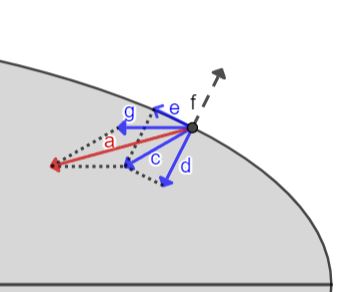
(a) is the centre pointing gravitational force. (g) is the component of (a) that provides the centripetal force. That leaves component(c) which is subdivided into two more components, (d) which is the downward normal force, which is balanced by the reaction normal force (f) and sideways force (e) which is not balanced and drives surface material towards the pole until the equilibrium oblate shape is achieved.
Just a doubt. In the first diagram why don't you put a component of
the normal force from the ground projected in the direction opposite
to the centripetal force? – Mark_Bell
The force opposite to the centripetal force (in the non rotating reference frame) is called the centrifugal force. This is a 'fictitious' force and does not appear in an inertial reference frame. If there was a counter force balancing the centripetal force, there would be no net force to keep the particle moving in a circular path. In the reference frame of a non inertial observer rotating with the Earth, the centrifugal force is real.

In the above animation, the initial primary forces are the gravitational force (a) and the centrifugal force (b) as seen by an observer on the surface rotating with the planet. Force a is resolved to component (d) that opposes the centrifugal force and component (c). The centripetal force (d) and the centrifugal force (b) cancel each other and there is no motion parallel to the equatorial plane. This leaves component (c) that is resolved to components (f) normal to the surface and (e) tangential to the surface. There is a reaction force (g) also normal to the surface and this cancels the force (f). The only force left is component (e) tangential to the surface that acts towards the equator. This could be opposed by sufficient friction force, but in the absence of friction (or very little friction such as surface liquid) the representative particle is propelled towards the equator. In the inertial reference frame, the fictitious centrifugal force vanishes and there is no force to oppose the centrifugal force and the particle follows a circular path as seen from the North pole.
The interactive animation is here. The oblateness of the spheroid can be altered with the R slider to find the equilibrium point (around R=5) and the over oblate case can be investigated, where the residual force is towards the pole. The animation is controlled by the video play button at the bottom left or alternatively gone through step by step or in slow motion using the T slider.
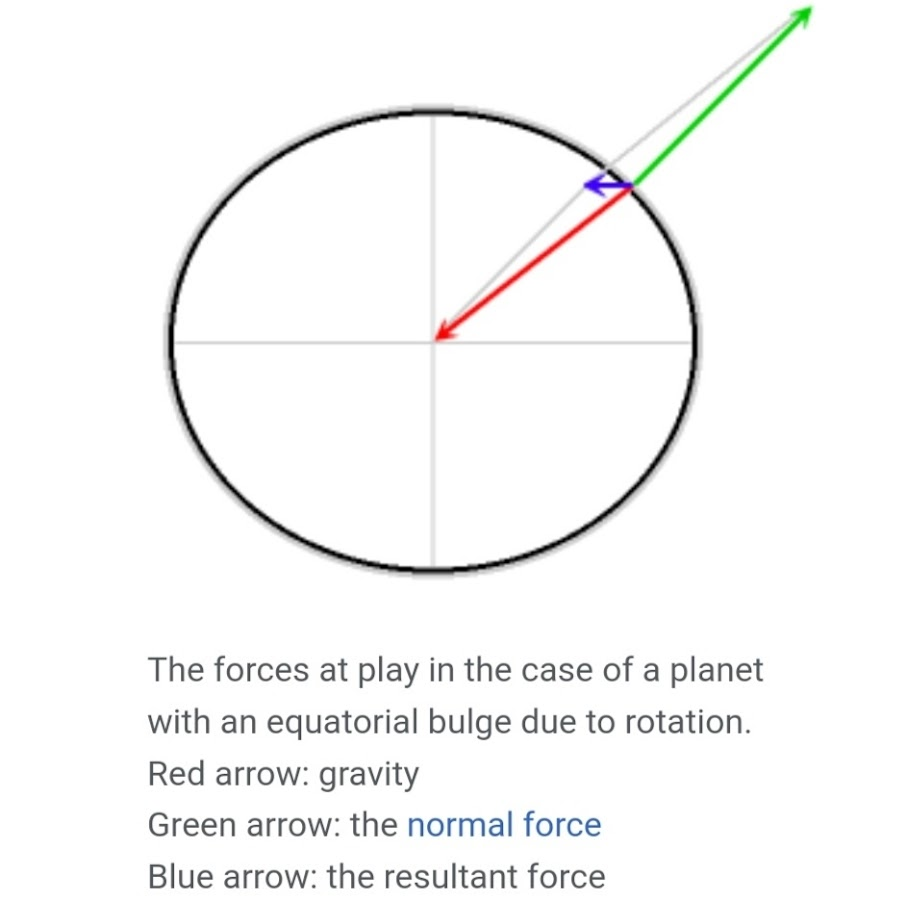
 This is what I see, what is the reason behind this?
This is what I see, what is the reason behind this?





