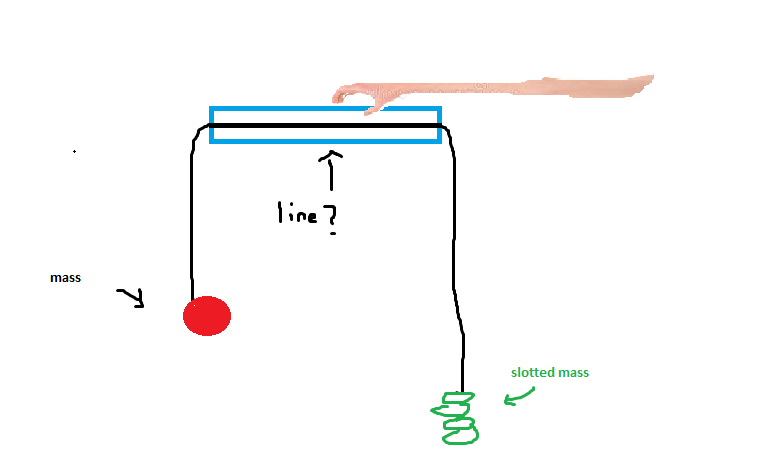
Is the slotted mass responsible for the centripetal force in vertical circular motion?

Question

Is the slotted mass responsible for the centripetal force in a vertical circular motion in this particular experiment?

I want to do the following experiment, where I hand spin a mass (red) that is attached to the same string with the slotted mass at the other end. I spin the mass by grabbing the hollow tube (blue).

I know the fact that in horizontal circular motion, the weight of the slotted mass would provide the centripetal force and hence the tension force. So, $Fc = m * g$ where m is the mass of the slotted mass.

But what if now I hand spin the mass in vertical motion? Would the centripetal force still provided by the weight of the slotted mass? Why or why not?

What impacts does the straight line in the hollow tube have on the centripetal force if it was spun in vertical circular motion?

Answer 1

Would the centripetal force still provided by the weight of the mass? Why or why not?

When you spin it vertically, you would most probably spin it with a constant angular velocity (try it out), which means that that the centripetal acceleration required at any point will be the same. However, in this case, the radial component of force due to gravity keeps on changing which means the tension will keep changing so as their resultant is equal to the centripetal force. So that means the slotted mass will oscillate. Also, we are assuming ideality in the sense that the tension is uniform throughout the string, which is definitely not the case.

What impacts does the straight line in the hollow tube have on the centripetal force if it was spun in vertical circular motion?

The centripetal force only depends on the angular velocity and has nothing to do with the length of that tube. If you keep all the other parameters (radius of the circular motion, angular velocity and the mass of the slotted weight) the same, then the only difference due to changing the length of the tube will be that, you will have to apply a larger torque (see the figure below) to keep the tube in its position. This because although the centripetal force is the same, stil the length of the moment arm has increased which means a higher torque.

Answer 2

I think it would be impossible to swing it with constant angular velocity without the radius changing.

If the angular velocity and radius are both constant, then the centripetal acceleration will be constant. This acceleration is caused by the resultant of the weight of the mass and the tension in the string. At the top of the circle, these act together, so the tension is really small, whereas at the bottom of the circle the tension is really big. If the tension in the string keeps changing, then the slotted masses will keep moving up and down, but if the string has a constant length, that will make the radius of the 'circle' get bigger and smaller as it goes round.

Much easier to analyse (but probably no easier to actually do) is to keep it going in a circle of constant radius, but with varying speed. If the radius and therefore the tension stay constant, then there will be a big centripetal acceleration at the top, so the mass will need to travel really fast. At the bottom the acceleration will need to be small so the velocity will need to be small. The problem is that this goes against energy conservation, that it's likely to gain kinetic energy as it goes down and lose kinetic energy as it goes up, so you have to find a way of getting energy in as it goes up, but removing it again as it comes back down!

This is very odd. Has anyone actually had a go at doing this, and is it at all possible to keep the radius of the circle constant?