0
$\begingroup$

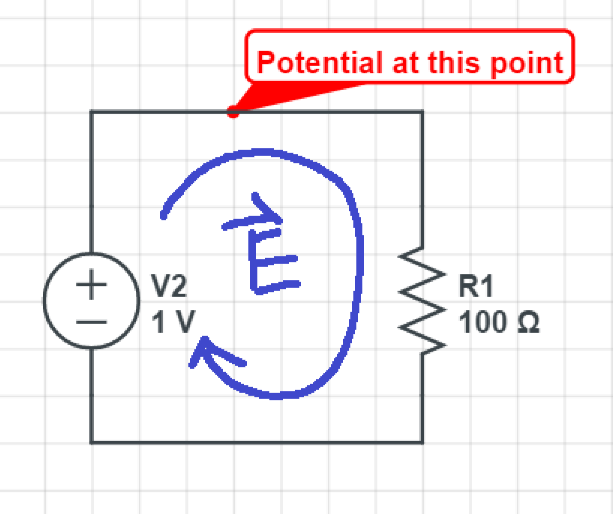
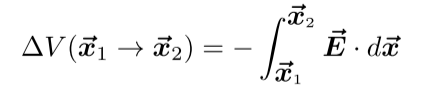Voltage is defined as: enter image description here

Given the circuit: enter image description here

Why would potential not drop, from the point differentially away from the positive terminal on the battery, to the point I have labeled. I understand that charges lose energy in resistors due to inter lattice collisions, where the acceleration generated due to thermal motion and voltage bias is translated from kinetic energy to heat energy. Before the resistor the resistance is neglible so the drift velocity will be high, and collsions will be minimal. Even so as the charges move from the positve terminal the Electric field will be strong and we will be losing the potential energy as we travel in the direction of the field. With this so why dosent potential drop as we move in the direction of the field? Is the energy associated with the charge essentially translated from potential to kinetic energy, where very little of this kinetic energy is translated to heat energy, and we assume the total potential is associated with the total energy the charge has at this point (KE + PE)?

Please address the question above. Further discussions will be encouraged though!

I am really looking for an answer to my question. It would be much appreciated if we address the core question I asking instead of side discussions!

Added picture: enter image description here

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ Do you understand that the field is $0$ inside a perfect conductor? $\endgroup$
    – BioPhysicist
    Commented Mar 3, 2020 at 0:13
  • $\begingroup$ The field is an external entity, it has nothing to do with the conductor at all actually... It is generated due to into charge forces (columb forces) of charges accumulated at the anode, essentially there will be a lack of electrons on the battery positive terminal and an abundance of electrons on the cathode side. I am strictly thinking of convential current but there will be an E field inside the conductor. $\endgroup$
    – Grant
    Commented Mar 3, 2020 at 0:24
  • $\begingroup$ Not quite. The conductor consists of mobile charges. Each of these charges also can contribute to a field. So the field inside the wire is the sum of field from the battery, plus the sum of the fields from these mobile charges. You can't assume the field would be the same as it is in their absence. $\endgroup$
    – BowlOfRed
    Commented Mar 3, 2020 at 0:37
  • $\begingroup$ Grant, I am not sure what you mean by "it has nothing to do with the conductor". A property of perfect conductors indeed is that the field is $0$ inside of them. Of course this is an idealization, but it is a pretty good one. As @BowlOfRed points out in their answer the field is still negligible at steady state. $\endgroup$
    – BioPhysicist
    Commented Mar 3, 2020 at 0:39
  • 4
    $\begingroup$ Does this answer your question? Electric Potential drop in wires $\endgroup$
    – BioPhysicist
    Commented Mar 3, 2020 at 0:40

2 Answers 2

Reset to default
1
$\begingroup$

Even so as the charges move from the positve terminal the Electric field will be strong and we will be losing the potential energy as we travel in the direction of the field.

This is true only for a short period immediately after the circuit is closed. The field will accelerate the charges down toward the resistor. But when the charges reach the resistor, they will be slowed down.

This means that some charges will pile up at the boundary between the wire and the resistor. These charges will create an electric field that opposes the field of the battery in that section of the wire.

Eventually, their field will be strong enough that exactly as many charges arrive at the resistor as leave the resistor. The circuit will be in steady-state, and the net field inside the wire will be negligible.

(If resistance were zero, the field would also be zero. For a small resistance, the field is small).

Improve this answer
$\endgroup$
7
  • $\begingroup$ Okay, so essentially what I am seeing is that charges will lose potential as it is translated to kinetic energy, but when they reach the resistor they essentially hit a barrier or bump in which they are slowed down. Doing so we get an accumulation of charges. The kinetic energy pushes aganist the resistive force of the accumulated E field so that in general the potential at this point is the same as it was at the anode of the battery? $\endgroup$
    – Grant
    Commented Mar 3, 2020 at 0:45
  • $\begingroup$ Agree on the first 2 sentences. In the third, I'm not sure the KE "pushes" against anything. The KE of the charges is established in the circuit (and constant throughout), and the field inside the wire is negligible, so the charges move in the wire with no change in PE or KE. $\endgroup$
    – BowlOfRed
    Commented Mar 3, 2020 at 0:50
  • $\begingroup$ Okay let me elaborate, the problem I have there is that the electric field due to the anode of the battery is smaller at the point where the resistor is, so the accumulation of charges strictly to the combative electric field of the anode versus top end of resistor would lead to a smaller potential (smaller accumulation of charges) at the top end of the resistor. The charges moving with the drift velocity (kinetic energy) has momentum associated with it which push aganist the electric field of the accumulated charges at the resistor, therefore this allows more charges to accumulate, restoring $\endgroup$
    – Grant
    Commented Mar 3, 2020 at 0:52
  • $\begingroup$ the potential at the top end of the battery due to the accumulation of charges. Also why do you say the field inside the wire is negligible? There is a field generated by the battery, and since the non induced E force is a conservative field there will be an electric field experienced inside the conductor regardless of direction. $\endgroup$
    – Grant
    Commented Mar 3, 2020 at 0:53
  • $\begingroup$ The net field inside is negligible because the charges in the wire react to the (external field) by moving in such a way that an opposing field is created. The battery's field is still contributing, but a cancelling field is also present. $\endgroup$
    – BowlOfRed
    Commented Mar 3, 2020 at 1:00
0
$\begingroup$

When we say charge in a wire, there are over a gazillion number of charges in that wire at any point of time. So, when we say a current I, we imagine it as sort of a quantity existing everywhere, just as the electric field.

The charge has kinetic energy because of the electric force acting on it. The potential energy of the battery/cell supplies energy to a chemical process establishing potential difference across the ends of the cell.

The said charge does lose kinetic energy as it interacts with the atoms. But the charges don't matter as much, since the electric field is defined between the opposite ends of the cell, as I have just explained in the previous paragraph.

One interesting observation here is that current travels faster than the electrons themselves, since it is the transmission of energy that takes place so quickly and that operates a light bulb, or fan, or etc.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Currently does not travel faster than the electrons themselves. Current is "drifting" against a linear drag force. The charges themselves have thermal motion since they are more or less in thermal eqilibrium with the conductor, and they bounce aroudn randomly VERY QUICKLY, the slow drift is due to the drift being biased in the direction of lesser potential. This is not an answer to my question.. Furthermore yes there are a bunch of charges but they are in equilibrium when the potential is not supplied aka they have no potential energy. $\endgroup$
    – Grant
    Commented Mar 3, 2020 at 0:22

Not the answer you're looking for? Browse other questions tagged or ask your own question.