1
$\begingroup$

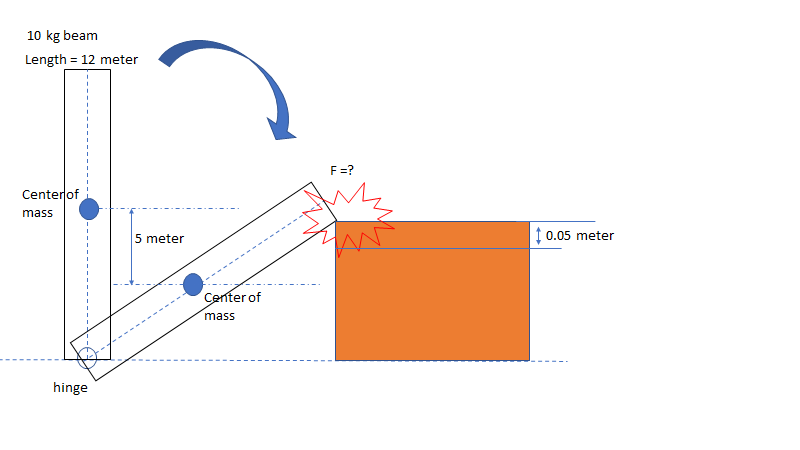
A beam of 10 Kg and length 12 meter starts to fall from initial vertical position with one end hinged.

When Beam's center of mass is dropped 5 meter from earlier position, Beam hits an object with its far end.

The object compresses by 0.05 meter.

To calculate impact force, I have taken full mass into account. So Impact Force X displacement 0.05 meter = mass 10 Kg X g 9.81 X h 5 meter Impact Force = 9810 N

I have two questions about my method

  1. If Beam weight is 10 Kg on weighing scale; Does it mean mass m is 10 Kg or weight mg is 10 kg? if mg is 10 then force will be only 981 N. ( sorry for the dumb question)

  2. As we see only head of beam makes an impact with the object. So should I consider full mass for impact force calculation? Or a factor of it?

  3. Will it make any difference if instead of beam, a flat disk of same height and weight is falling? All condition remaining same.

Please help me. Basically I have to make sure that the object being hit is safe.

enter image description here

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ Are you familiar with the work-energy theorem? It will give you the average impact force. And what do you mean by "safe"? $\endgroup$
    – Bob D
    Commented Feb 21, 2020 at 20:43
  • $\begingroup$ well safe means I have to design the part in such a way that it is not broken when the beam trips over it. Actually it is a disc that is falling , but for simplicity I have taken a beam for calculations. $\endgroup$
    – Sheldon
    Commented Feb 22, 2020 at 9:39
  • $\begingroup$ It is mechanical stress that causes failure not force alone. That makes the geometry at the impact site critical and also makes this an engineering problem not physics concepts and therefore off topic $\endgroup$
    – Bob D
    Commented Feb 22, 2020 at 12:09

1 Answer 1

Reset to default
0
$\begingroup$

1st step is to calculate the kinetic energy at the moment of impact from the difference in potential energy.

The problem then is to find the impulse needed to stop the beam, considering the hinge will produce a reaction impulse also. In regards to your 2nd question, only a fraction of the mass participates in the contact. This is because the point of contact is off-center. The calculation is $m_{\rm eff} = 1 / \left( \tfrac{1}{m} + \tfrac{d^2}{I}\right)$ where $d$ is the distance to the center of mass, and $I$ the mass moment of inertia of the beam.

Finally somehow you need to translate the impulse into a force, considering the elastic properties of the beam and the time of impact. this last part is really hard to estimate since there are so many factors that go into this.

Improve this answer
$\endgroup$
7
  • $\begingroup$ One question. When the beam impacts the object, isn't the kinetic energy of the beam equal to the loss of potential energy of the center of mass of the beam? It would seem that all of the loss of the beams kinetic energy is due to the deformation of the object, which for simplicity we can assume to be completely inelastic. Where else could the kinetic energy go? $\endgroup$
    – Bob D
    Commented Feb 21, 2020 at 21:45
  • $\begingroup$ Don't forget the rotational kinetic energy as well as the one from the center of mass. Then all the energy goes into the deformation, but also into deforming the beam which wasn't originally considered. $\endgroup$
    – John Alexiou
    Commented Feb 22, 2020 at 2:51
  • $\begingroup$ Right you are. Thanks $\endgroup$
    – Bob D
    Commented Feb 22, 2020 at 4:01
  • $\begingroup$ Also deformation at the hinge is not considered. Clearly the beam, hinge and hinge supporting structure are all considered rigid bodies. Regardless of the type of kinetic energy involved (rotational and/or translational) it is all absorbed by the deformable object. And the only source of the energy is $\Delta PE$. So why isn’t this simply $F_{ave}d=\Delta PE$ where $F_{ave}$ is the average impact force? $\endgroup$
    – Bob D
    Commented Feb 22, 2020 at 7:26
  • $\begingroup$ Thank you ja72 & Bob for your answers. i will calculate m_eff based on your formula. I think it will be very helpful. Regarding the deformation of beam: I am currently ignoring it . It will work as a safety factor. regarding Bob's Faved=ΔPE : I have done that exactly,if you see my question, however I feel it is not correct as beam is hinged at one point, and not falling freely. So I think 100% energy will not be transferred to the object being impacted. $\endgroup$
    – Sheldon
    Commented Feb 22, 2020 at 9:48

Not the answer you're looking for? Browse other questions tagged or ask your own question.