Relationship between wavelength and amplitude

Question

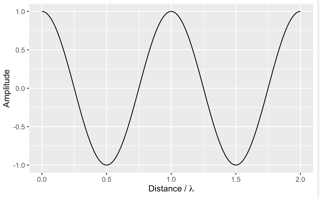
So, a wavelength is the distance over which the wave shape repeats. Now that means it is the length of a sine wave from 0 to 2π.

Now if we take an example of pendulum (which we can represent In sine wave) from mean position (i.e 0 in sine graph) and it goes after time T it comes back to mean position again (2π). So the distance the Bob travelled is 4A. According to this answer it is correct :- Oscillations concept about displacement

But there is no relation as:- λ= 4A
So why λ is not 4A, does it have to do anything with the above logic(in 2nd para) ?

End.

(Extra :- although there is a relation :-

Distance between a node and an antinode =λ/4.)

Answer 1

The confusion lies in the fact that you are mixing up the sinusoidal behaviour of the pendulum in time and the physical distance covered.

It’s simpler explain in terms of a simple harmonic oscillator. Here the displacement $x$ as a function if time is given by $$x(t)=A\sin\left(\omega t\right)$$ As you can see, the displacement is sinusoidal in time. For this wave, the “wavelength” will be the time interval after which the wave returns to the original position. Which is nothing but the time period $T$. So when we talk about the time period of the oscillations we are essentially talking about the “wavelength” of the wave.

Answer 2

A plane wave is described by $$ y(t) = A_0 \cos{(k x - w t)} $$

It has an amplitude $A_0$ in y-direction, a wavelength $\lambda = 2\pi/k$ in x-direction, and a frequency $f = w/(2\pi)$. In contrast, the motion of a pendulum is described as $$ \theta = \theta_{max} \sin{(wt)} $$ where we assumed a "small" angle $\theta_{max}$. By plugging this into the position relation, we obtain \begin{align} x(t) &= \ell \sin{\theta} = \ell \sin{(\theta_{max} \sin{(wt)})} \\ &\approx \ell \theta_{max} \sin{(wt)} \end{align}

Thus, it makes sense to define an amplitude of the oscillation $A_0 = \ell \theta_{max}$ and a frequency $f = w/(2\pi) = \sqrt{g/l}/(2\pi)$, but it does not make sense to speak of a wavelength $\lambda$. If somebody uses the term wavelength in the context of a pendulum, they probably meant to say amplitude.

Answer 3

A pendulum is a harmonic oscillator if the amplitude is restricted to a small angle. It is possible to combine the harmonic motion with wave propagation as follows:

We can think of a sine wave along the $x$-axis as being composed by one pendulum for each $x$ point, oscillating in the $y$-direction with the same frequency and amplitude, but with a gradient of phase.

The phase gradient is such that if an observer moves along the $x$-axis with a specific speed (called wave velocity), he will see a static periodic pattern.

The wave length is the minimum distance between $x$ points which repeat the pattern for that observer.