0
$\begingroup$

enter image description here

Source: Principles of Nano-Optics, for Lukas Novotny and Bert Hecht.

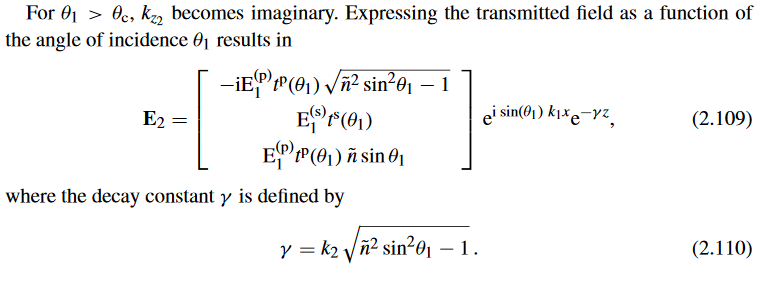
The equations above represent the electric field in the second medium when a light hit a surface and the condition of TIR (total internal reflection) is satisfied. Actually this is what called Evanescent field. The point is if I want to calculate the gradient force on a particle in this field, F(gradient)=0.5a*gradient|E|^2, where "a" is the Polarizability and "E" is the electric field. How does the gradient force hold in y- direction? If I make the derivative of the field in y direction it will vanish according to the equation above because there is no variable y that exist in the equation in y axis, on another hand how I read in some articles that they say the gradient force is exist in y direction but with no explanation or derivation!

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

There is no $y$ dependence in your expression for the electric field, and hence there is no gradient force along the $y$-direction you are asking in this case. The expression of the electric field you gave is for a plane wave whose wave vector is in the xz plane. Any plane wave dose not change along the perpendicular direction of its propagation direction. This is kind of an ideal case because any beam of electric field has finite span in space, otherwise it will have infinite energy! If your electric field has finite span, for example a cylindrical beam with a Gaussian envelope, then your electric field has $y$ dependence and you get a gradient force along $y$ direction. I did not find the article you read but I guess they assumed finite span in space.

Improve this answer
$\endgroup$
8
  • $\begingroup$ Thank you so much for your answer, actually I didn't get your point well about finite span in the space. I'm attaching here the article: ncbi.nlm.nih.gov/pubmed/30417030 this is one of the articles that they say the gradient force in y direction.. $\endgroup$
    – stdscience
    Commented Jan 9, 2020 at 10:06
  • $\begingroup$ In the figure 1 of the article you attached, I found a nano waveguide and a nano particle floating on it trapped by the evanescent field. I have also found that the waveguide is along the x-direction and has finite height along the z-direction. The waveguide has finite span (size) along the y-direction. The evanescent field is then uniform in its amplitude (or intensity) along the x-direction while the field is decayed along the z-direction. Along the y-direction, the evanescent field has a peak at the middle of the waveguide and is decaying radially. $\endgroup$
    – Above Threshold
    Commented Jan 9, 2020 at 19:50
  • $\begingroup$ Thus the gradient of the intensity of the evanescent field has both the y and z compoents in this case. The equation you provided, however, is for a plane wave and it is not applicable in the case that the article deals with. $\endgroup$
    – Above Threshold
    Commented Jan 9, 2020 at 19:52
  • $\begingroup$ Then I have a new problem now .X. ! why should be intensity decaying along y! If the wave plane inside the waveguide is traveling along x direction, so the evanescent field propagates along x-axis and in z-axis we have decaying of the intensity and along y axis we should have a constant intensity.. I can't under stand why we have intensity decay in y direction! and with the equation above I can know the intensity in each direction.. Please clarify it to me. $\endgroup$
    – stdscience
    Commented Jan 9, 2020 at 21:26
  • $\begingroup$ Then let me ask you a question. Why do you think (not by the equation you gave) the evanescent field decay only along the z-direction? The expression for the evanescent field you gave here is in the case of a plane wave with infinite span along the y-direction. That is why you do not find any y dependence in the equation. In the case of the article you try to understand, however, they used a waveguide, which has evanescent fields along the z direction and the y direction at the same time. $\endgroup$
    – Above Threshold
    Commented Jan 10, 2020 at 3:21

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.