3
$\begingroup$

If i see a reflection on a horizontal surface and i try to cancel it out using my polarized sunglasses, then it cancels out much of that reflection. But when i put a small mirror inside that reflection on the same surface and try to cancel out the reflection from that surface using polarized sunglasses, then it cancels out much of that reflection, but it do NOT cancel out the light that is reflected from the surface of the mirror. Why is that?

Please refer the image linked below, if you can not understand what i am describing

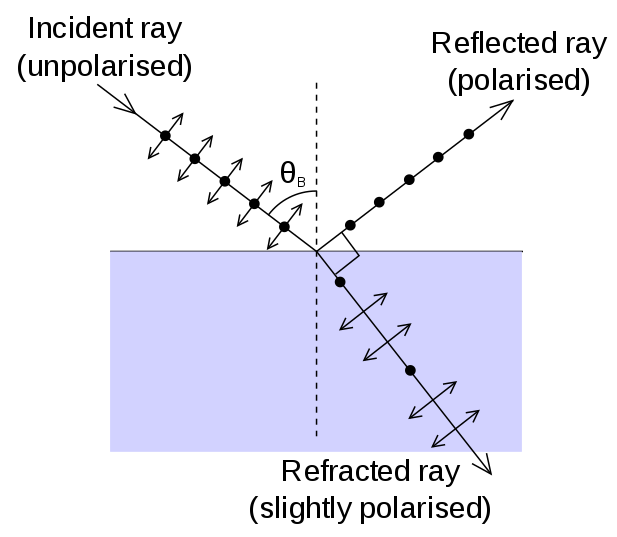
enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
3
$\begingroup$

Polarization results when unpolarized light impinges on a transparent medium at (or near) Brewster's angle : enter image description here
A mirror (electrically conducting surface) reflects both polarizations equally.

Your "horizontal surface" may be something like smooth "shiny" "black" plastic, but if it is "black" then why does it reflect at all? Because it is a dielectric material with an index of refraction different from air that has light absorbing particles in it. On the scale of a few light wavelengths it acts like a transparent medium.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Brewster’s angle explains about polarizing the unpolarized light at ONLY certain angle or just near that angle. But you can cancel out reflection/glare with polarized sunglasses at many different angles. So i don’t think polarized sunglasses works completely works on Brewster’s angle. After your answer, i have attached an image with my question; so you can refer it to understand what exactly i want to know. I am sorry but your answer do not explains many of my other questions. $\endgroup$
    – user248881
    Commented Dec 28, 2019 at 1:29
  • $\begingroup$ @user248881 These lectures cover normal and oblique reflections from perfect conductors. There might be a simple explanation (without the math) of why polarizations are preserved, but I don't have one now. $\endgroup$
    – Keith McClary
    Commented Dec 28, 2019 at 2:33
  • $\begingroup$ Do all the reflection/glare that we can see in this (i.sstatic.net/jV13f.jpg) image represents plane polarized light which is caused of Brewster’s angle? $\endgroup$
    – user248881
    Commented Dec 29, 2019 at 1:23
  • $\begingroup$ "When the reflecting surface is absorbing, reflectivity at parallel polarization (p) goes through a non-zero minimum at the so-called pseudo-Brewster's angle.", so it is not 100%. Brewster's angle for glass is approximately 56° (measured from the normal, vertical in this case). $\endgroup$
    – Keith McClary
    Commented Dec 29, 2019 at 2:18
2
$\begingroup$

Brewster's angle is only a special case and not as crucial as people might think in this case. To keep things short:

Mirror: Conventional mirrors have a metallic surface (e.g. aluminum or silver) where the mobile surface charge carriers cause a reflection of the light. Polarization usually does not matter enough here to cause a significant polarization of the reflected light. Hence, it can only be partially blocked by a polarization filter. You can look at Graphs for s- and p-polarization on this page (click on "Graphs" for plots) to verify that s- and p-pol don't make too much of a difference here.

Floor: Dielectric (non-conducting) materials also reflect a part of the light according to the Fresnel equations (This page actually shows a figure related to your question). For large angles of incidence (let's say roughly larger than $40°$) the reflection properties are usually completely different for s- and p-pol. P-pol light is then reflected poorly while a part of the s-pol light still gets reflected. Brewster's angle is an extreme case of this ($0\%$ reflection of p-pol light), but generally the p-pol reflection is much weaker for steep angles. This causes a strong polarization of the reflection.

Improve this answer
$\endgroup$
4
  • $\begingroup$ When the surface is rough we get diffuse reflection, and when the surface is smooth we get specular reflection. Now, in case of diffuse reflection, we don’t get polarized light, while in case of specular reflection, we get polarized light. From this we can conclude that as the surface gets smoother, there are more chances of polarization to occur. Now my question is if mirror has very smooth surface then how it can not polarize the unpolarized light? $\endgroup$
    – user248881
    Commented Jan 4, 2020 at 4:01
  • 1
    $\begingroup$ @user248881 metallic mirror has a different mechanism of reflection from that of a dielectric surface. $\endgroup$
    – Ruslan
    Commented Jan 5, 2020 at 9:50
  • $\begingroup$ @Ruslan Thank you very much! Can you please explain me the difference between reflection of light from the surface of a metallic mirror (silver) and a dielectric surface (glazed ceramic tiles)? $\endgroup$
    – user248881
    Commented Jan 5, 2020 at 11:13
  • $\begingroup$ @user248881 the difference is in interface conditions for EM fields. $\endgroup$
    – Ruslan
    Commented Jan 5, 2020 at 12:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.