Electrical generators: power balance

Question

Usually power generating synchronous generators are rated at around 85-90% efficiency. Curiosity struck me as of how a generator with field coils can ever go to such an high efficiency.

My thought process is as follows.

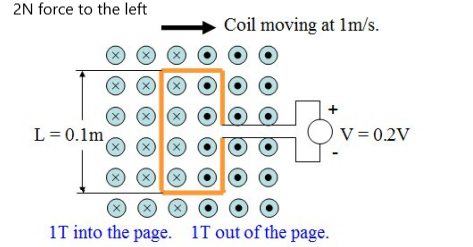
The power balance for the considered generator with permanent magnets are first considered 2N* 1 ms-1 and 2W generated power match in this case

Now imagine the 1T permanent magnet has now been replaced with electro magnet. In order to get one Tesla magnetic flux density according to the Ampere's law it takes ~500000 A on a 0.1 m conductor.

REF : https://www.khanacademy.org/science/physics/magnetic-forces-and-magnetic-fields/magnetic-field-current-carrying-wire/v/magnetism-6-magnetic-field-due-to-current

Obviously this doesn't add up and something is incorrect in my thinking. Appreciate it if someone can point what is wrongs in the above and show me how to calculate the correct excitation current on the field coils.

Answer 1

I'm not sure I understand the nature of your question. Generator efficiency depends on shaft support bearing losses, ohmic losses in all the windings, and flux leakage in the gaps between the pole pieces in the stator and the armature. Efficiency is maximized when these are all minimized.

Answer 2

I found the answer to my question here

In summary, the field winding does not have to provide all the magnetic flux due to the fact that the current-carrying armature, in turn, creates a magnetic field.

So the net magnetic flux becomes $B_{net} = B_{rotor} + B_{stator}$

In an imaginary totally resistive stator, the power balances out without any rotor influx.