0
$\begingroup$

SHM

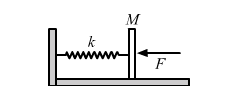
In the attached picture, is the spring mass system in equilibrium with a constant force $F$?

My question supposes that the system is slightly displaced from equilibrium (let's say to the left). Is the right side amplitude equal to the left side amplitude even when the force F direction is towards left?

I think “No”, as while going to the left side from the mean to the extreme, net force will be ($F-Kx$), and while going to the right from the mean to the extreme, the net force will be $(F+Kx)$. As the forces are different, the amplitudes should be different, too (because accelerations are different).

Am I right ?

Will it still be called SHM even if right-hand amplitude ≠ left-hand amplitude?

Improve this question
$\endgroup$

2 Answers 2

Reset to default
1
$\begingroup$

In the equilibrium position the spring must be compressed by a distance $a$, say. Then, with the usual notation, writing $F_0$ for the constant force,$$F_0=ka.$$ Displace the mass by $x$ to the left from its equilibrium position and the net force to the right on the mass will be $$k(x+a)-F_0=kx.$$ $$\text{So}\ \ \ \ \ \ \ \ \ \ \ \ \ \ \ m\ddot x=-k x$$ So we have ordinary symmetrical shm about the equilibrium position because we can check that the differential equation is satisfied by $x=A\ \cos \omega t\ \ \ \ \text{or}\ \ \ \ \ x=A\ \sin \omega t \ \ \text{or any linear combination thereof}$.

This is exactly the same situation as a mass hanging from a spring (in which case $F_0=mg$).

Improve this answer
$\endgroup$
4
  • $\begingroup$ But when the the block is moving towards right (from mean {a} to extreme) then net force towards left at any pos. x will be K(a+x) +𝐹(net restoring) while moving towards left(from mean to extreme ) will be 𝑘(𝑥+𝑎)−𝐹(net restoring) . Now as the restoring force is different while going towards two extreme shouldn't their amplitude be different ? $\endgroup$
    – user232991
    Commented Jun 30, 2019 at 17:26
  • $\begingroup$ @Who Don't want to be rude, but your sentence is incomplete. Note that I've added to my answer. $\endgroup$
    – Philip Wood
    Commented Jun 30, 2019 at 17:30
  • $\begingroup$ your answer explains the case where mass is displaced left .When the mass is displaced right won't K(x+a) +F add up? $\endgroup$
    – user232991
    Commented Jun 30, 2019 at 17:33
  • $\begingroup$ $F_0=ka$ holds whether the mass is displaced to the left or right of the equilibrium position, because we obtained it by considering the mass at equilibrium. So the net force to the right is $kx.$ If the body is displaced to the left of equilibrium, $x$ is positive (your convention); if the body is displaced to the right $x$ is negative $\endgroup$
    – Philip Wood
    Commented Jun 30, 2019 at 17:45
0
$\begingroup$

The addition of the constant F will shift the equilibrium position to the left. Then the "right hand amplitude" and the "left hand amplitude" is the same with respect to this new equilibrium position.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Why the amplitude is the same ? $\endgroup$
    – user232991
    Commented Jun 30, 2019 at 16:28
  • $\begingroup$ With respect to the original equilibrium position, the amplitude is not symmetric. However, with respect to the shifted equilibrium position, then the spring's force is determined by Hooke's law $F=kx'$, where $x'$ is measuring from the new equilibrium position. This is just another SHM system, i.e. the amplitude is symmetric. $\endgroup$
    – Leo L.
    Commented Jun 30, 2019 at 16:32
  • $\begingroup$ Can you please elaborate how will the the new amplitude will be symmetric about new equilibrium? $\endgroup$
    – user232991
    Commented Jun 30, 2019 at 17:28

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.