Proof that body performs SHM

Question

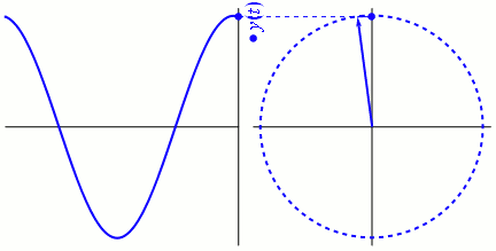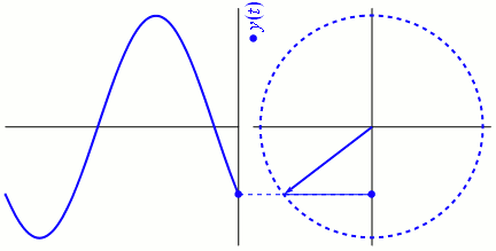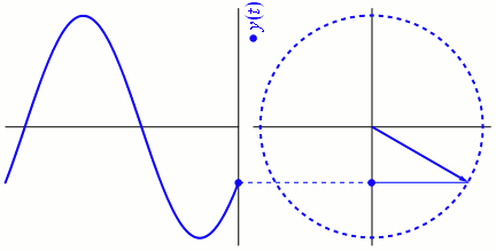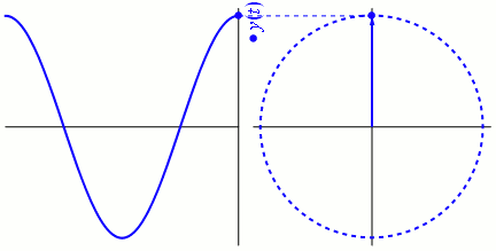
At core, SHM is the shadow of a particle revolving with omega on a circle of radius equal to amplitude. Now we say that body performs SHM either if the equation of its position makes a sinusoidal function or if its acceleration is proportional to displacement and in opposite direction.

I get how in the first way we can always equate it to the shadow of a particle revolving but in second way, i.e., by seeing its acceleration proportional to displacement how can we say for sure it has an equivalent particle shadow? Yes, if it starts from the mean position, we can make a similar shadow which starts from mean and the particle revolves with omega (according to acceleration of body and amplitude). What if the body starts with velocity v from any random distance from mean? How then can we be sure we will find its equivalence to a particle shadow?

Usually in that case, going by the questions we solved in class, we find the amplitude by equations of SHM itself. But we can't use the equation without proving SHM.

I am sure there is some obvious point here but I can't see it.

Answer 1

A defining equation for shm is $\ddot x = - \omega^2\,x$ where $x$ is the displacement from a fixed point, $\ddot x$ is the acceleration and $\omega^2$ is a positive constant which is a characteristic of the motion.

The solution to this second order differential equation requires two initial conditions and your the body starts with velocity v from any random distance from mean could be them as at time, $t=0$ you are given the displacement, $x_{\rm initial}$ and the velocity, $\dot x_{\rm initial}$.
From these you can get an equation of motion of the form $x(t) = A \sin (\omega\,t+\phi)$.

In terms of your projection idea, $A$ would be the radius of the circle and $\phi$ the position on the circle, relative to the $x$-axis, where the motion starts from at time $t=0$.

So in the animation below you can start the clock at any position on the circle and the projected point on the axis will execute shm, ie be a solution of $\ddot x = - \omega^2\,x$.