Why a Box moving at Constant Velocity isn't doing Work?

Question

This was a question from an A-Level past paper and the question is pretty straightforward.

I was in a hurry so I couldn't think much on this problem and chose $C$ as my answer, checked it on the answer key, and yes, my answer was correct. So I decided to briefly look at the options once again, and wondered why I chose $C$.

By definition $W=F×d$ , and according to Newton's 1st Law, an object at constant velocity means there isn't any unbalanced force acting on it, thus if $F$ is $0$ , it is said that the object isn't doing any work.

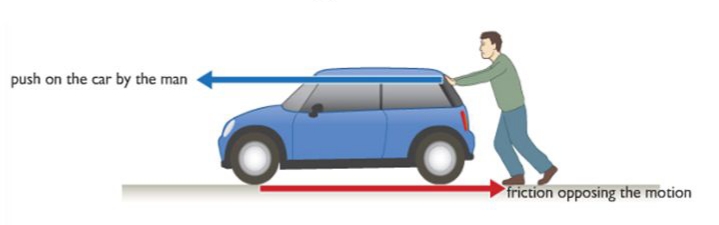So I changed the scenario a bit, imagine that I'm pushing a car at a constant velocity, according to the definition, I'm not doing work on the car, but that doesn't seem very intuitive to me. I'm clearly exerting my energy to push the car which eventually exhausts me.

Coming back to the first case, I assumed the surface, where the box was sliding, to be frictionless and is placed on a massive vacuum chamber. If my hand was initially pushing that box and after a while, I released it, it'll move at a constant velocity, this suddenly becomes sensible, as there is nothing pushing it and thus no work done.

It seems that when letting go a box with no resistive force, I wasn't doing any work, but when I was pushing the car, I did. It is somehow contradictive and confusing. What concept am I missing? Any concise explanation would be great!

Answer 1

Your example of pushing a car at a constant velocity is doing work, because you are applying force on the car.

The difference is that in that case, there is a friction force that also does work, in direct opposition to the work provided by pushing. The work done on the car by you is counteracted by the work done on the car due to friction, and thus net kinetic energy doesn't change, because both you and the ground are doing work on the car.

The key difference in the examples here is the inclusion of a force that opposes the motion. In the car example, if you stopped pushing, the friction would continue doing work on the car until it stops; which is why it requires a constant force (and thus work) to move the car.

Answer 2

A mathematical equation would really help you which is work energy theorem:- $$dW_{total}=dK_{system}$$ $$dK_{system}=0$$ $$dW_{total}=0$$

Hope this helps!

Answer 3

I believe you are not missing any concept and already pointed out the relevance of friction in the car example.

Intuitively, whenever anything happens that transfers energy from one form to another, you are doing work. In the car example you transfer energy from pushing into heating up the surface that the car has friction with. In the box example, there is no friction. Were the box accelerating, than there would be work required to push it to move ever faster. But since it moves at constant velocity, no energy is flowing from anywhere into anywhere else. The state does not change, the box will be moving the same way for eternity.