If displacement is 0, why isn't the work performed on an object 0?

Question

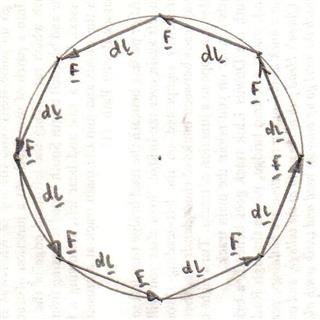
Work is force x distance/displacement. The exact definition is something I'm a little confused about since my professor said it could either be distance or displacement. So going with that, if the displacement is 0, then why isn't the work performed on an object 0?

I used an example when I explained my question to the professor. If you push on a cardboard box around a circular track (like in a school field), and the position of the box is the same on its return trip, then the displacement is 0. So regardless of the force exerted on the box, wouldn't F x 0 = 0, thus the work performed on the box being 0?

My professor said not to focus too much on the definitions of displacement/distance and that my statement is not necessarily true, and to figure it out on my own why the work isn't 0. I still don't understand this concept at all and I'm trying to understand why a displacement of 0 doesn't necessarily mean that Work performed is 0 either.

Answer 1

You must understand that work is firstly path dependent. Work = $\int F . dl $, this is a path integral. If displacement is 0, the work done need not necessarily be 0 if the direction of force is changing throughout the path. A simple example would be moving a block from A to B and back to A under the influence of friction. Even though the displacement is 0 as the direction of kinetic friction changes when you change the direction of motion and hence friction does indeed do work. Testimony to this is the heat dissipated during the process.

It is better to think of total work done as a sum of infinitesimal works $dW$. Hope this makes sense!

Answer 2

You have not computed the work done in taking each step when going around a complete circle.

It is always displacement that you should use but as shown below it so happens that in this case the total work done is the magnitude of the force $\times$ distance travelled around the circumference of the circle.

Imagine going around an octagon in steps $d\vec l$ whilst applying a force

$\vec F$ which is in the same direction as the step.

The work done in going around the complete octagon is $\Sigma \vec F \cdot d \vec l = F \;\Sigma dl$

Now make the step length smaller and smaller and smaller.
The octagon tends to a circle and $\Sigma dl$ tends to $2 \pi r$ where $r$ is the radius of a circle.

So the work done in going around a complete circle is $F2\pi r$.