0
$\begingroup$

I can't believe I haven't found an answer elsewhere.....

I have read repeatedly about blue/anti-blue gluons, etc., but no reason as to why they don't destroy each other immediately.....

Or maybe they do? But only after they have carried the 'charge' between quarks?

Edit: What about a red-antigreen gluon and an antired-green one?

Improve this question
$\endgroup$
8
  • 2
    $\begingroup$ You could ask this question in a much simpler context - when a gamma ray produces an electron-positron pair, why don't they immediately annihilate? The answer will likely be similar. $\endgroup$
    – probably_someone
    Commented Jun 17, 2019 at 17:51
  • 6
    $\begingroup$ There are no "green" gluons, nor "anti-green" ones. They transform in the adjoint. You may come up with charges of the type "green--anti-red" and so on. $\endgroup$
    – user178876
    Commented Jun 17, 2019 at 17:53
  • 2
    $\begingroup$ @marmot This is as good as an answer, if you wrote up a few framing sentences around it. The OP might not understand these two labels for a single particle.... $\endgroup$
    – Cosmas Zachos
    Commented Jun 17, 2019 at 18:53
  • 2
    $\begingroup$ Kurt, we don't have a set of gluons and a separate set of antigluons. Very roughly, a green quark can turn into a red quark by emitting a green-antired gluon, and if a red quark absorbs that gluon it will turn green. But that's just a rough "cartoon" of the actual QCD model. You need matrices & group theory to describe it properly. $\endgroup$
    – PM 2Ring
    Commented Jun 17, 2019 at 20:02
  • 3
    $\begingroup$ A single gluon has a color and an anticolor label. A green-antigreen gluon is the gauge QFT excitation coupling to a green quark and an antigen antiquark. As @marmot says, you cannot talk about three $\bar{G}G, ~ \bar{R} R, ~ \bar{B} B$ gluons, since they'd sum up to a color singlet, so no gluons. You must always be mindful that these are overstretched metaphors to summarize the math in codespeak, and hardly anything else. Taking these cartoons too seriously ignoring the math always leads to grief! $\endgroup$
    – Cosmas Zachos
    Commented Jun 17, 2019 at 21:35

1 Answer 1

Reset to default
4
$\begingroup$

Gluons transform in the adjoint representation. The charges "red", "green" and "blue" refer to the weights of the fundamental representation. So you may assign the gluons charges like "red"--"anti-green" (but this does not quite work out because this fails to take into account the "tracelessness" condition, i.e. you will naively get 9 instead of 8 gluons). A color-neutral combination of gluons can annihilate into photons, but not directly since gluons do not carry electric charge. The following shows a loop diagram that could with a lot of good will be interpreted as "gluon annihilation" (but recall that gluons are not asymptotically free states, so the term "annihilation" has to be interpreted in a somewhat unusual way). The second diagram shows that the answer by TEH is not entirely correct (but it is true to a very good approximation since the corresponding amplitude is highly suppressed).

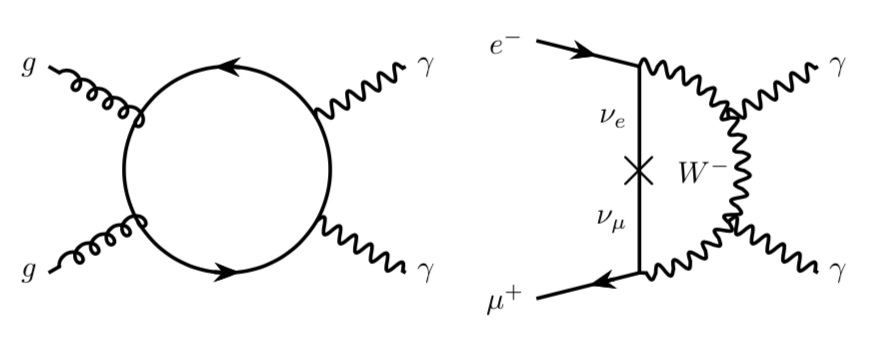
enter image description here

So the bottom-line is that, as long as you can form an invariant contraction of the incoming states, they can annihilate. In particular, gluons can, since their only quantum numbers are that they are octets under SU(3), and $\boldsymbol{8}\times\boldsymbol{8}$ contains a singlet. The annihilation rate may, however, be very much suppressed, as in the case of $e^-\mu^+\to\gamma\gamma$.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.