I was reading this and came across the statement
After releasing the knife, it will fly forward and continue to rotate around its center of gravity with the same angular velocity it had during the throwing movement.
However I'm not sure how to show that this would be true. Given the text diagram
Time 0 Pt A ------- %%CoM%%
Time 1 Pt A %%COM%%
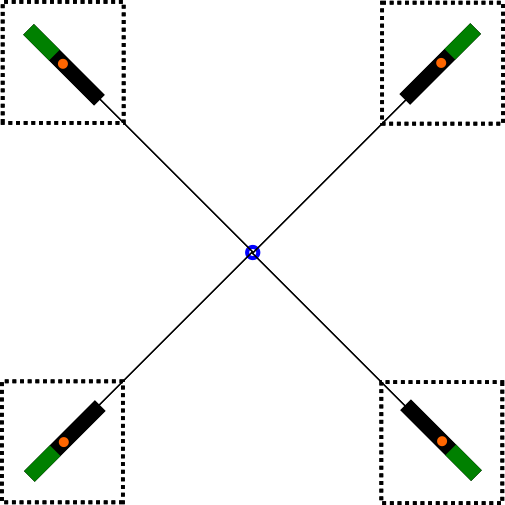
At time 0, the knife (or let's say rod) is rotating at $\omega$ (rad/sec) around Pt A. But does that mean the rod is rotating at $\omega$ around its own center of mass (CoM)? Pictorially, I convinced myself it's the case (see picture below). But I'm not sure how the equations would work out.
At time 1 -- even if the rod's angular velocity is the same around the two points (Pt A / CoM), does the it stay the same after throwing?
I've tried to think about it from the conservation of angular momentum $L$ perspective. Say we define a system that only includes the rod. I was worried that the arm produces some external torque. But I think since we are only pulling tangentially, there might not be any external torque on the rod. In which case, the inertia of the rod (around CoM) does not change and the angular velocity (around CoM) does not change either. Then the quoted statement holds.
Am I overthinking this? I've just made myself more and more confused. Any clarity appreciated -- maybe this is the wrong approach entirely.