In gaussian optics, a thick lens is just an abstraction that simplifies a system of thin lenses. Any number of thin lenses can be represented by a single thick lens. Sometimes the thickness ends up negative, but it's just an abstraction so it doesn't matter as long as the numbers work.
A thick lens can also approximate a real, physical lens, since a real lens can be approximated by two thin lenses (one between object-side and glass, and another between image-side and glass).
This thick lens abstraction behaves exactly like thin lens, with just one difference: the reference points for measuring all distances are different for the object and image sides. These reference points are called the front and rear principal points. If you measure all object-side distances from the front principal point and all image-side distances from the rear principal point, all the exact same formulas apply.
A reason you might come across different-looking equations is probably different conventions on naming, signs and reference points. Judging by the formula from your book, there seems to be just one coordinate system, in the middle between the two principal points. Everything to the right of it is positive (image side) and everything to the left is negative (object side). It also seems like $t$ is the distance between the origin and the principal points, so for all the formulae, this distance needs to be subtracted.
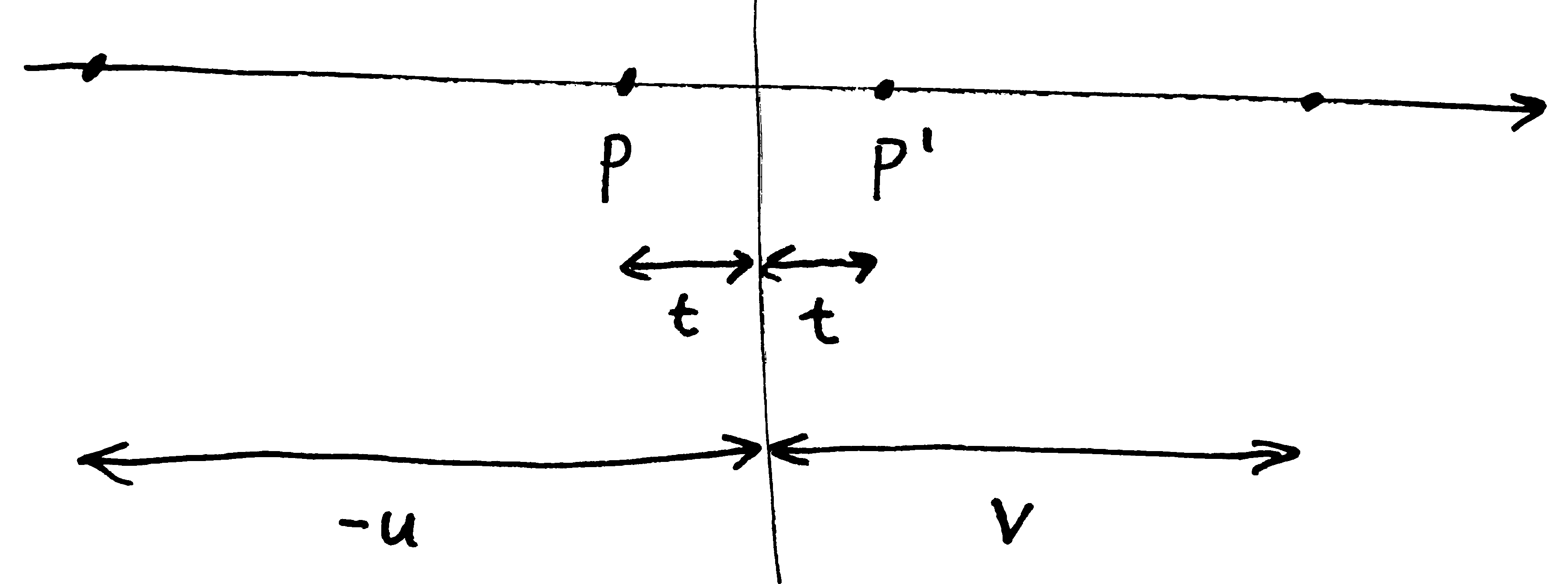
Here's your thin lens formula with signs rearranged a bit:
$$ \frac{1}{v} + \frac{1}{-u} = \frac{1}{f} $$
And here's with subtracting $t$ to get a thick lens version with one coordinate system:
$$ \frac{1}{v-t} + \frac{1}{-u-t} = \frac{1}{f} $$
$$ \frac{1}{v-t} - \frac{1}{u+t} = \frac{1}{f} $$

