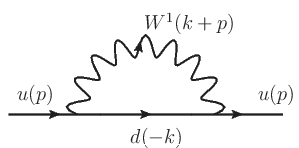
Imagine we want ot compute one of the diagrams for the self-energy of the quark $u$, with external momentum $p$. Inside the loop, we would have a $W^+$ and a $d$-quark propagator, with an undetermined loop momentum $k$ we will need to integrate over.
The Feynman rule for the vertex is
\begin{equation} ig\gamma^\mu \frac{1}{2}(1-\gamma_5) \equiv ig\gamma^\mu P_L, \end{equation} where $P_L$ is the left chiral projector.
The Feynman rule for a fermion propagator is
\begin{equation} i\frac{\gamma^\alpha k_\alpha + m}{k^2 - m^2 + i\varepsilon} \end{equation}
In the $R_\xi$ gauge, the Feynman rule for the propagator of a massive vector boson is
\begin{equation} D_{\mu\nu} = \frac{i}{k^{2}-M^{2}+i\varepsilon}\left[-g_{\mu \nu}+(1-\xi) \frac{ k_{\mu} k_{\nu}}{k^{2}-\xi M^{2}}\right]. \end{equation}
The unitary gauge is the so-called "physical" gauge, since in that gauge we do not have to consider the Goldstone bosons coming from the symmetry breaking and we only have to compute the diagrams concerning "physical" particles. Therefore, it may be interesting to use $\xi \rightarrow \infty$ to go to the unitary gauge, and the massive vector boson propagator would read
\begin{equation} D_{\mu\nu}^{\xi = \infty} = i\frac{-g_{\mu \nu}+ \frac{ k_{\mu} k_{\nu}}{M^{2}}}{k^{2}-M^{2}+i\varepsilon}. \end{equation}
However, if we want to compute the self-energy diagram above, we would have an integration over all loop momenta $k$,
\begin{equation} \mathcal M = \int \frac{d^d k}{(2\pi)^d} \bar{u}(p) \ (i g \gamma^\mu P_L) \frac{i(\gamma^\alpha k_\alpha+m)}{k^2-m^2 - i\varepsilon} D_{\mu\nu} (i g \gamma^\nu P_L) \ u(p). \end{equation}
Is it then legitimate to go to the unitary gauge limit and use $D_{\mu\nu}^{\xi = \infty}$ instead of $D_{\mu\nu}$?
If not, what is the right thing to do?