I'm trying to explicitly compute the following box diagram in the Feynman-t'Hooft gauge:
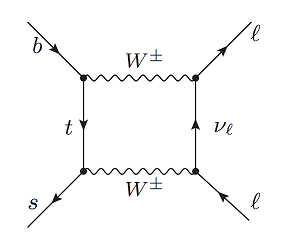
If I neglect the impulsion of the $s$ quark, then the final amplitude is given by
$$\mathcal{A} \propto [\bar{s}(0) \gamma_\alpha \gamma_\delta \gamma_\mu P_L b(p_b)] \cdot [\bar{u}(p_1) \gamma^\mu \gamma^\Delta \gamma^\alpha P_L v(p_2)]\cdot I^\delta_\Delta,$$ where
$$ I^{\delta\Delta}=\int \frac{\mathrm{d}^4k}{(2\pi)^4} \frac{k^\delta(k-p_2)^\Delta}{[k^2-m_t^2][(k-p_b)^2-m_W^2][k^2-m_W^2][(k-p_2)^2-m_W^2]}.$$
If I consider vanishing external momenta $p_b$ and $p_2$, then it is easy to compute this integral and express the final amplitude in terms of $m_t$ and $m_W$. However, when I carry out this computation in the general case, I don't know how to simplify the final amplitude. More precisely, I find some expressions like
$$\mathcal{A}\propto [\bar{s}(0) \gamma_\mu p_2^\mu P_L b(p_b)] \cdot [\bar{u}(p_1) P_L v(p_2)]+\dots $$ and I don't know how to get rid of the impulsion $p_2$ in the left part of the r.h.s of the equation. I can't use Dirac's equation, because $p_2$ is in the "wrong" current and the conservation of 4-momenta is completely useless, because this would just replace $p_2$ by $p_1$.
Could you give some hints about how to simplify this expression? Some references about the effect of external momenta in box diagrams might also be helpful.
