Amplitude associated with $\bar{b}+\bar{\nu_\mu}+\bar{d}\rightarrow b + u + \mu^{-}$ Feynman diagram of lowest order

Question

I was reading Introduction to Elementary Particles by Griffiths and in particular the sixth chapter about the golden rule for a "Toy Theory". In this chapter, six rules to find the amplitude $M$ associated with a certain Feynman diagram. Then the example is applied to two cases:

• Lowest-order contribution to $A\rightarrow B+C$ with $A$, $B$ and $C$ spinless particles Section 6.3.2.
• 6.3.3 Higher-order Diagrams describes the lowest-order of spinless $A+A \rightarrow B+B$

This exercise seemed interesting so I thought I could pick a random Feynmann diagram and try to apply the rules to it. I know quarks have spin 1/2, gluons have spin 1 (except $H^0$), but I will assume for the purpose of this exercise I am working with spinless particles.

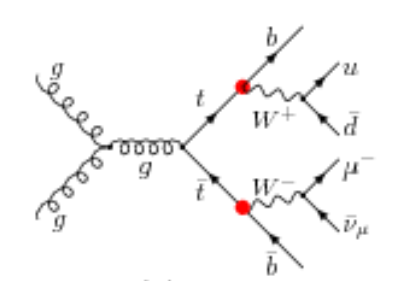
I picked here a Feynman diagram among others of $\bar{b}+\bar{\nu_\mu}+\bar{d}\rightarrow b + u + \mu^{-}$:

Step 1: Notation

1. Notation: Label the incoming and outgoing four-momenta $p_1 , p_2 , \dots , p_n$. Label the internal momenta $q_1, q_2, \dots$. Put an arrow beside each line, to keep track of the 'positive direction' (forward in time for external lines, arbitrary for internal lines

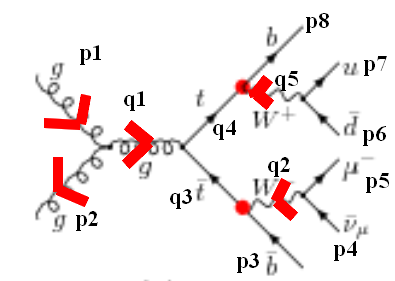
Arrows are already present on non-gluons so I need here to label the four-momenta and put an arrow on gluons.

My Feynman diagram now looks like this:

Step 2: Vertex factors

2.Vertex factors: For each vertex, write down a factor $-ig$. $g$ is called the coupling constant; it specifies the strength of the interaction between $A$, $B$ and $C$. In this toy theory, $g$ has the dimensions of momentum; in 'real-world' theories, we shall encounter later on, the coupling constant is always dimensionless.

The diagram has 6=1+1+2*2 vertices: 1 with three gluons, one to transform top to antitop and 2 of each branched part of the tree. Therefore the factor is $\left(-ig \right) ^{6} = - g^6$.

Step 3: Propagators

3. Propagators: For each internal line, write a factor $\frac{i}{q_j^2 - m_j^2 c^2}$ where $q_j$ is the four-momentum of the line and $m_j$ is the mass of the particle the line describes. (Note that $q_j^2 \neq m_j^2 c^2$, because a virtual particle does not lie on its mass shell.)

I will do it in the order I named $q_i$.

1. The first internal line represents a gluon with a mass of zero. I am not sure if this is correct, but since we assumed we were doing the exercise as mentioned in the chapter 6, I juste note $m_g$ despite knowing it is zero. The four-momenta is $q_1$. The factor of this internal line is: $\frac{i}{q_1^2 - m_g^2 c^2}$.
2. The second internal line represents a $W^-$ boson particle whose mass is $m_W = 80.385±0.015 MeV/c^2$. The four-momenta is $q_2$. The factor of this internal line is: $\frac{i}{q_2^2 - m_W^2 c^2}$.
3. The third internal line represents an antitop quark whose mass is $m_t = 173,100±600 MeV/c²$. The factor of this internal line is: $\frac{i}{q_3^2 - m_t^2 c^2}$.
4. The forth internal line represents a top quark. The factor of this internal line is: $\frac{i}{q_4^2 - m_t^2 c^2}$.
5. The fifth internal line represents a $W^+$ boson antiparticle. The factor for this internal line is: $\frac{i}{q_5^2 - m_W^2 c^2}$.

I am left with the following Step 3 factor: $$\frac{-i}{\left(q_1^2 - m_g^2 c^2\right) \left(q_2^2 - m_W^2 c^2\right)\left(q_3^2 - m_t^2 c^2\right)\left(q_4^2 - m_t^2 c^2\right)\left(q_5^2 - m_W^2 c^2\right)} $$

The overall factor up to this point is:

$$\frac{i g^6}{\left(q_1^2 - m_g^2 c^2\right) \left(q_2^2 - m_W^2 c^2\right)\left(q_3^2 - m_t^2 c^2\right)\left(q_4^2 - m_t^2 c^2\right)\left(q_5^2 - m_W^2 c^2\right)} $$

Step 4: Conservation of energy and momentum

4. Conservation of energy and momentum: For each vertex, write a delta function of the form $\left( 2 \pi \right) ^4 \delta^4 \left( k_1 + k_2 + k_3 \right)$ where the $k$'s are the three four-momenta coming into the vertex (if the arrow leads outward, the $k$ is minus the four-momentum of that line). This factor imposes the conservation of energy and momentum at each vertex, since the delta function is zero unless the sum of the incoming momenta equals the sum of the outgoing momenta.

We have six vertices, therefore the non-delta part of this factor is $\left(2 \pi\right)^{4 \times 6} = \left(2 \pi\right)^{24}$. The delta parts of this factor becomes, in the order of Step 2:

1. $\delta^4 \left( p_1 - p_2 - q_1 \right)$
2. $\delta^4 \left( q_1 + q_3 - q_4 \right)$
3. $\delta^4 \left( p_4 - p_5 - q_2 \right)$
4. $\delta^4 \left( p_3 + q_2 - q_3 \right)$
5. $\delta^4 \left( p_6 - p_7 - q_5 \right)$
6. $\delta^4 \left( q_4 - q_5 - p_8 \right)$

The factor of this step becomes:

$$\left(2 \pi\right)^{24} \cdot \delta^4 \left( p_1 - p_2 - q_1 \right) \delta^4 \left( q_1 + q_3 - q_4 \right) \delta^4 \left( p_4 - p_5 - q_2 \right)\delta^4 \left( p_3 + q_2 - q_3 \right)\delta^4 \left( p_6 - p_7 - q_5 \right)\delta^4 \left( q_4 - q_5 - p_8 \right)$$

The factor up to now is therefore:

$$\frac{i g^6 \cdot \left[ \left(2 \pi\right)^{24} \cdot \delta^4 \left( p_1 - p_2 - q_1 \right) $\delta^4 \left( q_1 + q_3 - q_4 \right) \delta^4 \left( p_4 - p_5 - q_2 \right)\delta^4 \left( p_3 + q_2 - q_3 \right)\delta^4 \left( p_6 - p_7 - q_5 \right)\delta^4 \left( q_4 - q_5 - p_8 \right) \right]}{\left(q_1^2 - m_g^2 c^2\right) \left(q_2^2 - m_W^2 c^2\right)\left(q_3^2 - m_t^2 c^2\right)\left(q_4^2 - m_t^2 c^2\right)\left(q_5^2 - m_W^2 c^2\right)} $$

Step 5: Integration over internal momenta

5. Integration over internal momenta: For each internal line, write down a factor $\dagger$ $\frac{1}{\left( 2\pi \right)^{4} } d^4 q_j$ and integrate over all internal momenta.

The note at the bottom of the page states the following:

Notice (again) that every $\delta$ gets a factor of $(2\pi)$ and every $d$ gets a factor of $1/(2\pi)$.

About the factors $(2\pi)$ we had $6$ vertices so $4 \times 6$ bundles of them. Now we reduce it by 5 because we have fives internal lines: $4 \times (6-5) = 4$. We are left with:

$$\int_{q_1} d^4 q_1 \int_{q_2} d^4 q_2 \int_{q_3} d^4 q_3 \int_{q_4} d^4 q_4 \int_{q_5} d^4 q_5 \frac{i g^6 \cdot \left[ \left(2 \pi\right)^{4} \cdot \delta^4 \left( p_1 - p_2 - q_1 \right) \delta^4 \left( q_1 + q_3 - q_4 \right) \delta^4 \left( p_4 - p_5 - q_2 \right)\delta^4 \left( p_3 + q_2 - q_3 \right)\delta^4 \left( p_6 - p_7 - q_5 \right)\delta^4 \left( q_4 - q_5 - p_8 \right) \right]}{\left(q_1^2 - m_g^2 c^2\right) \left(q_2^2 - m_W^2 c^2\right)\left(q_3^2 - m_t^2 c^2\right)\left(q_4^2 - m_t^2 c^2\right)\left(q_5^2 - m_W^2 c^2\right)} $$

We need to 'discard', as Griffiths calls them, $q_i$, we send $q_1 \rightarrow p_1 - p_2$. The factor becomes:

$$\int_{q_2} d^4 q_2 \int_{q_3} d^4 q_3 \int_{q_4} d^4 q_4 \int_{q_5} d^4 q_5 \frac{i g^6 \cdot \left[ \left(2 \pi\right)^{4} \cdot \delta^4 \left( p_1 - p_2+ q_3 - q_4 \right) \delta^4 \left( p_4 - p_5 - q_2 \right)\delta^4 \left( p_3 + q_2 - q_3 \right)\delta^4 \left( p_6 - p_7 - q_5 \right)\delta^4 \left( q_4 - q_5 - p_8 \right) \right]}{\left(\left( p_1 - p_2 \right)^2 - m_g^2 c^2\right) \left(q_2^2 - m_W^2 c^2\right)\left(q_3^2 - m_t^2 c^2\right)\left(q_4^2 - m_t^2 c^2\right)\left(q_5^2 - m_W^2 c^2\right)} $$

We got rid of $q_1$ we do the same with $q_2$ and $q_5$ (by symmetry) and we send $q_2 \rightarrow p_4 - p_5$ and $q_5 \rightarrow p_6 - p_7$. We can both because there is no unknown internal four-momenta that would give us trouble.

We get the following factor:

$$ \int_{q_3} d^4 q_3 \int_{q_4} d^4 q_4 \frac{i g^6 \cdot \left[ \left(2 \pi \right)^{4} \cdot \delta^4 \left( p_1 - p_2+ q_3 - q_4 \right) \delta^4 \left( p_3 + p_4 - p_5 - q_3 \right)\delta^4 \left( q_4 + p_7 - p_6 - p_8 \right) \right]}{\left(\left( p_1 - p_2 \right)^2 - m_g^2 c^2\right) \left( \left( p_4 - p_5 \right)^2 - m_W^2 c^2\right)\left(q_3^2 - m_t^2 c^2\right)\left(q_4^2 - m_t^2 c^2\right)\left( \left( p_6 - p_7 \right)^2 - m_W^2 c^2\right)} $$

The second and third Dirac help us send $q_3 \rightarrow p_3 + p_4 - p_5$ and $q_4 \rightarrow p_6 - p_7 + p_8$.

The factor becomes:

$$ \frac{i g^6 \cdot \left[ \left(2 \pi \right)^{4} \cdot \delta^4 \left( p_1 - p_2+ p_3 + p_4 - p_5 - p_6 + p_7 - p_8 \right) \right]}{\left(\left( p_1 - p_2 \right)^2 - m_g^2 c^2\right) \left( \left( p_4 - p_5 \right)^2 - m_W^2 c^2\right)\left( \left( p_3 + p_4 - p_5 \right)^2 - m_t^2 c^2\right)\left( \left( p_6 - p_7 + p_8 \right)^2 - m_t^2 c^2\right)\left( \left( p_6 - p_7 \right)^2 - m_W^2 c^2\right)} $$

Step 6: Cancel the delta function

6. Cancel the delta function: The result will include a delta function $\left( 2 \pi \right)^{4} \delta^{4} (p_1 + p_2 + \dots - p_n)$ reflecting overall conservation of energy and momentum. Erase this factor $\dagger$ and multiply by $i$. The result is $M$.

With the note at the bottom of the page:

Of course, the Golden Rule immediately puts this factor back in Equations 6.15 and 6.37, and you might wonder why we don't just keep it in $M$. The problem is that $|M|^2$, not $M$, comes into the Golden Rule and the square of a delta function is undefined. So you have to remove it here, even though you'll be putting it back at the next stage.

The Dirac we found is fitting well the conservation of four-momenta, as expected.

We get:

$$ M = \frac{- g^6}{\left(\left( p_1 - p_2 \right)^2 - m_g^2 c^2\right) \left( \left( p_4 - p_5 \right)^2 - m_W^2 c^2\right)\left( \left( p_3 + p_4 - p_5 \right)^2 - m_t^2 c^2\right)\left( \left( p_6 - p_7 + p_8 \right)^2 - m_t^2 c^2\right)\left( \left( p_6 - p_7 \right)^2 - m_W^2 c^2\right)}$$

Remarks:

1. It feels like we could go faster by writing four momenta conservation first as a linear combination of in-going and out-going momentum rather than to have to name $q_i$ every time.
2. The $\left( 2 \pi \right)$ factor feels like it could be summarized $\left( 2 \pi \right)^{4\times \left( \text{vertices} - \text{internal lines} \right)}$

Question:

Is my reasoning correct assuming I was dealing with spinless particles? Feel free to leave any constructive comment as I am a very beginner in this domain and I feel like it was an interesting exercise.

Answer 1

Yes, your final result is correct, assuming you're treating everything as a scalar.

As you noticed, Griffiths' rules are a little bit clunky. They were chosen to be as explicit as possible, so people have a chance to see everything working. If you go to a "proper" quantum field theory textbook, its Feynman rules for the same theory will be more streamlined:

1. Give each line a directed momentum, using momentum conservation as much as possible. For a "tree" diagram, this will automatically fix all the momenta. But in general, you can have "loops" in the diagram that have an extra, arbitrary momentum $p$ running around them.
2. For each loop, write an integral $d^4p / (2\pi)^4$ over the loop momentum.
3. For each internal line of momentum $q$, write down $i/(q^2 - m_0^2 + i \epsilon)$.
4. Multiply by $\sqrt{Z}^{n}$, where $n$ is the total number of incoming and outgoing particles, and $Z$ is the field strength renormalization factor.
5. Divide by the symmetry factor.

The result of this procedure will be the contribution to $i \mathcal{M}$ from that diagram. Using these rules, one would simply write down your final answer in a single step. There's no need to write down piles of integrals and delta functions when you know they're all going to cancel out anyway.

Here, steps (1), (2), and (3) are just a more efficient version of what Griffiths has (which you can check give the same result). Step (4) is more subtle and is required when you deal with loop diagrams, which Griffiths never does. Step (5) is an annoying technicality that Griffiths avoids.