-1
$\begingroup$

In the double slit experiment I know that the electron fires as a particle one at a time then splits goes through both slits and recombined and interferes with itself and hit the wall creating a interference pattern.

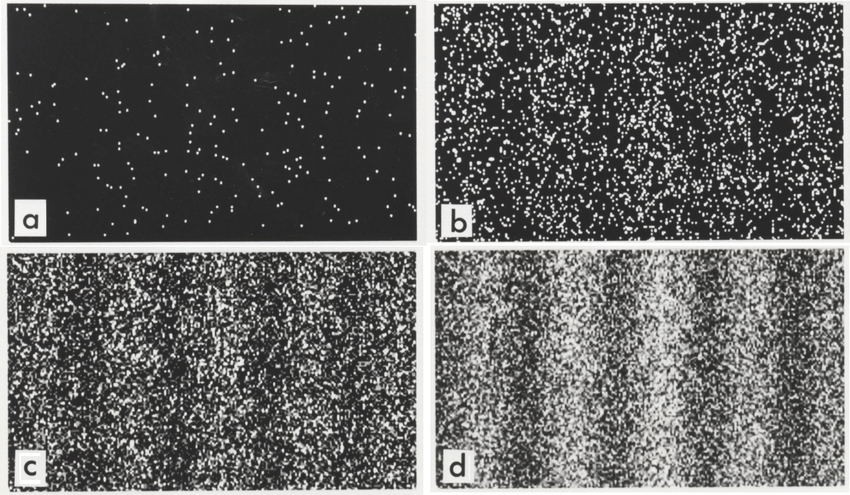
double slit electron interference pattern

But I don’t understand how it can just change just by observing it. Like say you’re in the middle of a experiment and the interference pattern is building up and say if an ant sees the experiment how does that just make the electrons behave as a particle and rewrite the past and get rid of the interference pattern it had been building up.

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ "Interferes with itself" is a term from the early 1900s, better to say the electron path is effected by the geometry of both slits. The path is the wave function, due to the wavelength of the electron the path is not ideal for the dark spots, it is difficult to travel there. The bright spots are where electrons wavelength matches better with the distance travelled. $\endgroup$
    – PhysicsDave
    Commented Jun 4, 2019 at 2:17
  • 3
    $\begingroup$ Just looking at the experiment doesn't wreck the interference pattern, whether the observer is a human, an ant, or a computer. You need to detect which slit each electron passes through to do that. $\endgroup$
    – PM 2Ring
    Commented Jun 4, 2019 at 3:03

2 Answers 2

Reset to default
1
$\begingroup$

Take it like this: there is an interaction between the edges and the electron. The electron is deflected near the edges.
If you want to observe the electron on its way to the screen, you disturb the electron much more than when it interacts with the edge. The pattern on the screen is disturbed.

Another thing: Not you, not me, not everyone has ever measured the edge-electron interaction. All we interpret are the edges on the screen.
But I'm sure there's a way to observe this interaction. As a result of the disturbance of the edges by the electron excitations called phonons, they fade away inside the wall.
They may be measurable. One measurement for the left side of the wall and one for the right side. And transmitted one by one electrons.

Improve this answer
$\endgroup$
3
0
$\begingroup$

What is seen in the bottom frame on the right , is a probability distribution: how probable is it to find an electron at the (x,y) of the screen. On the top left you see an invidual electron , whole, leaving a footprint on the screen consistent with a classical particle footprint. There is no spread of energy in space, as would be the case of the electron were really a wave, like water waves.

What is waving is the probability distribution, which reproduces a classical wave interference pattern.

The electron is a quantum mechanical entity/particle and the experiment is a scattering experiment "electron" scattering off "two slits a specific width and specific distance apart". The quantum mechanical solution of the problem is a wave function, $Ψ$, which in the theory of quantum mechanics will predict the probability distribution as $Ψ^*Ψ$.

So the electron passes as a whole from either slit with a given probability finally measured in the last frame.

Observing in physics means detecting interactions, not "looking" or "imagining".

Trying to check which slit the electron goes through changes the boundary conditions of the problem, because of the detectors introduced. It is a different scattering experiment has different $Ψ$ solutions which destroy the probability pattern seen in the last frame. This has been explored here

Overall, the results suggest that the type of scattering an electron undergoes determines the mark it leaves on the back wall, and that a detector at one of the slits can change the type of scattering. The physicists concluded that, while elastically scattered electrons can cause an interference pattern, the inelastically scattered electrons do not contribute to the interference process

Improve this answer
$\endgroup$
4
  • $\begingroup$ Can you please tell me if they figured out what the real difference between the elastic and inelastic scattering was in terms of destroying the pattern? I do understand that inelastic scattering means that the photons' or electrons' energy will change and phase too, but how does that destroy the pattern? $\endgroup$
    – Árpád Szendrei
    Commented Jun 4, 2019 at 4:23
  • $\begingroup$ @ÁrpádSzendrei the inelastic scattering in the "which way" experiment comes from new point sources, the electrons scattering on the fields from the sides of the slits or detector . As new sources they are incoherent even if the energy difference is small $\endgroup$
    – anna v
    Commented Jun 4, 2019 at 4:38
  • $\begingroup$ are you saying that as the electrons scatter on the detector, they act as new point sources? So it is like if they were emitted/shot by new sources at new positions in space and thus they are incoherent with the original source (the electron gun)? $\endgroup$
    – Árpád Szendrei
    Commented Jun 4, 2019 at 4:46
  • $\begingroup$ I am saying that the picture above is a coherent QM scattering for the given geometry. This coherence is destroyed when extra boundary conditions enter as in the experiment linked. The extra scatterings are no longer coherent with the Ψ of the original experiment, the interactions of the point sources on the extra detection is out of step and the pattern is destroyed. $\endgroup$
    – anna v
    Commented Jun 4, 2019 at 4:50

Not the answer you're looking for? Browse other questions tagged or ask your own question.