Is acceleration of particle by photon a continuous process?

Question

There are a lot of questions, so I assigned a number to each one.

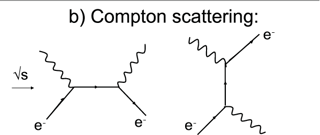
Let's assume we have electron which is not moving in some inertial frame of reference. Let this electron be hit by a photon. Since photon has a momentum, the electron start's to move with constant velocity v. Thus it changed its velocity from 0 to v over some time t, so this would imply acceleration.

Is it true that during such process there is some amount of time, for which the electron has acceleration, or rather such "time of interaction" is singular/infinitesimal(1)? If the latter is true, then wouldn't that mean that speeding up particle is non continuous process(2)?

But if speeding up is a continuous process, then since electron is accelerated for some amount of time, can it spontaneously emit the photon back to the universe during that time interval(3)? Would that mean it could bounce the whole photon back, or deflect it, or emit photon with smaller energy in some direction, and start moving in yet another direction(4)?

Can it even emit a photon with higher energy than it initially absorbed(5)? For that one we can assume that earlier our electron absorbed the photon and started to move, and then it was hit by another photon, which would give the electron an "acceleration window", which would allow emit back both photons(or one with the combined energy).

If in the above scenario, the answer is affirmative, then would it be still affirmative, if the electron would be hit by the first photon, then after it got constant velocity, we would change frame of reference to such the electron is not moving, and then hit it with a second electron(6)?

Note that if the answer for question (2) is affirmative, then questions (3), (4), (5) and (6) do not make sense anymore.

Answer 1

Electrons and photons are elementary particles in the standard model of particle physics, quantum mechanical entities, and cannot be modeled by using classical physics or classical electrodynamics.

Ones studies the interactions between elementary particles using Feynman diagrams , in your case the diagrams describing electron photon scattering.

At the vertex there is a dp/dt exchanged which is what force is at the quantum level. Elastic scattering also is represented in this way, when in the center of mass of the interaction the energies are unchanged and only the angles change.

1) there is no "time" in the sense you envisage, because energy and momentum are transmitted only once through a vertex and an exchanged virtual particle. The diagrema is a repersentation of an integral, and the virtual particle is under the integral, a mathematical tool. There is a quantum indeterminacy on time, due to the probabilistic nature of quantum mechanics, but not connected with any radiation.

2) If there were continuous acceleration and a second particle/photon , one would have to draw a diagram with extra vertices introducing more dp/dt. The more vertices one introduces the smaller the contribution of the diagram to the crossection, because of the 1/137 of the electromagnetic coupling constant which will enter multiplicatively in the integrals represented by the feynman diagrams. There are no continuous impulses

Energy is conserved , and it is energy that is absorbed and reemitted with a photon, the electron's mass does not change because it interacted with a photon, it is only the virtual electron that has a continuously under the integral variable mass. It is all in the maths.

Answer 2

The problem here is thinking about electron-photon collision in classical terms, as if it were described by Newton's equations... in fact, we have a collision from a state $p=0,\hbar k$ to state $p', \hbar k'$, where the collision process is governed by the Schrödinger equation - the notions such as acceleration or direction of motion are simply not defined here.

Mathematically the collision time is infinite, but practically there are other factors that limit it (e.g., in accelerators).

See also Definition of collision