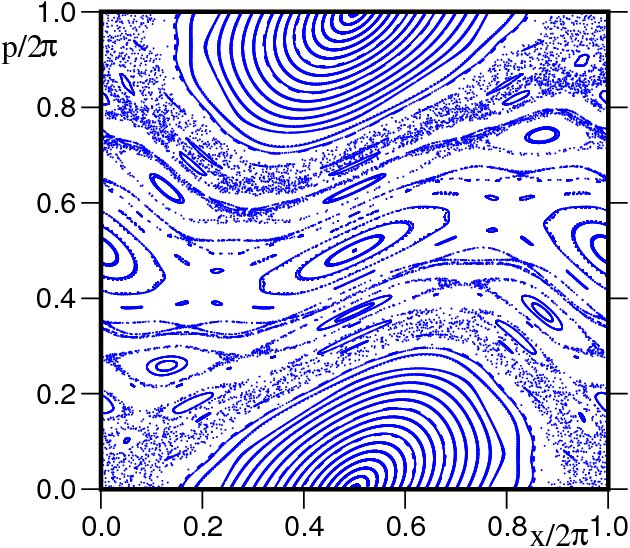
In a recent lecture on ergodicity and many-body localization, the presenter, Dmitry Abanin, mentioned that it is possible for a classical dynamical system to be chaotic but still fail to obey the ergodic hypothesis, which is frankly a pretty remarkable combination of properties. Unfortunately, the lecture had a lot of ground to cover and Abanin did not elaborate.
So:
- Are any explicit examples known that have been shown to be both chaotic and non-ergodic?
- Is there some clear explanation for what properties of those systems allow them to show this behaviour?