Viscous flow through a concave tube

Question

Consider gravity-driven incompressible flow through a concave tube with known radius $R(z)$, where pressure is atmospheric $P_a$ on both ends.

How does the viscous pressure distribution $P(z)$ compare with that of purely inviscid flow?

I think both flows should definitely have a pressure drop, so that $P(z) < P_a$ throughout the tube. Furthermore, I think the viscous flow should result in a larger pressure drop to balance the shear stresses that don't exist in inviscid flow. I therefore think the pressure distributions for viscous and inviscid flow should look something like this:

Is this qualitatively correct?

Answer 1

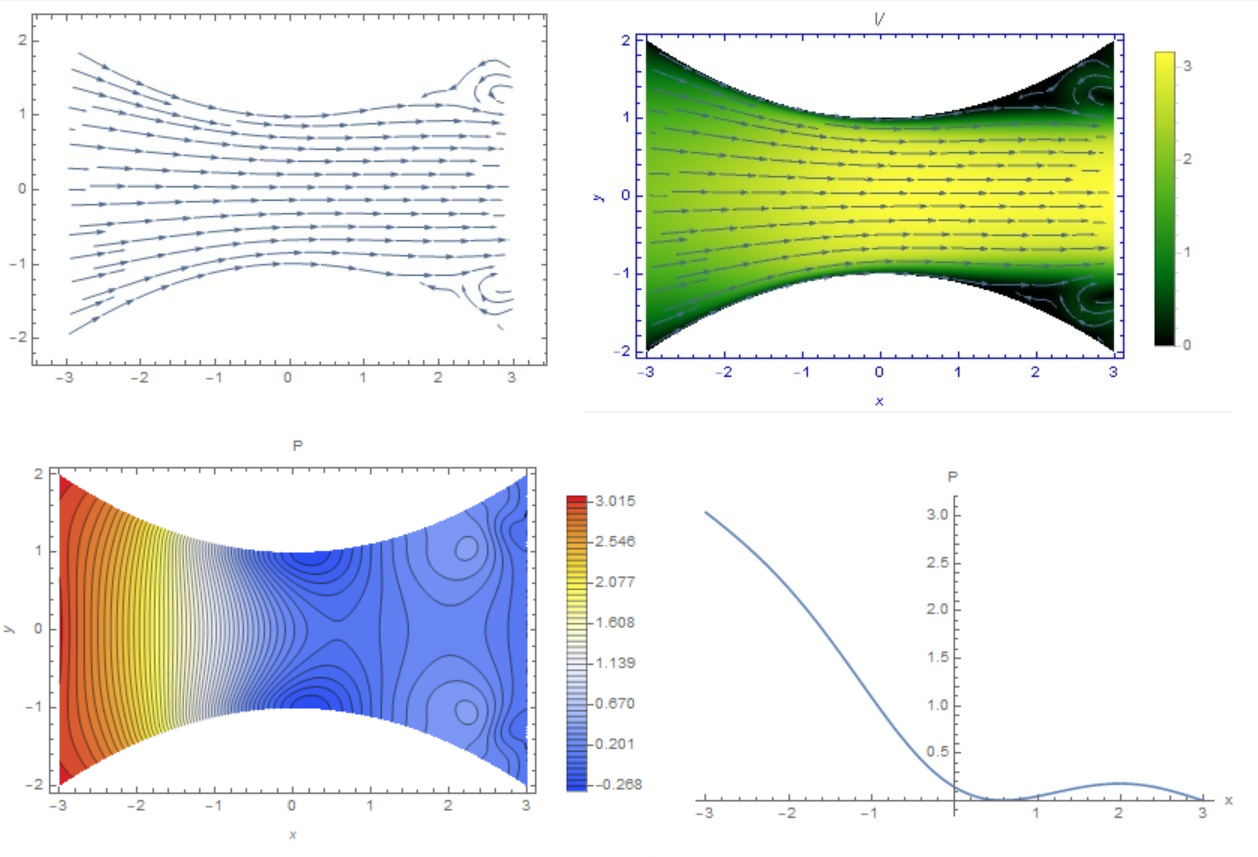
In the case of viscous flow, I gave the answer here. Viscous laminar flow during expansion of the nozzle will come off the walls with the formation of a jet and return flow zones - see Figure 1.

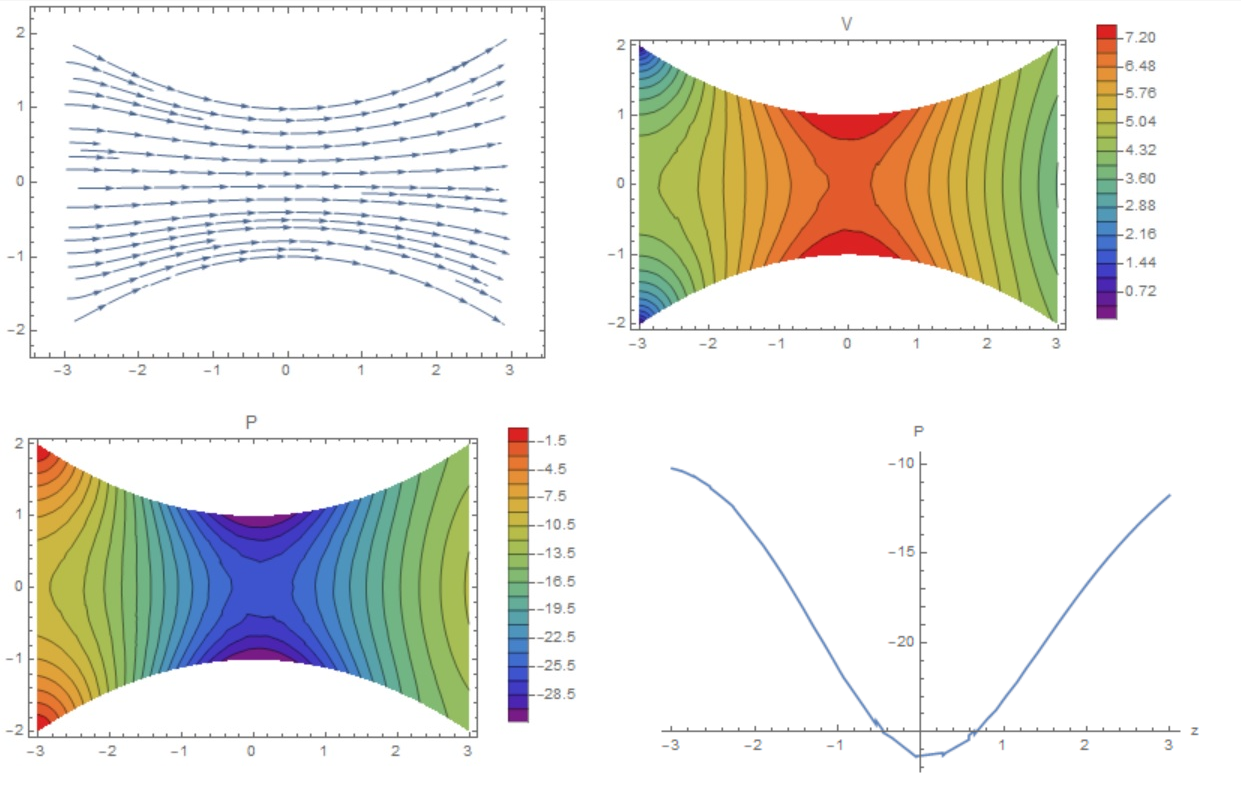
In the case of an inviscid continuous flow, the distribution of velocity and pressure is shown in Fig.2. In this case, the pressure distribution on the axles is similar to what @Drew drew. In both cases, a parabolic velocity profile is specified at the input.

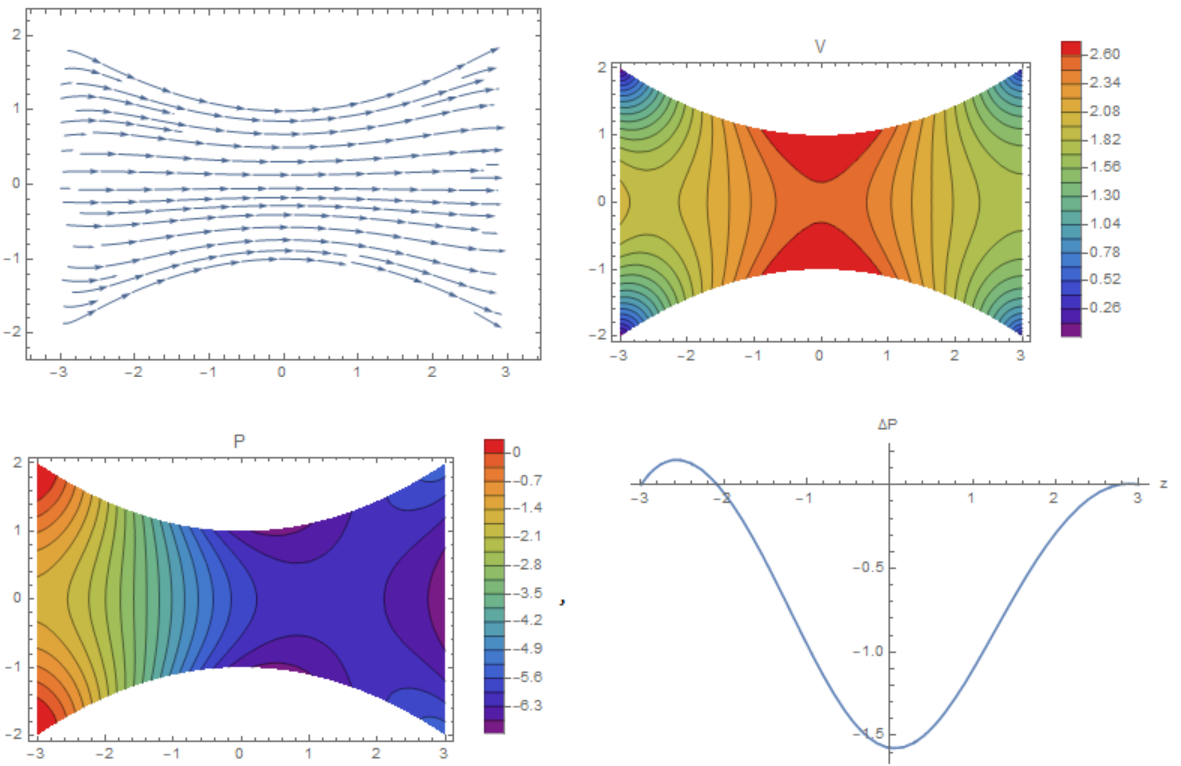
Align the pressure in the viscous flow at the inlet and outlet of the pipe by subtracting $\rho gz$. The distribution of the velocity of the flow does not change, and the distribution of pressure on the axis takes the form similar to that painted @Drew.

Now we need to calibrate the two solutions so that we can coordinate the pressure on the axis. First, we can calibrate by the water flow rate at the pipe inlet by setting the same velocity profile. Such inviscid flow is shown in Fig.4, where $\Delta P=P-\rho gz$.

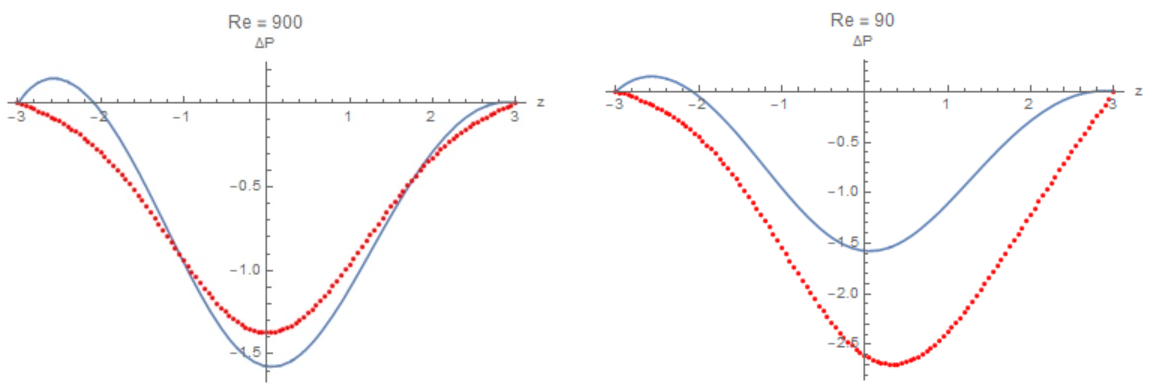
Finally, it is possible to compare two pressure profiles on the axis for viscous (red) and inviscid flow (blue) with the same velocity profile at the inlet to the pipe - Figure 5.

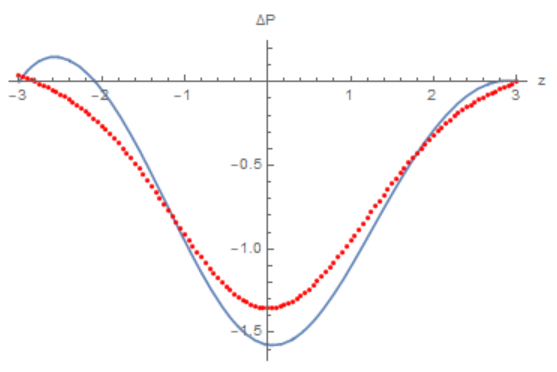
However, if we reduce the Reynolds number to $Re=90$ and create a non-separable flow, then the pressure distribution will be similar to what @Drew suggested - see Figure 6 (right).