1
$\begingroup$

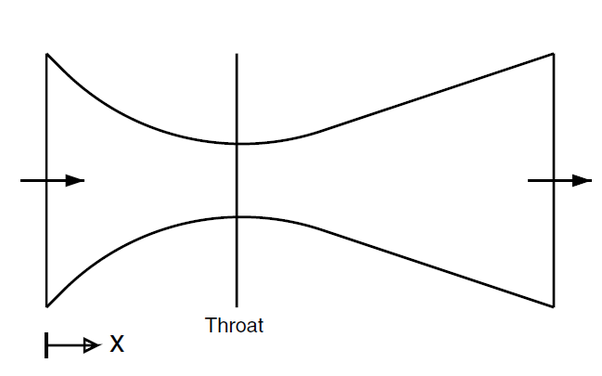
Consider flow through the converging and diverging portions of a tube with varying radius: enter image description here

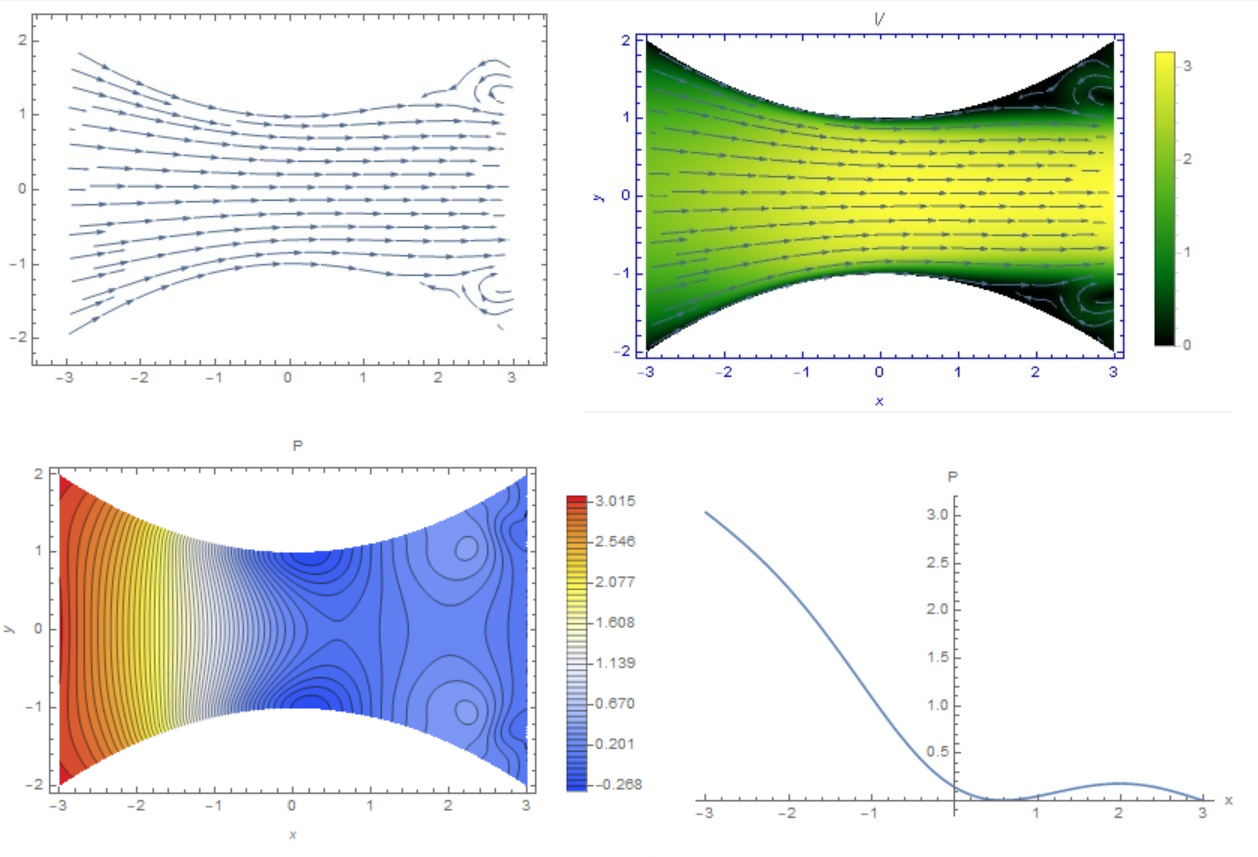
In inviscid flow, Bernoulli's equation states that velocity at the throat will be the highest, so this area will have the lowest pressure.

If the flow is viscous and locally fully developed throughout, however, then my intuition says that the throat will have the highest pressure. Is this true?

I reason this is true because the volume flow rate $Q$ must stay constant in an incompressible flow. If the flow is locally fully developed at each point $x$, then the flow rate is:

$$ Q = \frac{\pi R^4}{8 \mu}(\frac{-dP}{dx})$$

As $R(x)$ decreases, then $\frac{-dP}{dx}$ must increase in the algebraic sense. The only way this is possible, and to keep pressure continuity at the throat, is if the pressure distribution has a maximum at the throat.

Does viscous flow through a nozzle result in an increase in pressure, opposite of what inviscid flow predicts?

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ No. Viscous friction is dissipative and always causes the pressure to decrease in the flow direction, while non-viscous inertial effects associated with the Bernoulli equation can result in either a pressure decrease (as in the converging section) or a pressure increase (as in the diverging section). In viscous flows, both mechanisms are present simultaneously. $\endgroup$
    – Chet Miller
    Commented May 8, 2019 at 23:10
  • $\begingroup$ @ChetMiller So let's say the tube was placed vertically so that gravity was the only driving force, and we poured viscous fluid down it. Wouldn't that allow for a pressure increase with flow direction? $\endgroup$
    – Thermodynamix
    Commented May 8, 2019 at 23:35
  • $\begingroup$ Yes, that is certainly possible. But the pressure increase would be less with viscous effects in play. So the viscous effects would contribute a pressure decrease in the flow direction. Still, I stand corrected. Thanks @Drew. $\endgroup$
    – Chet Miller
    Commented May 9, 2019 at 0:06

1 Answer 1

Reset to default
1
$\begingroup$

Figure 1 shows the distribution of velocity (top) and pressure (bottom) in a laminar flow in a channel of variable cross section with Reynolds number $Re=900$. The minimum pressure is observed in the minimum cross section, and at some point downstream. fig1

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.