According to Maxwell's Equations, the electromagnetic waves in vacuum travel at the speed of light $c$. While solving Maxwell's equations using Lorenz gauge conditions (or basically evaluating scalar and vector potentials via D'Alembert operator) we find retarded potential.
The retarded potential is basically evaluated at the time when the field began to propagate from the point where it was emitted to an observer, also known as retarded time.
Calculation of Lienard-Wiechert potential shows that the magnitude of electric field at a point due to a moving charge is from the position of the particle at retarded time, however the direction of the field at the same point is in the outward direction from the present position of the positive charge as shown in Fig. 1. A commonly attributed reason is that the signal takes a finite time to propagate from a point in the charge or current distribution (the point of cause) to another point in space (where the effect is measured).
However, if any information cannot travel faster than $c$, how can the direction at the observing point $A$ be outwards from the present position? If the information containing direction can reach the observer instantaneously, why not the magnitude?
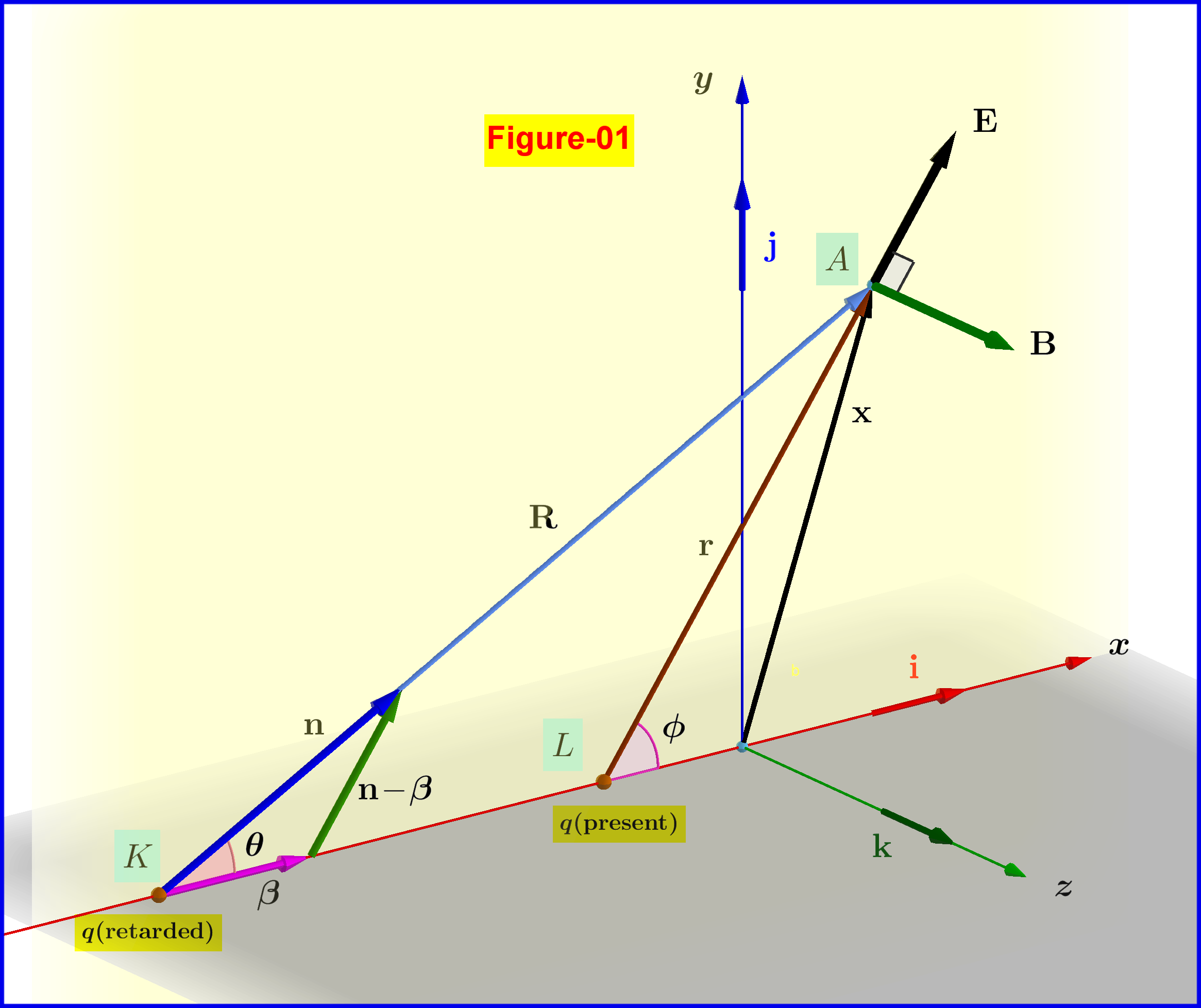 Fig. 1: Magnitude of the electric field at A appears to be as if the charge is at K, however, the direction at A is as if it is at L.
Fig. 1: Magnitude of the electric field at A appears to be as if the charge is at K, however, the direction at A is as if it is at L.
