The classical (retarded) Lienard-Wiechert scalar and vector potentials describe the electromagnetic field due to an arbitrarily moving electric point charge.
Thus given the motion of electron $A$ one can calculate the EM field at the position of electron $B$. Using the Lorentz force law one can then calculate the force exerted on electron $B$ due to electron $A$.
But should there also be a reaction force exerted back on electron $A$ from electron $B$ ?
The standard view asserts that the momentum gained by electron $B$ is balanced by an opposite momentum in the EM field.
However, consider the following particle interaction picture of the electromagnetic interaction between electron $A$ and electron $B$.
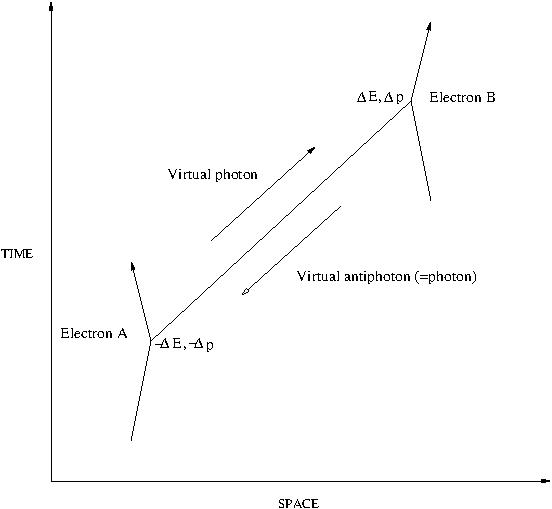
Electron $A$ emits a virtual photon which is absorbed by electron $B$ so that it gains some positive energy-momentum ($\Delta E$,$\Delta p$).
This process is described classically by electron $B$ being acted upon by the retarded Lienard-Wiechert EM field due to electron $A$.
But, according to the Feynman-Stueckelberg interpretation, a virtual photon travelling from $A$ to $B$, delivering energy-momentum ($\Delta E$,$\Delta p$) to electron $B$, is equivalent to a virtual photon travelling from $B$ to $A$, delivering energy-momentum ($-\Delta E$,-$\Delta p$) to electron $A$.
Thus in the particle interaction picture there is also a reaction force ($-\Delta p/\Delta t$) back on electron $A$ due to a virtual photon travelling backwards in time from electron $B$.
The standard classical description due to retarded Lienard-Wiechert potentials does not describe this reaction force back on the source particle.
Thus the standard classical description of the EM interaction between charged particles seems to be incomplete - momentum is not conserved.
It seems that in order to complete the classical description of particle-particle electromagnetic interactions one needs to employ advanced Lienard-Wiechert potentials as well as the retarded potentials.
Does this make sense?
